基于多李雅普诺夫函数的一般非线性系统渐近镇定

杨可馨; 李永强; 侯忠生; 冯宇

doi:10.16383/j.aas.c240309

基于多李雅普诺夫函数的一般非线性系统渐近镇定

doi: 10.16383/j.aas.c240309 cstr: 32138.14.j.aas.c240309

1.
浙江工业大学信息工程学院杭州 310000
2.
青岛大学自动化学院青岛 266071

基金项目: 国家自然科学基金(62073294, 62373206, U2341216)资助

详细信息

作者简介:
杨可馨：浙江工业大学信息工程学院硕士研究生. 主要研究方向为非线性控制. E-mail: 211122030042@zjut.edu.cn

李永强：浙江工业大学信息工程学院副教授. 2014年获得北京交通大学控制理论与控制工程专业博士学位. 主要研究方向为非线性控制, 最优控制, 机器人控制和强化学习. 本文通信作者. E-mail: yqli@zjut.edu.cn

侯忠生：青岛大学自动化学院首席教授. 1994年获得东北大学博士学位. 主要研究方向为无模型自适应控制, 数据驱动控制, 学习控制和智能交通系统. E-mail: zshou@qdu.edu.cn

冯宇：浙江工业大学信息工程学院教授. 2011年获得法国南特矿业学院博士学位. 主要研究方向为网络化控制系统, 不确定系统的鲁棒分析与控制, 博弈论与机器学习在决策问题中的应用. E-mail: yfeng@zjut.edu.cn

计量
- 文章访问数: 68
- HTML全文浏览量: 25
- 被引次数: 0
出版历程
- 收稿日期: 2024-05-31
- 录用日期: 2024-11-06
- 网络出版日期: 2024-11-26

Asymptotically Stabilization for General Nonlinear Systems Based on Multiple Lyapunov Functions

1.
College of Information Engineering, Zhejiang University of Technology, Hangzhou 310000
2.
College of Automation, Qingdao University, Qingdao 266071

Funds: Supported by National Natural Science Foundation of China (62073294, 62373206, U2341216)

More Information

Author Bio:
YANG Ke-Xin　Master student at the College of Information Engineering, Zhejiang University of Technology. Her main research interest is nonlinear control

LI Yong-Qiang　Associate professor at the College of Information Engineering, Zhejiang University of Technology. He received his Ph.D. degree in control theory and control engineering from Beijing Jiaotong University in 2014. His research interest covers nonlinear control, optimal control, robotic control, and reinforcement learning. Corresponding author of this paper

HOU Zhong-Sheng　Chair professor at the College of Automation, Qingdao University. He received his Ph.D. degree from Northeastern University in 1994. His research interest covers model-free adaptive control, data-driven control, learning control, and intelligent traffic systems

FENG Yu　Professor at the College of Information Engineering, Zhejiang University of Technology. He received his Ph.D. degree from École nationale supérieure des mines de Nantes in 2011. His research interest covers networked control systems, robust analysis and control for uncertainty systems, and applications of game theory and machine learning in decision-making

摘要

摘要: 针对离散时间非线性系统, 提出一种基于多李雅普诺夫(Lyapunov)函数的控制器设计方法. 该方法不仅能够保证闭环系统稳定性, 还能够扩大闭环吸引域(Domain of attraction, DOA). 首先, 给出基于多Lyapunov函数下系统渐近稳定的充分条件. 结果表明, 由多个Lyapunov函数的负定不变集构成的并集是一个稳定的控制集合, 其从控制空间到状态空间的投影是闭环DOA的估计. 随后, 使用区间分析算法求解集合的内近似估计, 基于此算法可以求解多Lyapunov函数的负定不变集的近似值和闭环DOA的估计值, 并给出相应控制器的设计方法. 最后, 通过仿真算例验证本文方法的有效性.
- 非线性系统 /
- 多李雅普诺夫函数 /
- 稳定性 /
- 吸引域 /
- 区间分析
Abstract: In this paper, a controller design method based on multiple Lyapunov functions is proposed for discrete-time nonlinear systems. The proposed method can not only ensure the stabilization of the closed-loop system, but also enlarge the closed-loop domain of attraction (DOA). Firstly, the sufficient conditions for the asymptotically stabilization of the system based on multiple Lyapunov functions are given. It is shown that the union of negative-definite-invariant sets of multiple Lyapunov functions is a stable control set, and its projection from the control space to the state space is an estimate of the closed-loop DOA. Then, the interval analysis algorithm is used to solve the inner approximate extimation of the set. Based on this algorithm, the negative-definite-invariant sets of multiple Lyapunov functions can be approximated and the closed-loop DOA can be estimated, the design method of the corresponding controller is also given. Finally, the simulation example is given to verify the effectiveness of the proposed method.
- Nonlinear systems /
- multiple Lyapunov functions /
- stabilization /
- domain of attraction (DOA) /
- interval analysis

HTML全文

图 1 引理1渐近稳定的说明

Fig. 1 The illumination of asymptotically stabilization of lemma 1

下载: 全尺寸图片幻灯片

图 2 负定集取并集的说明

Fig. 2 Description of the union set of negative-definite sets

下载: 全尺寸图片幻灯片

图 3 单个Lyapunov函数生成的近似负定集和负定不变集

Fig. 3 The approximate negative-define set and negative-define-invariant set for a given Lyapunov function

下载: 全尺寸图片幻灯片

图 4 粒子群算法寻找最优Lyapunov函数

Fig. 4 Particle swarm optimization algorithm for finding the optimal Lyapunov function

下载: 全尺寸图片幻灯片

图 5 不同数量Lyapunov函数负定不变集和闭环DOA对比

Fig. 5 Comparison of negative-definite-invariant sets and closed-loop DOA for different numbers of Lyapunov functions

下载: 全尺寸图片幻灯片

图 6 多Lyapunov函数的近似负定集和负定不变集以及收敛轨迹

Fig. 6 The approximate negative-definite sets and negative-definite-invariant sets of multiple Lyapunov functions and convergence trajectories

下载: 全尺寸图片幻灯片

参考文献(26)

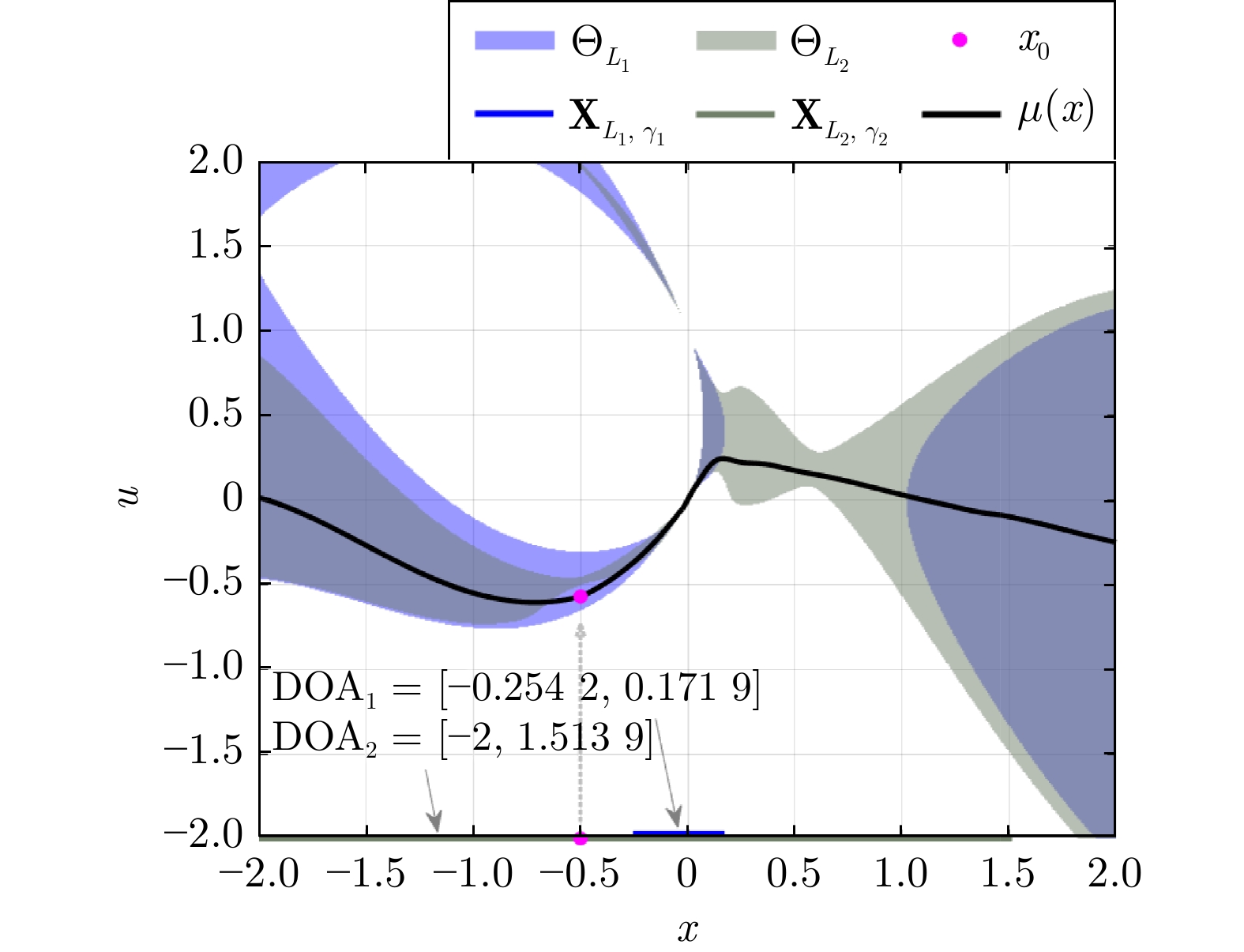
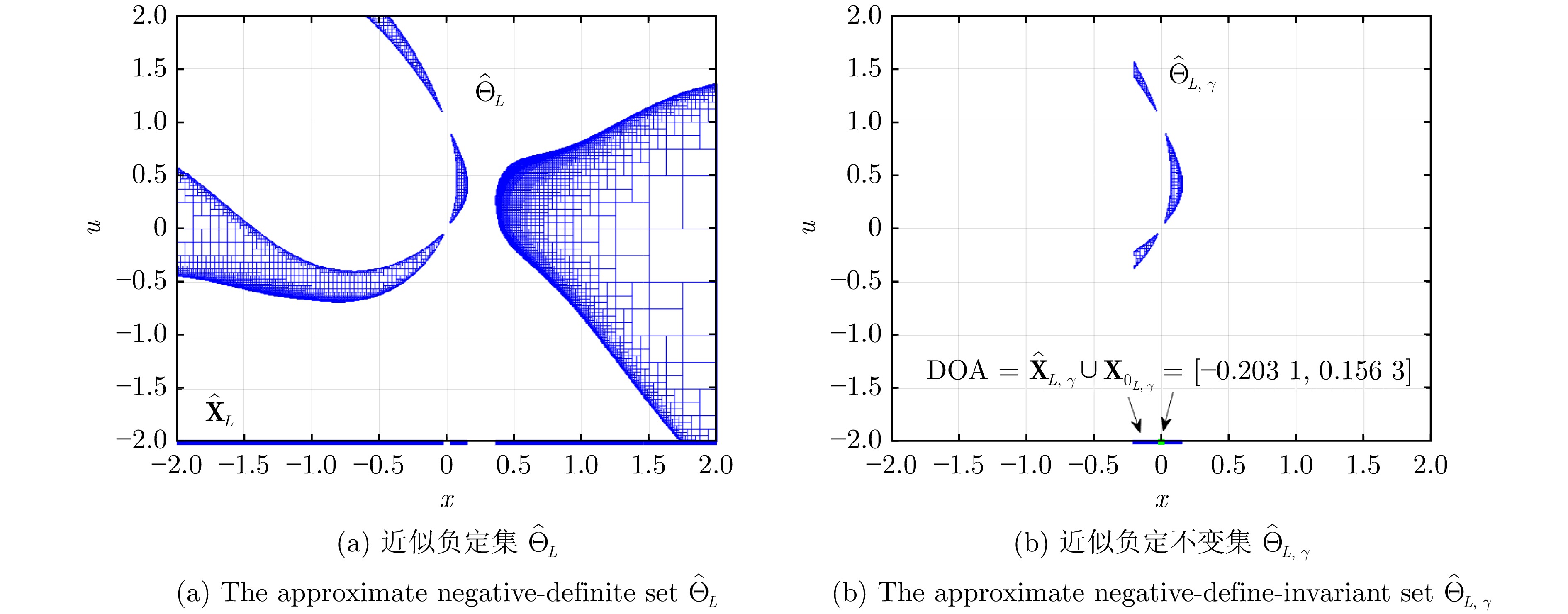
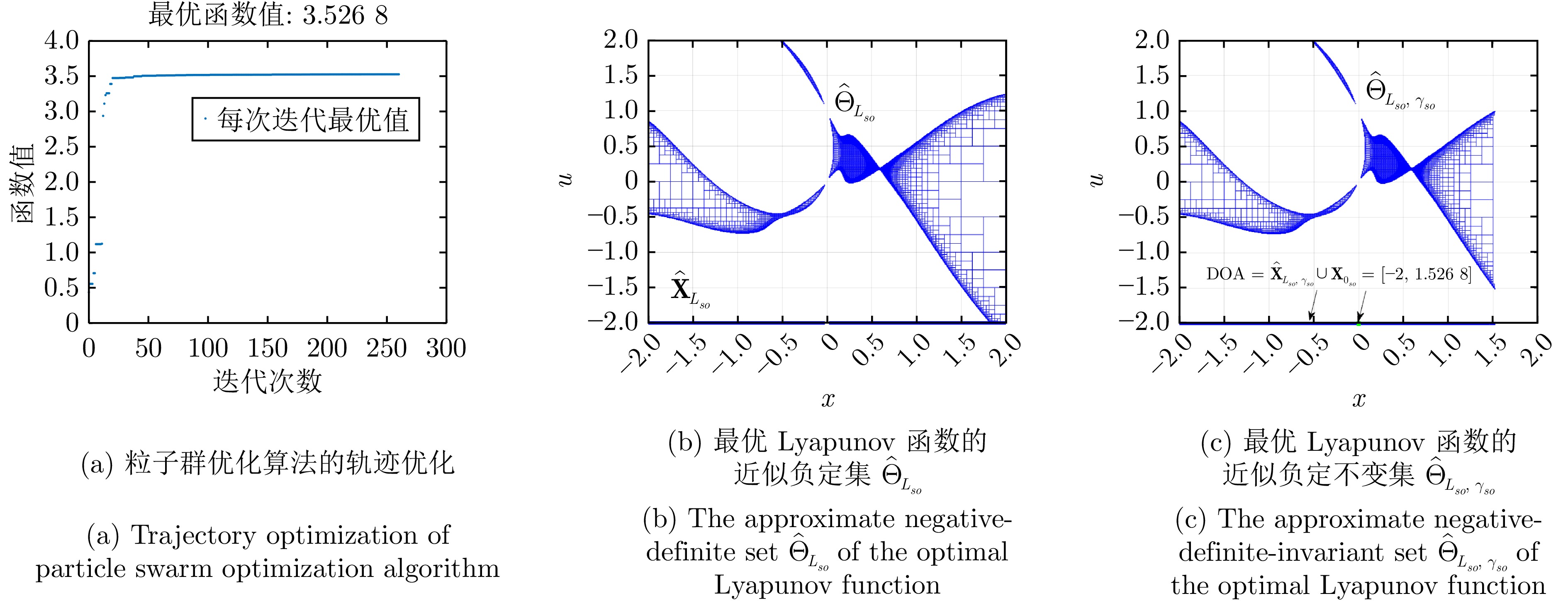
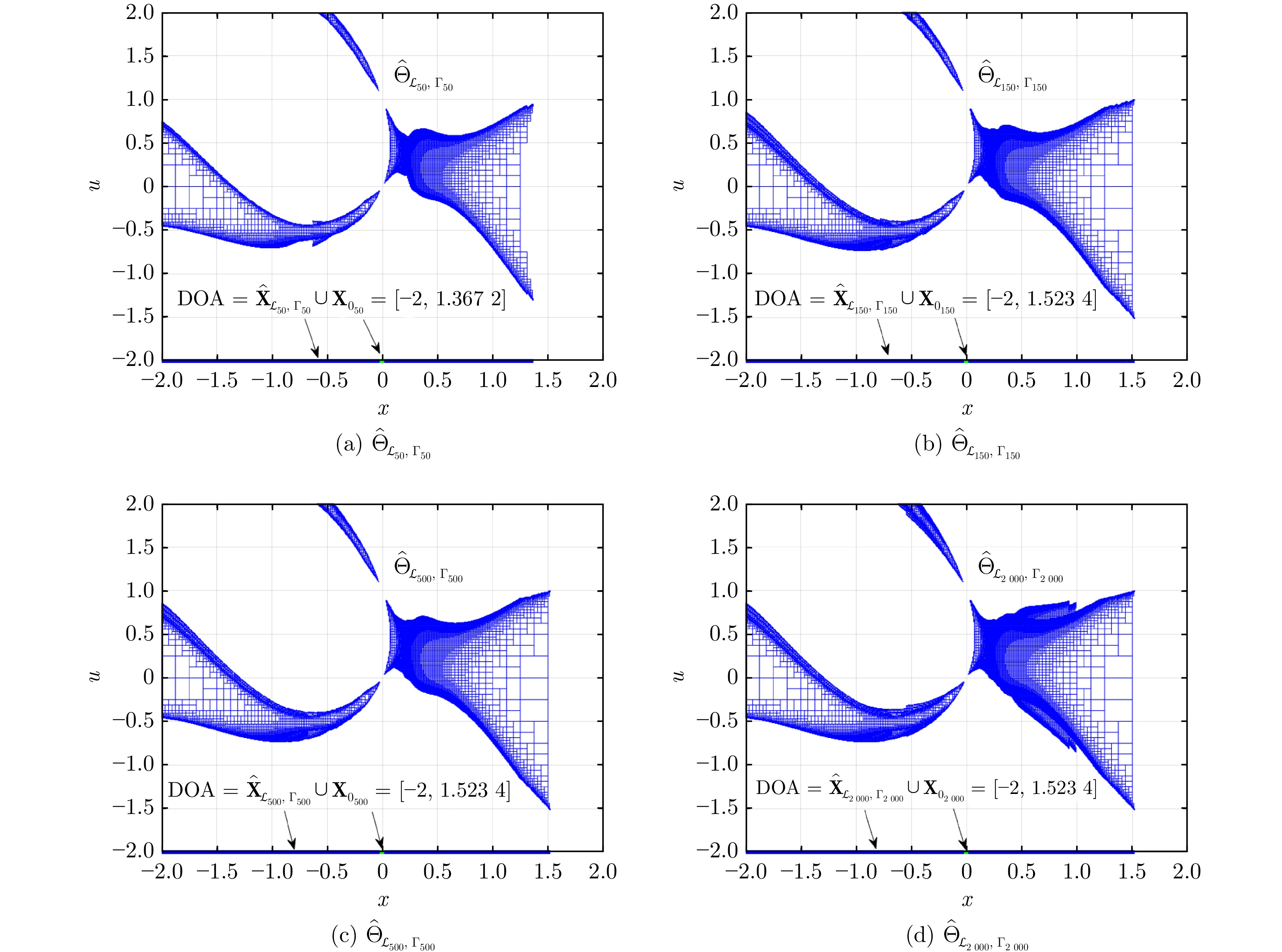
[1]	Khalil H K. Nonlinear Systems Third Edition. Upper Saddle River: Prentice Hall, 2002. 126−133
[2]	Monfared M N, Yazdanpanah M J. Optimal dynamic Lyapunov function and the largest estimation of domain of attraction. IFAC-PapersOnLine, 2017, 50: 2645−2650 doi: 10.1016/j.ifacol.2017.08.469
[3]	Tibken B. Estimation of the domain of attraction for polynomial systems via LMIs. In: Proceedings of the 39th IEEE Conference on Decision and Control (Cat.No.00CH37187). Sydney, Australia: IEEE, 2000. 3860−3864
[4]	Ali M M A, Jamali A, Asgharnia A, Ansari R, Mallipeddi R. Multi-objective Lyapunov-based controller design for nonlinear systems via genetic programming. Neural Computing and Applications, 2022, 34: 1345−1357 doi: 10.1007/s00521-021-06453-1
[5]	Dabbaghi B, Hamidi F, Jerbi H, Aoun M. Estimating and enlarging the domain of attraction for a nonlinear system with input saturation. In: Proceedings of the IEEE International Workshop on Mechatronic Systems Supervision (IW_MSS). Hammamet, Tunisia: IEEE, 2023. 1−5
[6]	Binu K U, Mija S J, Cheriyan E P. Nonlinear analysis and estimation of the domain of attraction for a droop controlled microgrid system. Electric Power Systems Research, 2022, 204: Article No. 107712 doi: 10.1016/j.jpgr.2021.107712
[7]	Saleme A, Tibken B, Warthenpfuhl S, Selbach C. Estimation of the domain of attraction for non-polynomial systems: A novel method. IFAC Proceedings, 2011, 44: 10976−10981 doi: 10.3182/20110828-6-IT-1002.01450
[8]	Wang S J, Lu J J, She Z K. Estimating the minimal domains of attraction of uncertain discrete-time switched systems under state-dependent switching. Nonlinear Analysis: Hybrid Systems, 2024, 54: Article No. 101527 doi: 10.1016/j.nahs.2024.101527
[9]	Majumdar A, Vasudevan R, Tobenkin M M, Tedrake R. Convex optimization of nonlinear feedback controllers via occupation measures. The International Journal of Robotics Research, 2014, 33(9): 1209−1230 doi: 10.1177/0278364914528059
[10]	Najafi E, Lopes G A D, Babuska R. Balancing a legged robot using state-dependent Riccati equation control. IFAC Proceedings, 2014, 47: 2177−2182 doi: 10.3182/20140824-6-ZA-1003.01724
[11]	Bedoui S, Bacha A B B, Elloumi S, Braiek N B. Enlarging nonlinear discrete system's domain of attraction via linear control law. In: Proceedings of the 5th International Conference on Advanced Systems and Emergent Technologies (IC_ASET). Hammamet, Tunisia: IEEE, 2022. 489−493
[12]	Yadipour M, Hashemzadeh F, Baradarannia M. Controller design to enlarge the domain of attraction for a class of nonlinear systems. In: Proceedings of the International Conference on Research and Education in Mechatronics (REM). Wolfenbuettel, Germany: IEEE, 2017. 1−5
[13]	Meng F W, Wang D N, Yang P H, Xie G Z, Guo F. Application of sum-of-squares method in estimation of region of attraction for nonlinear polynomial systems. IEEE Access, 2020, 8: 14234−14243 doi: 10.1109/ACCESS.2020.2966566
[14]	Davó M A, Prieur C, Fiacchini M, Nešić D. Enlarging the basin of attraction by a uniting output feedback controller. Automatica, 2018, 90: 73−80 doi: 10.1016/j.automatica.2017.12.044
[15]	Li Y Q, Hou Z S. Data-driven asymptotic stabilization for discrete-time nonlinear systems. Systems & Control Letters, 2014, 64: 79−85
[16]	Rossa M D, Jungers R M. Multiple Lyapunov functions and memory: A symbolic dynamics approach to systems and control. arXiv: 2307.13543, 2024.
[17]	Chen S Z, Ning C Y. Improved multiple Lyapunov functions of input-to-output stability and input-to-state stability for switched systems. Journal of Advanced Transportation, 2022, 606: 47−62
[18]	Yang D, Zong G D, Liu Y J, Ahn C K. Adaptive neural network output tracking control of uncertain switched nonlinear systems: An improved multiple Lyapunov function method. Information Sciences, 2022, 606: 380−396 doi: 10.1016/j.ins.2022.05.071
[19]	Jaulin L, Kieffer M, Didrit O, Walter E. Applied Interval Analysis: With Examples in Parameter and State Estimation, Robust Control and Robotics. Berlin: Springer-Verlag, 2001.
[20]	陈辉, 邓东明, 韩崇昭. 基于区间箱粒子多伯努利滤波器的传感器控制策略. 自动化学报, 2021, 47(6): 1428−1443 Chen Hui, Deng Dong-Ming, Han Chong-Zhao. Sensor control based on interval box-particle multi-Bernoulli filter. Acta Automatica Sinica, 2021, 47(6): 1428−1443
[21]	Li Y Q, Lu C L, Li Y N, Hou Z S, Feng Y, Feng Y J. Stabilization with closed-loop DOA enlargement: An interval analysis approach. arXiv: 1912.11775, 2021.
[22]	Haddad W M, Chellaboina V. Nonlinear Dynamical Systems and Control: A Lyapunov-based Approach. Princeton: Princeton University Press, 2008.
[23]	Kieffer M, Braems I, Walter E Jaulin L. Guaranteed Set Computation With Subpavings. Boston: Springer, 2001. 167−178
[24]	Jaulin L, Walter E. Set inversion via interval analysis for nonlinear bounded-error estimation. Automatica, 1993, 29: 1053−1064 doi: 10.1016/0005-1098(93)90106-4
[25]	Topcu U, Packard A, Seiler P, Balas G. Help on SOS [Ask the experts]. IEEE Control Systems Magazine, 2010, 30(4): 18−23 doi: 10.1109/MCS.2010.937045
[26]	Powers V, Wörmann T. An algorithm for sums of squares of real polynomials. Journal of Pure and Applied Algebra, 1998, 127: 99−104 doi: 10.1016/S0022-4049(97)83827-3