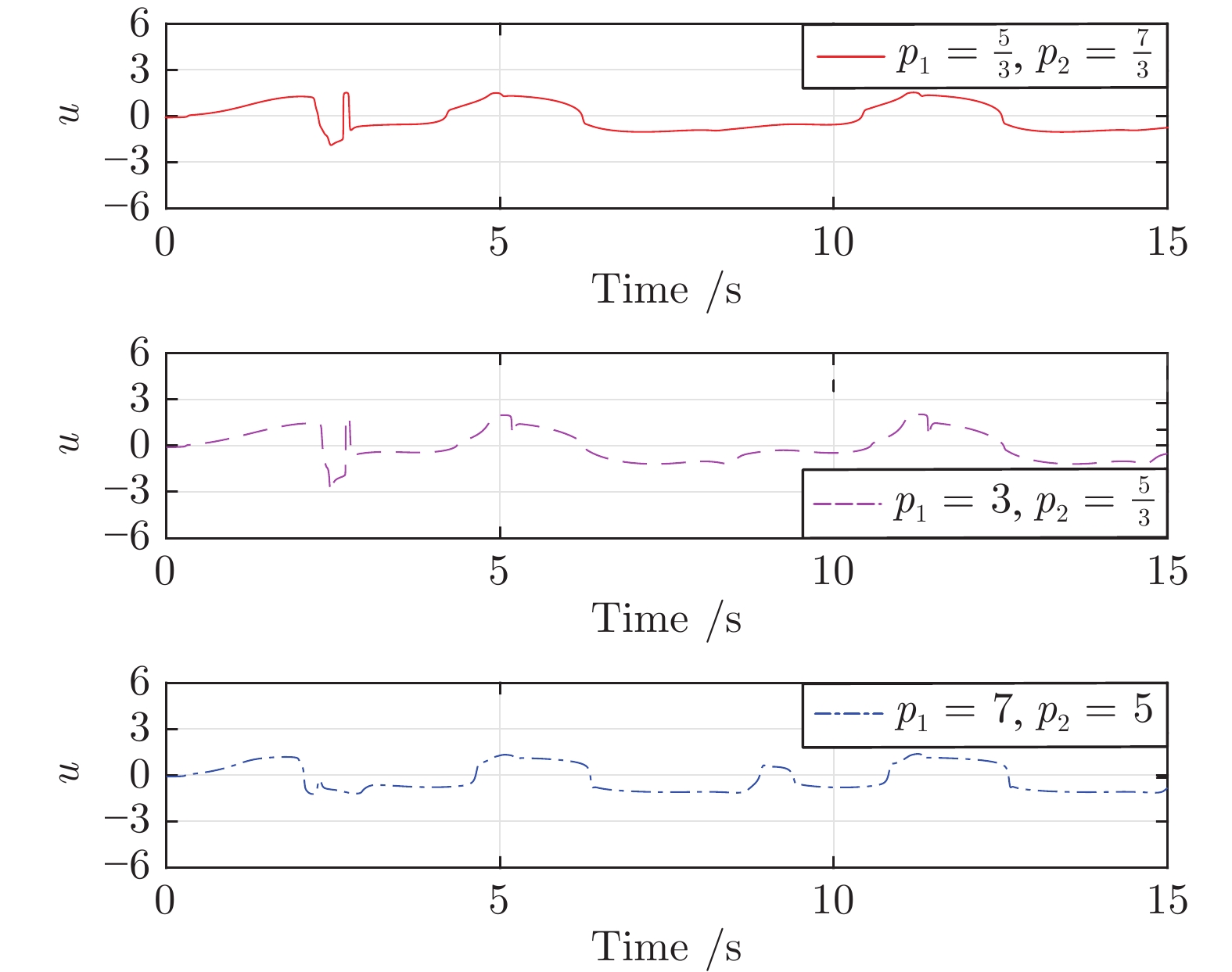
|
[1]
|
Rui C L, Reyhangolu M, Kolmanovsky I, Cho S, McClamroch N H. Nonsmooth stabilization of an underactuated unstable two degrees of freedom mechanical system. In: Proceedings of the 36th IEEE Conference on Decision and Control. California, USA: IEEE, 1997. 3998−4003
|
|
[2]
|
Su Z G, Qian C J, Wang Q, Wang Z. Reduced-order observer and controller design for a 1 000 mw ultra-supercritical unit. In: Proceedings of the 58th ISA Power Generation Division Symposium. Florida, USA: ISA, 2015. 129−140
|
|
[3]
|
Lin W, Qian C. Adding one power integrator: a tool for global stabilization of high-order lower-triangular systems. Systems and Control Letters, 2000, 39(5): 339-351 doi: 10.1016/S0167-6911(99)00115-2
|
|
[4]
|
Lin W, Qian C. Adaptive regulation of high-order lowertriangular systems: an adding a power integrator technique. Systems and Control Letters, 2000, 39(5): 353-364 doi: 10.1016/S0167-6911(99)00114-0
|
|
[5]
|
Qian C, Lin W. A continuous feedback approach to global strong stabilization of nonlinear systems. IEEE Transactions on Automatic Control, 2001, 46(7): 1061-1079 doi: 10.1109/9.935058
|
|
[6]
|
Lin W, Qian C J. Adaptive control of nonlinearly parameterized systems: A nonsmooth feedback framework. IEEE Transactions on Automatic Control, 2002, 47(5): 757-774 doi: 10.1109/TAC.2002.1000270
|
|
[7]
|
Qian C J, Lin W. Practical output tracking of nonlinear systems with uncontrollable unstable linearization. IEEE Transactions on Automatic Control, 2002, 47(1): 21-36 doi: 10.1109/9.981720
|
|
[8]
|
Lin W, Pongvuthithum R. Adaptive output tracking of inherently nonlinear systems with nonlinear parameterization. IEEE Transactions on Automatic Control, 2003, 48(10): 1737-1749 doi: 10.1109/TAC.2003.817922
|
|
[9]
|
Yang B, Lin W. Homogeneous observers, iterative design, and global stabilization of high-order nonlinear systems by smooth output feedback. IEEE Transactions on Automatic Control, 2004, 49(7): 1069-1080 doi: 10.1109/TAC.2004.831186
|
|
[10]
|
Back J, Cheong S G, Shim H, Seo J H. Nonsmooth feedback stabilizer for strict-feedback nonlinear systems that may not be linearizable at the origin. Systems and Control Letters, 2007, 56(11-12): 742-752 doi: 10.1016/j.sysconle.2007.04.009
|
|
[11]
|
Yan X H, Liu Y G. Global practical tracking for high-order uncertain nonlinear systems with unknown control directions. SIAM Journal on Control and Optimization, 2010, 48(7): 4453-4473 doi: 10.1137/090769727
|
|
[12]
|
Fu J, Ma R, Chai T. Global finite-time stabilization of a class of switched nonlinear systems with the powers of positive odd rational numbers. Automatica, 2015, 54: 360-373 doi: 10.1016/j.automatica.2015.02.023
|
|
[13]
|
Sun Z Y, Xue L R, Zhang K. A new approach to finitetime adaptive stabilization of high-order uncertain nonlinear system. Automatica, 2015, 58: 60-66 doi: 10.1016/j.automatica.2015.05.005
|
|
[14]
|
Fu J, Ma R, Chai T. Adaptive finite-time stabilization of a class of uncertain nonlinear systems via logic-based switchings. IEEE Transactions on Automatic Control, 2017, 62(11): 5998-6003 doi: 10.1109/TAC.2017.2705287
|
|
[15]
|
Sun Z Y, Shao Y, Chen C C. Fast finite-time stability and its application in adaptive control of high-order nonlinear system. Automatica, 2019, 106: 339-348 doi: 10.1016/j.automatica.2019.05.018
|
|
[16]
|
段纳, 解学军. 具 有iISS未建模动态的非线性系统的状态反馈调节. 自动化学报, 2010, 36(7): 1033-1036 doi: 10.3724/SP.J.1004.2010.01033Duan Na, Xie Xue Jun. State-feedback Regulation of Nonlinear Systems with iISS Unmodeled Dynamics. Acta Automatica Sinca, 2010, 36(7): 1033-1036 doi: 10.3724/SP.J.1004.2010.01033
|
|
[17]
|
张健, 刘允刚. 一类不确定非线性 系统无过参数自适应控制设计新方法. 中国科学: 信息科学, 2011, 41(7): 892-902Jian Zhang, Liu Yun-Gang. A new approach to adaptive control design without overparametrization for a class of uncertain nonlinear systems. Science China Information Sciences, 2011, 41(7): 892-902
|
|
[18]
|
满永超, 刘允刚. 高阶不确定非线性系统线性状态反馈自适应控制 设计. 自动化学报, 2014, 40(1): 24-32Man Yong-Chao, Liu Yun-Gang. Adaptive Control Design via Linear State-feedback for High-order Uncertain Nonlinear Systems. Acta Automatica Sinca, 2014, 40(1): 24-32
|
|
[19]
|
孙丞, 孙鹤旭, 刁心薇. 一类非齐次高阶非线性 系统的连续反馈控制设计. 自动化学报, 2014, 40(1): 151-155Sun Cheng, Sun He-Xu, Diao Xin-Wei. Continuous Feedback Control Design for a Class of Non-homogeneous Highorder Nonlinear Systems. Acta Automatica Sinca, 2014, 40(1): 151-155
|
|
[20]
|
Su Zhigang, Qian Chunjiang, Shen Jiong. Interval homogeneity-based control for a class of nonlinear systems with unknown power drifts. IEEE Transactions on Automatic Control, 2017, 62(3): 1445-1450 doi: 10.1109/TAC.2016.2575819
|
|
[21]
|
Chen C C, Qian C, Lin X, Sun Z Y, Liang Y W. Smooth output feedback stabilization for a class of nonlinear systems with time-varying powers. International Journal of Robust and Nonlinear Control, 2017, 27(18): 5113-5128 doi: 10.1002/rnc.3826
|
|
[22]
|
Man Yong-Chao, Liu Yun-Gang. Global adaptive stabilization and practical tracking for nonlinear systems with unknown powers. Automatica, 2019, 100: 171-181 doi: 10.1016/j.automatica.2018.11.011
|
|
[23]
|
Guo C, Xie R, Xie X J. Adaptive control of full-state constrained high-order nonlinear systems with time-varying powers. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2019, doi: 10.1109/TSMC.2019.2946350
|
|
[24]
|
Wang M, Liu Y, Man Y. Switching adaptive controller for the nonlinear systems with uncertainties from unknown powers. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2020, 50(7): 2375-2385 doi: 10.1109/TSMC.2018.2814345
|
|
[25]
|
Man Y, Liu Y. Global adaptive stabilization for planar nonlinear systems with unknown input powers. Science China Information Sciences, 2021, 64: 199204:1-199204:3 doi: 10.1007/s11432-018-9774-y
|
|
[26]
|
Tee K P, Ge S S, Tay E H. Barrier Lyapunov functions for the control of output-constrained nonlinear systems. Automatica, 2009, 45(4): 918-927 doi: 10.1016/j.automatica.2008.11.017
|
|
[27]
|
Jankovic M. Robust control barrier functions for constrained stabilization of nonlinear systems. Automatica, 2018, 96: 359-367 doi: 10.1016/j.automatica.2018.07.004
|
|
[28]
|
Khalil H K. Nonlinear Systems, 3rd Edition. Englewood Cliffs: Prentice-Hall, 2002.
|
|
[29]
|
Qian C, Lin W. Non-Lipschitz continuous stabilizers for nonlinear systems with uncontrollable unstable linearization. Systems and Control Letters, 2015, 42(3): 185-200
|
|
[30]
|
Hardy G H, Littlewood J E, Pólya G. Inequalities. London: Cambridge University Press. 1934.
|
|
[31]
|
Wang C, Zuo Z. Adaptive trajectory tracking control of output constrained multi-rotors systems. IET Control Theory and Applications, 2014, 8(13): 1163-1174 doi: 10.1049/iet-cta.2013.0949
|
|
[32]
|
Ren B, Ge S S, Tee K P, Lee T H. Adaptive neural control for output feedback nonlinear systems using a barrier Lyapunov function. IEEE Transactions on Neural Networks, 2010, 21(8): 1339-1345 doi: 10.1109/TNN.2010.2047115
|
|
[33]
|
Logemann M, Ryan E P. Ordinary Differential Equations: Analysis, Qualitative Theory and Control. London, UK: Springer. 2014.
|
|
[34]
|
Liu Y H, Su C Y, Li H, Adaptive output feedback funnel control of uncertain nonlinear systems with arbitrary relative degree. IEEE Transactions on Automatic Control, 2020, doi: 10.1109/TAC.2020.3012027.
|
|
[35]
|
Liu Y H, Liu Y, Liu Y F, Su C Y, Zhou Q, Lu R, Adaptive approximation-based tracking control for a class of unknown high-order nonlinear systems with unknown powers. IEEE Transactions on Cybernetics, 2020, doi: 10.1109/TCYB.2020.3030310.
|




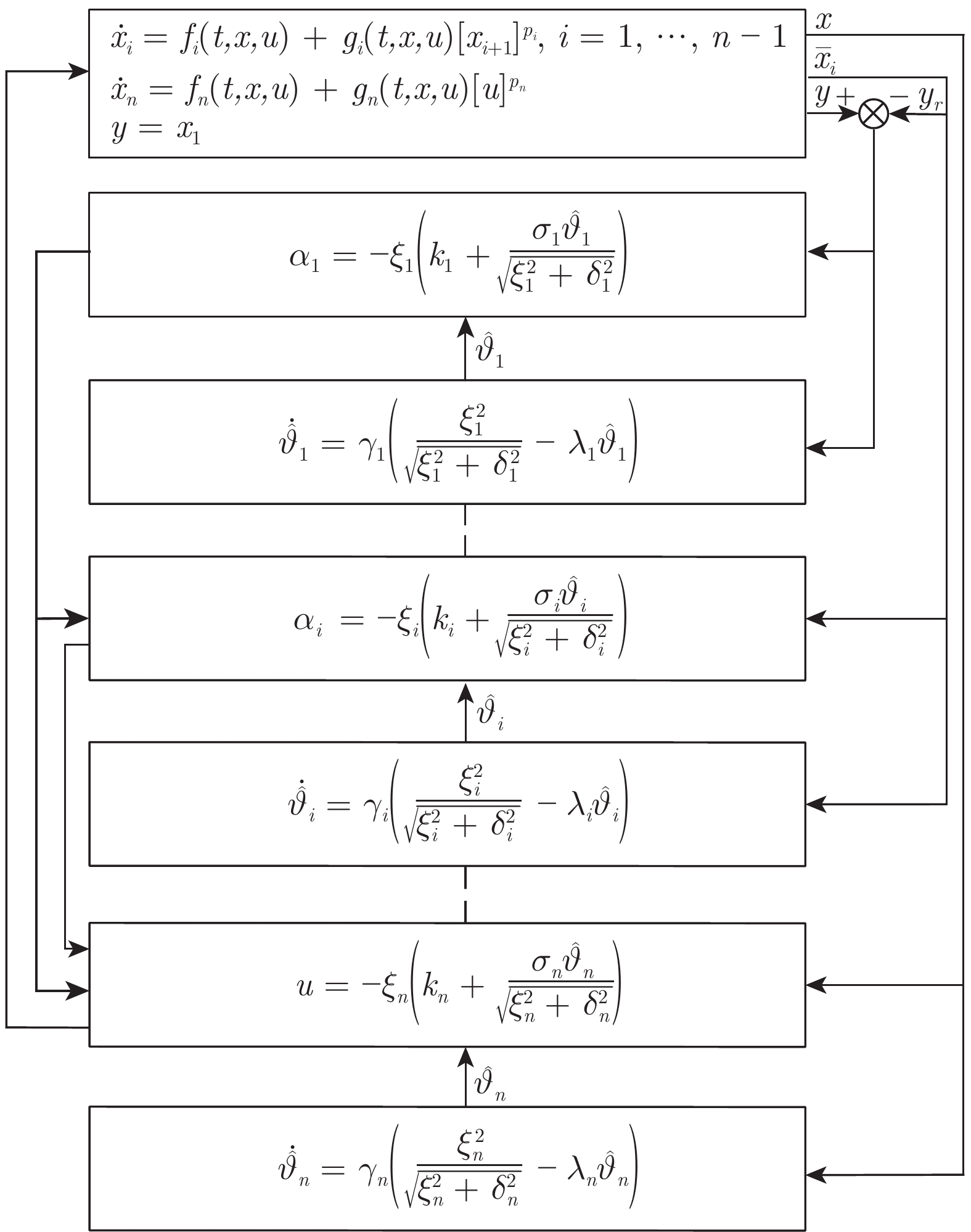
 下载:
下载: