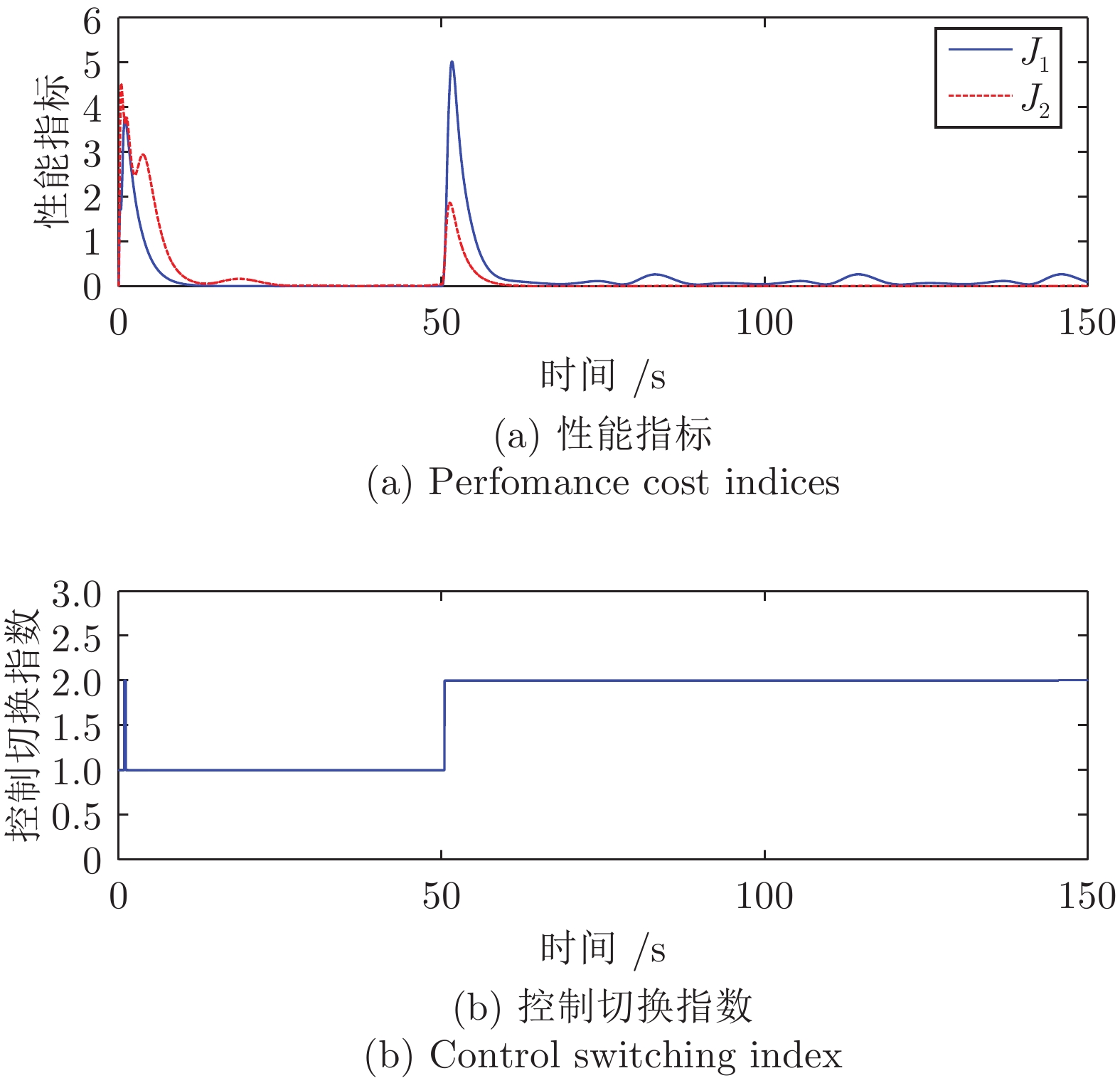
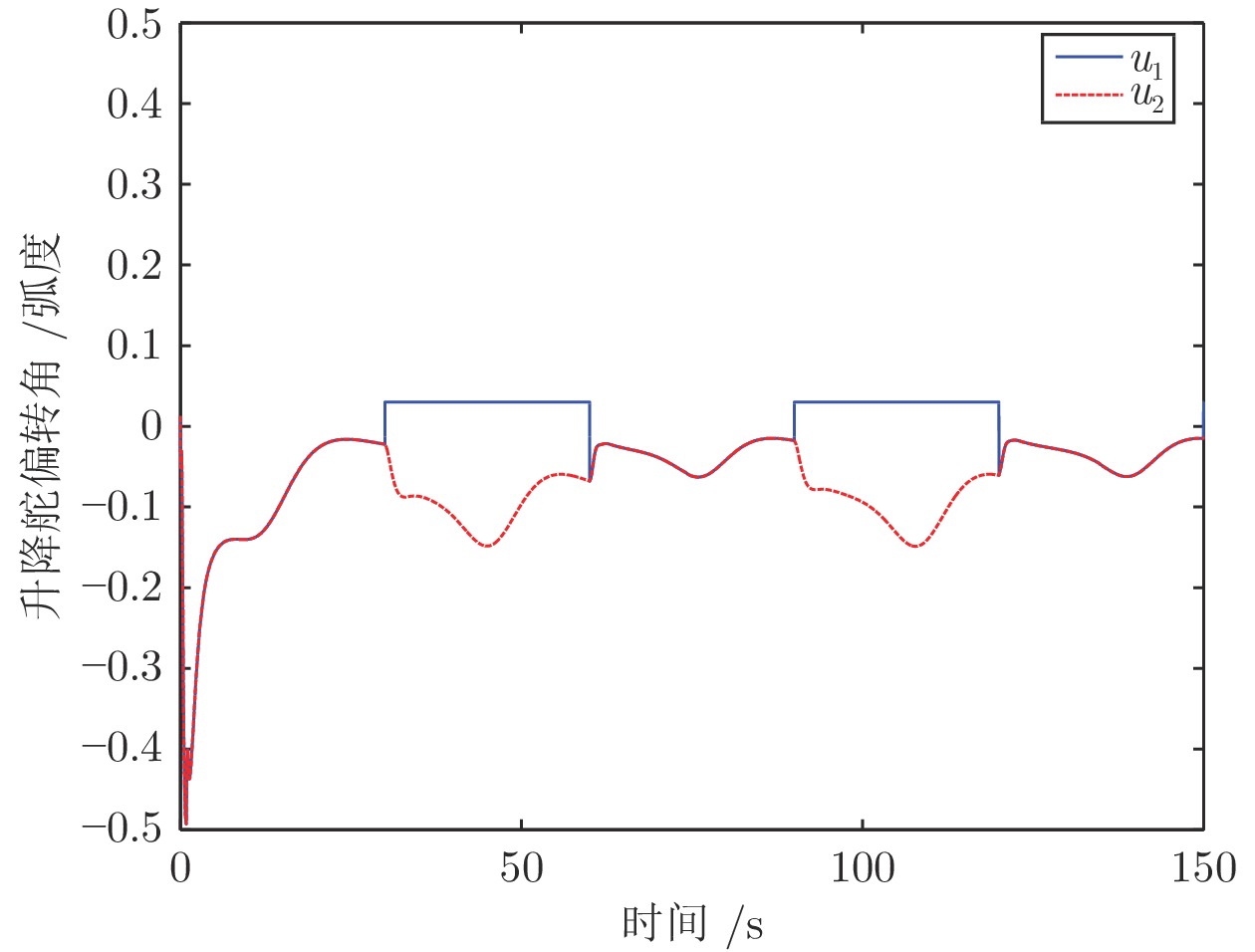
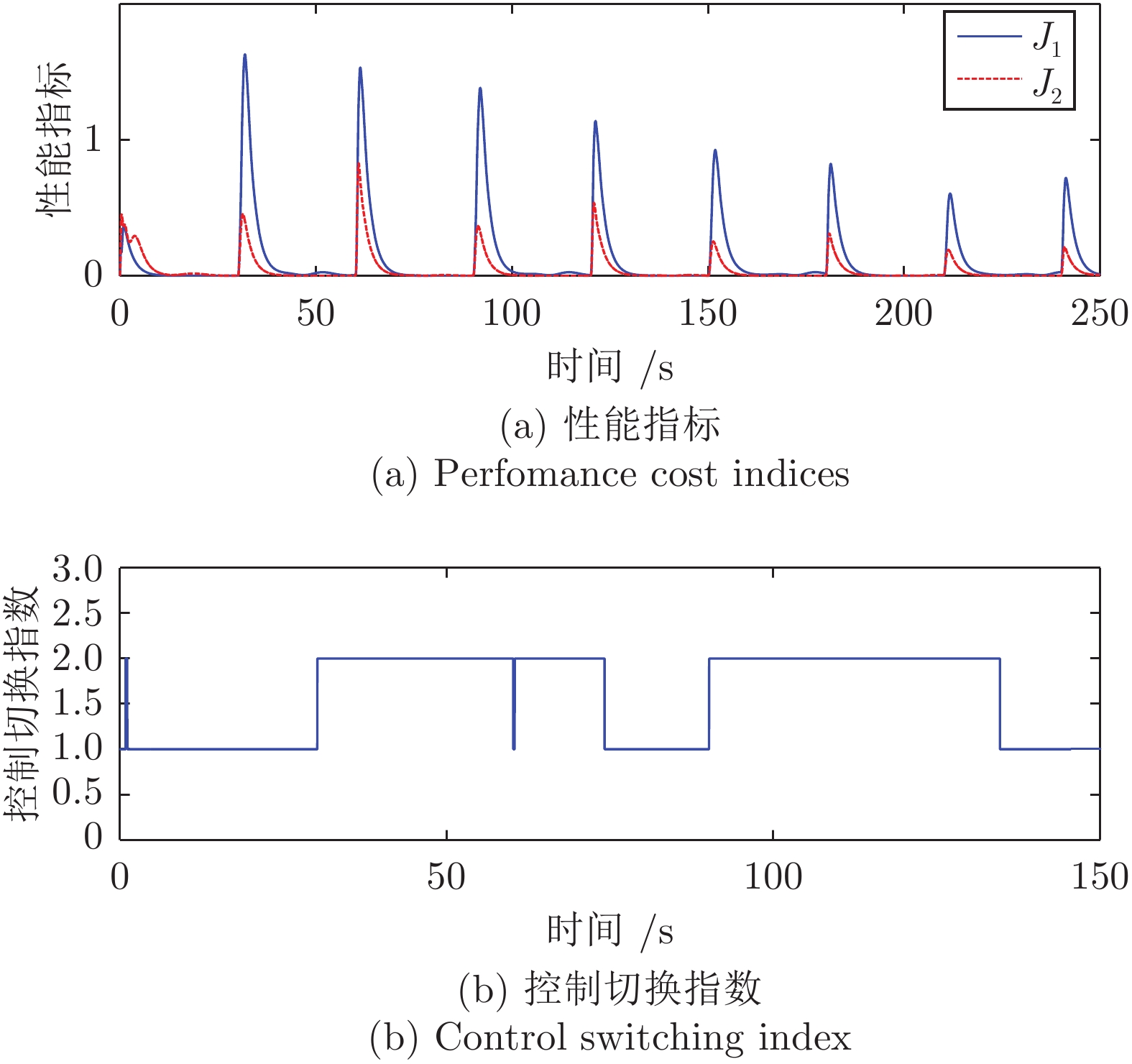
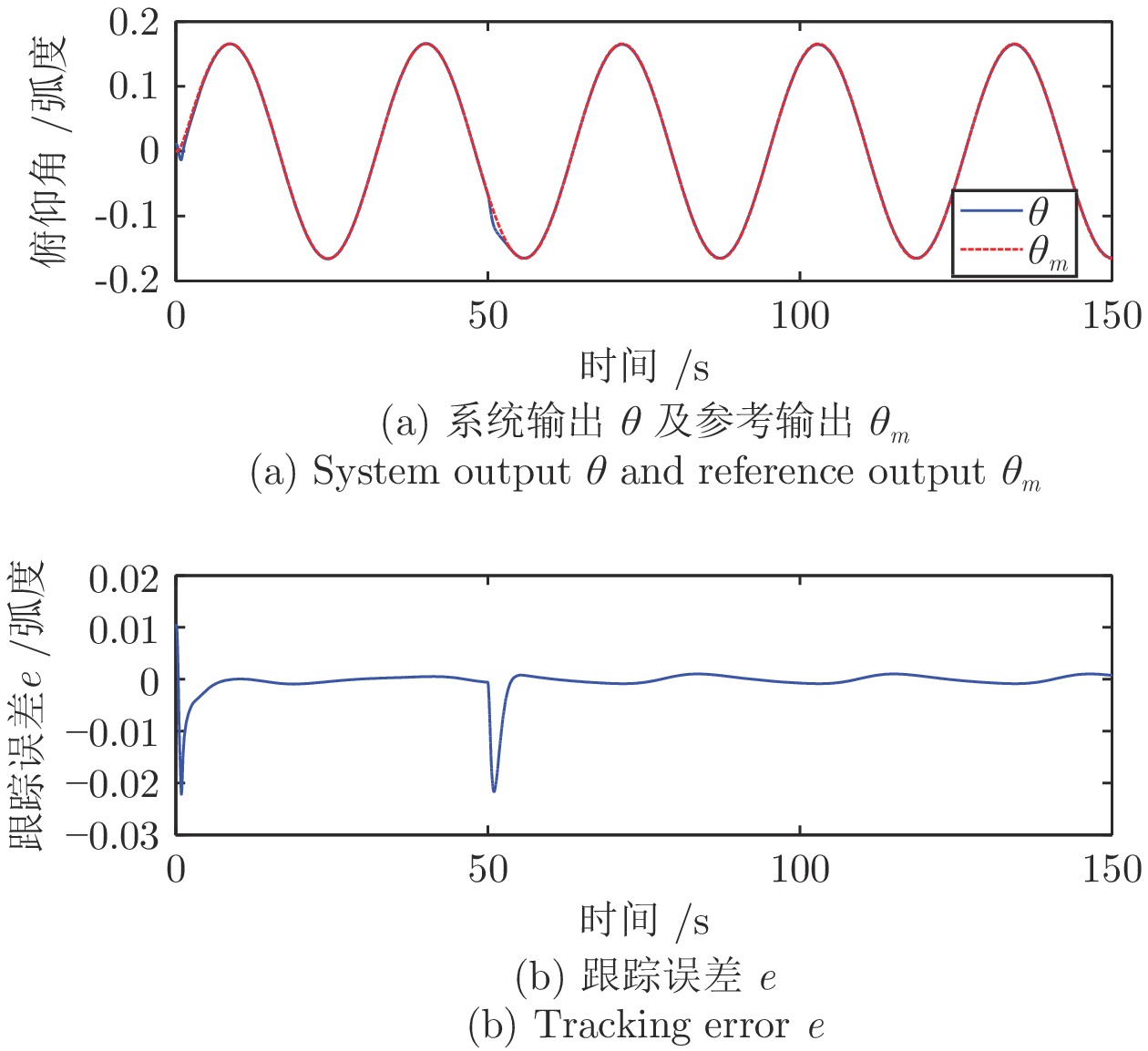
|
[1]
|
周东华, 叶银忠. 现代故障诊断与容错控制. 北京: 清华大学出版社, 2000.Zhou Dong-Hua, Ye Yin-Zhong. Modern fault diagnosis and fault-tolerant control. Beijing: Tsinghua University Press, 2000.
|
|
[2]
|
Tao G, Chen S H, Tang X D, Joshi S M. Adaptive Control of Systems with Actuator Failures, Springer, 2004.
|
|
[3]
|
Tao G. Direct adaptive actuator failure compensation control: a tutorial. Journal of Control and Decision, March 2014, 1(1): 75-101 doi: 10.1080/23307706.2014.885292
|
|
[4]
|
李俊领, 杨光红. 自适应容错控制的发展与展望. 控制与决策, 2014, 29(11): 1921-1926Li Jun-Ling, Yang Guang-Hong. Development and prospect of adaptive fault-tolerant control. Control and Decision, November 2014, 29(11): 1921-1926 (in Chinese)
|
|
[5]
|
杨浩, 姜斌, 周东华. 互联系统容错控制的研究回顾与展望. 自动化学报, 2017, 43(1): 9-19Yang Hao, Jiang Bin, Zhou Dong-Hua. Review and perspectives on fault tolerant control for interconnected systems. Acta Automatica Sinica, 2017, 43(1): 9-19 (in Chinese)
|
|
[6]
|
冯莉, 柴毅, 许水清, 张可, 杨志敏. 基于迭代学习的线性不确定重复系统间歇性故障估计. 自动化学报, 2020, 46(2): 307-319Feng Li, Chai Yi, Xu Shui-Qing, Zhang Ke, Yang Zhi-Min. Iterative learning based intermittent fault estimation for a class of linear uncertain repeated systems. Acta Automatica Sinica, 2020, 46(2): 307-319 (in Chinese)
|
|
[7]
|
Tong S C, Huo B Y, Li Y M. Observer-based adaptive decentralized fuzzy fault-tolerant control of nonlinear large-scale systems with actuator failures. IEEE Transactions on Fuzzy Systems, 2014, 22(1): 1-15 doi: 10.1109/TFUZZ.2013.2241770
|
|
[8]
|
Zhong M Y, Song Y, Ding S X. Parity space-based fault detection for linear discrete time-varying systems with unknown input. Automatica, 2015, 59(9): 120-126
|
|
[9]
|
Zhang Y M, Li X R. Detection and diagnosis of sensor and actuator failures using IMM estimator. IEEE Transactions on Aerospace and Electronic Systems, Oct. 1998, 34(4): 1293-1313 doi: 10.1109/7.722715
|
|
[10]
|
Xu D, Jiang B, Shi P. Robust NSV fault-tolerant control system design against actuator faults and control surface damage under actuator dynamics. IEEE Transactions on Industrial Electronics, 2015, 62(9): 5919-5928 doi: 10.1109/TIE.2015.2450714
|
|
[11]
|
Chen G, Song Y D. Robust fault-tolerant cooperative control of multi-agent systems: A constructive design method. Journal of the Franklin Institute, 2015, 352(10): 4045-4066 doi: 10.1016/j.jfranklin.2015.05.031
|
|
[12]
|
肖冰, 胡庆雷, 张友民. 执行器卡死并失效的航天器姿态稳定控制. 南京航空航天大学学报, 2011, 43: 5-9Xiao Bing, Hu Qing-Lei, Zhang You-Ming. Sliding mode fault tolerant attitude control for flexible spacecraft under actuator fault. Journal of Nanjing University of Aeronautics & Astronautics, 2011, 43: 5-9 (in Chinese)
|
|
[13]
|
李晓航, 朱芳来. 延迟不确定马尔科夫跳变系统的执行器和传感器故障同时估计方法. 自动化学报, 2017, 43(1): 72-82Li Xiao-Hang, Zhu Fang-Lai. Simultaneous estimation of actuator and sensor faults for uncertain time-delayed markovian jump systems. Acta Automatica Sinica, 2017, 43(1): 72-82 (in Chinese)
|
|
[14]
|
鄢镕易, 何潇, 周东华. 一类存在参数摄动的线性随机系统的鲁棒间歇故障诊断方法. 自动化学报. 2016, 42(7): 1004-1013Yan Rong-Yi, He Xiao, Zhou Dong-Hua. Robust Diagnosis of Intermittent Faults for Linear Stochastic Systems Subject to Time-varying Perturbations. Acta Automatica Sinica, 2016, 42(7): 1004-1013 (in Chinese)
|
|
[15]
|
Wang W, Wen C Y. Adaptive compensation for infinite number of actuator failures or faults. Automatica, 2011, 47(10): 2197-2210 doi: 10.1016/j.automatica.2011.08.022
|
|
[16]
|
Wang C L, Guo L. Adaptive compensation for infinite number of actuator failures with an application to flight control. International Journal of Adaptive Control and Signal Processing, 2016, 30(3): 443-455 doi: 10.1002/acs.2585
|
|
[17]
|
Zhang Y W, Qin S J. Adaptive actuator/component fault compensation for nonlinear systems. AIChE Journal, 2008, 54(9): 2404-2412 doi: 10.1002/aic.11546
|
|
[18]
|
Jin X Z, Yang G H. Robust adaptive fault-tolerant compensation control with actuator failures and bounded disturbances. Acta Automatica Sinica, 2009, 35(3): 305-309
|
|
[19]
|
Song G, Tao G. Adaptive state feedback control with sensor failure compensation for asymptotic output tracking. International Journal of Adaptive Control and Signal Processing, 2019, 33(1): 130-156 doi: 10.1002/acs.2958
|
|
[20]
|
Ye D, Su L, Wang J L, Pan Y N. Adaptive reliable $ H_{\infty}$ optimization control for linear systems with time-varying actuator fault and delays. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2017, 47(7): 1635-1643 doi: 10.1109/TSMC.2017.2656386 optimization control for linear systems with time-varying actuator fault and delays. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2017, 47(7): 1635-1643 doi: 10.1109/TSMC.2017.2656386
|
|
[21]
|
胡庆雷, 张爱华, 李波. 推力器故障的刚体航天器自适应变结构容错控制. 航空学报, 2013, 34(4): 909-918Hu Qing-Lei, Zhang Ai-Hua, Li Bo. Adaptive variable structure fault tolerant control of rigid spacecraft under thruster faults. Acta Aeronautica et Astronautica Sinica, 2013, 34(4): 909-918 (in Chinese)
|
|
[22]
|
Liu L, Liu Y J, Li D P, Tong S C, Wang Z S. Barrier Lyapunov function based adaptive fuzzy FTC for switched systems and its applications to resistance inductance capacitance circuit system. IEEE Transactions on Cybernetics, 2020, 50(8): 3491-3502 doi: 10.1109/TCYB.2019.2931770
|
|
[23]
|
Wang Z S, Liu L, Wu Y M, Zhang H G. Optimal fault-tolerant control for discrete-time nonlinear strict-feedback systems based on adaptive critic design. IEEE Tansactions on Neural Networks and Learning Systems, 2018, 29(6): 2179-2191 doi: 10.1109/TNNLS.2018.2810138
|
|
[24]
|
Wen L Y, Tao G, Yang H, Jiang B. Adaptive Actuator failure compensation for possibly nonminimum-phase systems using control separation based LQ design. IEEE Transactions on Automatic Control, January 2019, 64(1): 143-158 doi: 10.1109/TAC.2018.2809719
|
|
[25]
|
Ataei-Esfahani A, Wang Q. Robust failure compensation for a morphing aircraft model using a probabilistic approach. IEEE Transactions on Control Systems Technology, 2007, 15(2): 324-331 doi: 10.1109/TCST.2006.883188
|
|
[26]
|
Shen Q K, Shi P, Wang S Y, Shi Y. Fuzzy adaptive control of a class of nonlinear systems with unmodeled dynamics. International Journal of Adaptive Control and Signal Processing, 2019, 33(4): 712-730 doi: 10.1002/acs.2980
|
|
[27]
|
后德龙, 王青, 董朝阳. 飞行器切换多胞系统执行机构失效自适应补偿控制. 控制与决策, 2014, 29(12): 2235-2241Hou De-Long, Wang Qing, Dong Chao-Yang. Adaptive actuator failure compensate control for switched polytopic system of flight vehicles. Control and Decision, 2014, 29(12): 2235-2241 (in Chinese)
|
|
[28]
|
于进勇, 陈洁, 周绍磊, 顾文锦. 一类高超声速飞行器自适应容错控制器设计. 中国科学: 信息科学, 2012, 42(11): 1391-1402 doi: 10.1360/112012-455Yu Jin-Yong, Chen Jie, Zhou Shao-Lei, Gu Wen-Jin. Hypersonic aircraft adapative fault-tolerant controller design. Scientia Sinica Informationis, 2012, 42(11): 1391-1402 (in Chinese) doi: 10.1360/112012-455
|
|
[29]
|
Zhang K K, Jiang B, Yan X G, Mao Z H, Polycarpou M M. Fault-tolerant control for systems with unmatched actuator faults and disturbances. IEEE Transactions on Automatic Control, 25 May 2020: 1-1, doi: 10.1109/TAC.2020.2997347
|
|
[30]
|
Sastry S, Bodson M. Adaptive Control Stability Convergence and Robustness, Prentice Hall: Englewood Cliffs, NJ, 1989
|
|
[31]
|
Liu L, Liu Y J, Tong S C, Chen C L P. Integral barrier Lyapunov function based adaptive control for switched nonlinear systems. Science China Information Sciences, March 2020, 63(3): 132203:1-132203:14
|
|
[32]
|
Tang L, Li D J. Time-varying barrier Lyapunov function based adaptive neural controller design for nonlinear pure-feedback systems with unknown hysteresis. International Journal of Control, Automation, and Systems, July 2019, 17(7): 1642-1654 doi: 10.1007/s12555-018-0745-y
|
|
[33]
|
任章, 廉成斌, 熊子豪. 高超声速飞行器强鲁棒自适应控制器设计新方法. 导航定位与授时, 2014, 1(1): 22-30 doi: 10.3969/j.issn.2095-8110.2014.01.005Ren Zhang, Lian Cheng-Bin, Xiong Zi-Hao. Novel design method of strength robustness adaptive controller for hypersonic vehicle. Navigation Positioning & Timing, 2014, 1(1): 22-30 (in Chinese) doi: 10.3969/j.issn.2095-8110.2014.01.005
|
|
[34]
|
Narendra K S, Balakrishnan J. Improving transient response of adaptive control systems using multiple models and switching. IEEE Transactions on Automatic Control, 1994, 39(9): 1861-1866 doi: 10.1109/9.317113
|
|
[35]
|
Narendra K S, Balakrishnan J. Adaptive control using multiple models. IEEE Transactions on Automatic Control, 1997, 42(2): 171-187 doi: 10.1109/9.554398
|
|
[36]
|
Tan C, Tao G, Qi R Y, Yang H. A direct MRAC based multivariable multiple-model switching control scheme. Automatica, 2017, 84: 190-198 doi: 10.1016/j.automatica.2017.07.020
|
|
[37]
|
Yao X L, Yang Y, Su M. A novel adaptive actuator failure compensation scheme based on multi-design integration for half-car active suspension system. Advances in Mechanical Engineering, 2019, 11(12): 1687814019894097.
|
|
[38]
|
Wang C, Wen C, Lin Y. Adaptive actuator failure compensation for a class of nonlinear systems with unknown control direction. IEEE Transactions on Automatic Control, 2016, 62(1): 385-392
|
|
[39]
|
Yang Q, Ge S S, Sun Y. Adaptive actuator fault tolerant control for uncertain nonlinear systems with multiple actuators. Automatica, Oct 1 2015, 60: 92-99 doi: 10.1016/j.automatica.2015.07.006
|
|
[40]
|
Tong S, Huo B, Li Y. Observer-based adaptive decentralized fuzzy fault-tolerant control of nonlinear large-scale systems with actuator failures. IEEE Transactions on Fuzzy Systems, Jan 21 2013, 22(1): 1-5
|
|
[41]
|
Ouyang H, Lin Y. Adaptive fault-tolerant control for actuator failures: A switching strategy. Automatica, Jul 1 2017, 81: 87-95 doi: 10.1016/j.automatica.2017.03.014
|
|
[42]
|
Wang H, Liu X, Liu P X, Li S. Robust adaptive fuzzy fault-tolerant control for a class of non-lower-triangular nonlinear systems with actuator failures. Information Sciences, Apr 1 2016, 336: 60-74 doi: 10.1016/j.ins.2015.12.008
|
|
[43]
|
Wen L Y, Tao G, Yang H, Zhang Y J. An adaptive disturbance rejection control scheme for multivariable nonlinear systems. International Journal of Control, 2016, 89(3): 594-610 doi: 10.1080/00207179.2015.1088172
|





































 下载:
下载: