Control Oriented Modeling and Singular Perturbation Analysis in Flapping-wing Flight
-
摘要: 针对扑翼飞行中的周期性和时标不一现象, 以及扑翼飞行实际控制中的问题, 本文基于奇异摄动理论, 提出了一种针对扑翼周期系统的稳定性分析方法. 具体而言, 首先建立了扑翼飞行器的多刚体模型, 为后文对翅翼动力学的奇异摄动分析铺平道路; 其次, 对多刚体模型进行简化, 抽象出扑翼飞行动力学的核心问题, 并针对实际控制中的问题, 提出了利用奇异摄动理论分析扑翼飞行周期稳定性的方法, 指出了其相对于其他方法的优越性; 最后, 在自制的四自由度扑翼飞行器完成了真实的飞行实验, 验证了所提方法的有效性.Abstract: In view of the periodicity and the time scale difference in flapping flight, as well as the practical problems in the control of flapping flight, a singular perturbation theory based system stability analysis method for periodic flapping-wing motions is proposed in this paper. Firstly, a multi-rigid body model of flapping wing vehicle is established, which paves the way for the singular perturbation analysis of flapping wing dynamics. Secondly, the multi rigid body model is simplified, and the core problem of flapping wing flight dynamics is abstracted. By using the singular perturbation theory, the stability of flapping wing flight periodic orbit is analyzed, and its superiority compared with other methods is discussed. Finally, the experiments of real flight are conducted in the self-made four degrees of freedom flapping wing aircraft, which verify the effectiveness of the proposed method.
-
图 1 不同种类扑翼飞行多刚体模型中的坐标系建立
Fig. 1 The coordinate setup in the multi-rigid body dynamics of different flapping wing flights
图 2 翅翼两级连杆系统中各刚体坐标系之间的旋转平移示意图
Fig. 2 The schematic for the rotations and the translations between different frames of a two-link wing
图 3 扑动翅翼周期轨道控制示意图
Fig. 3 The schematic for the control of flapping-wing periodic orbit
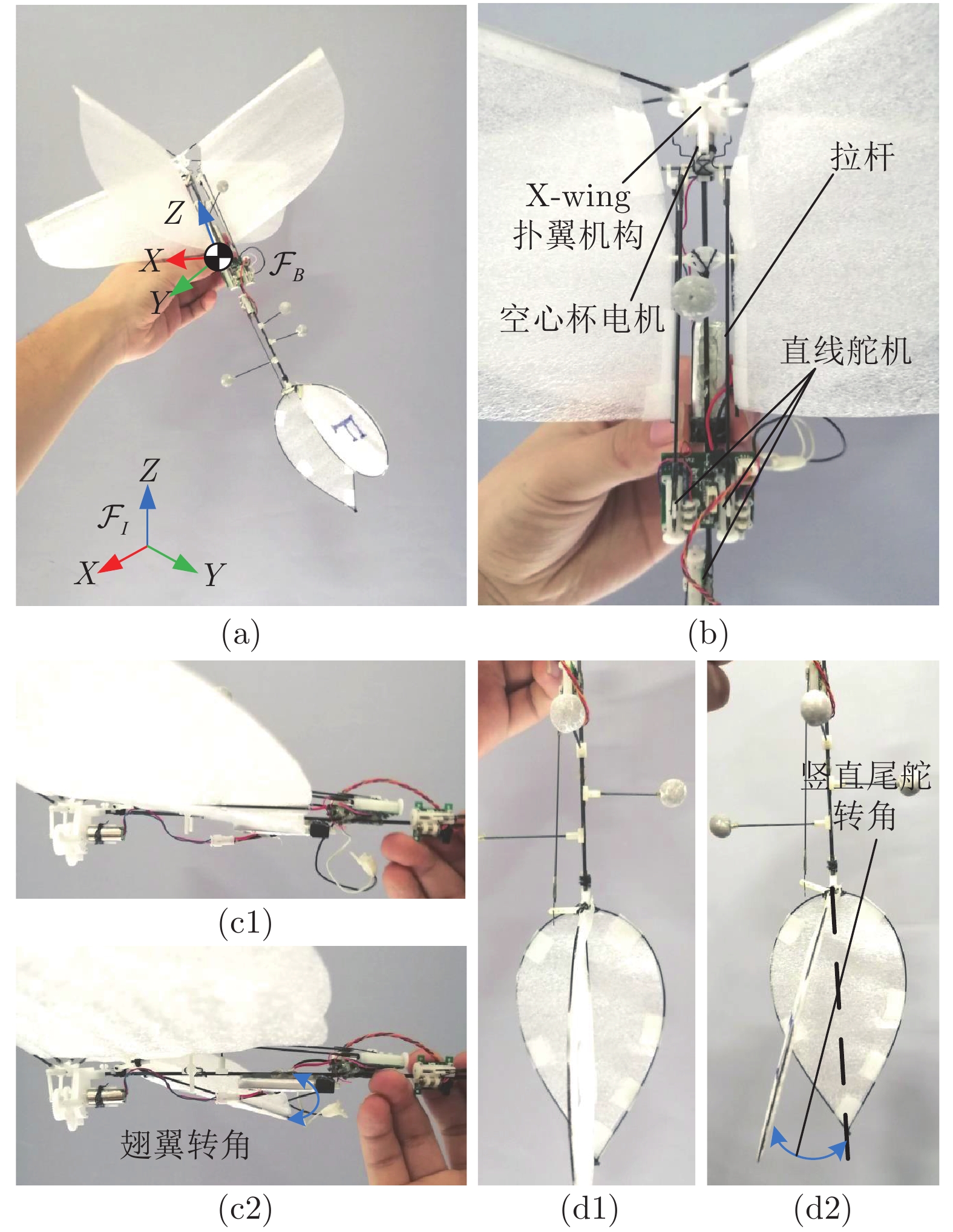
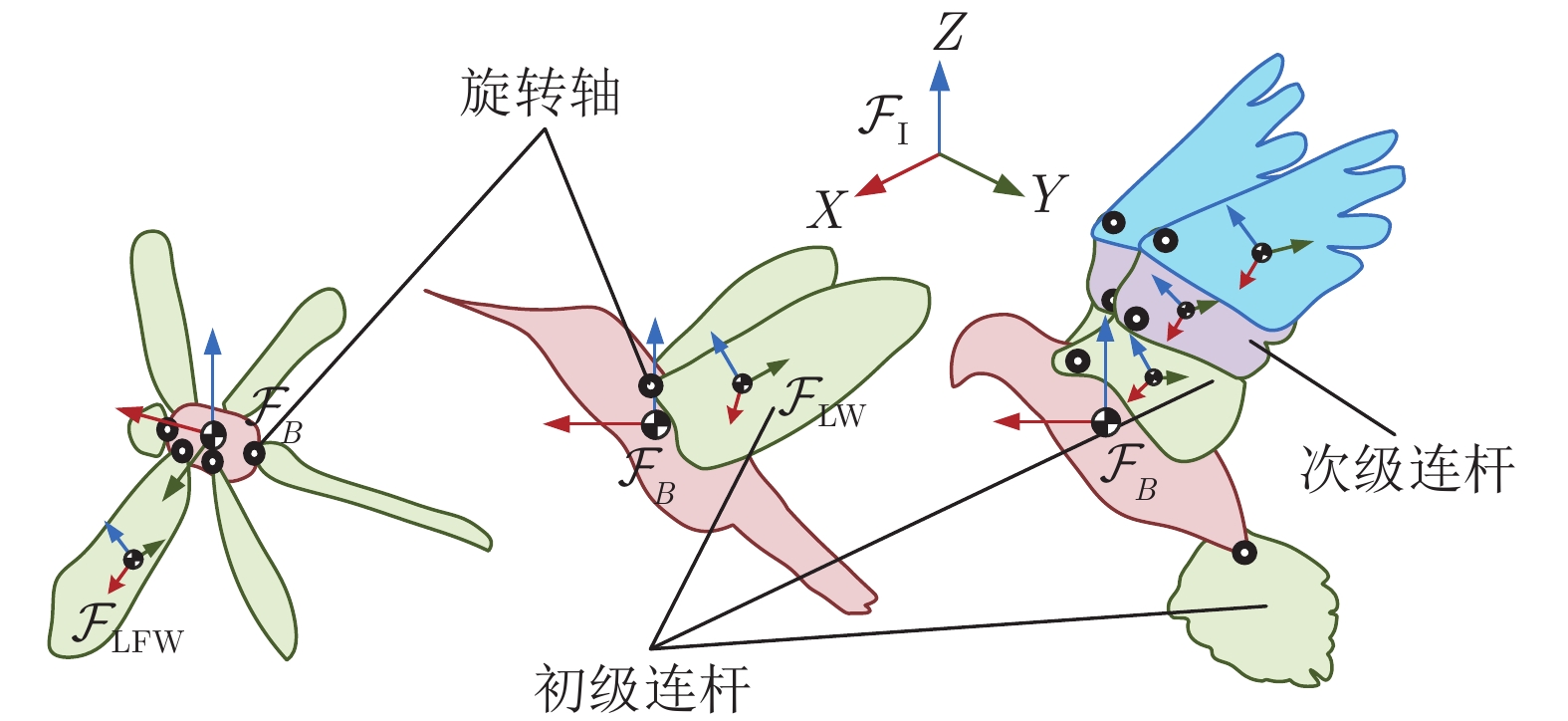
图 4 四自由度扑翼飞行器示意图 ((a)惯性坐标系与机体坐标系设置; (b)翅翼驱动与控制机构; (c1)无偏转的翅翼姿态; (c2)偏转后的翅翼姿态; (d1) 无偏转的尾翼姿态; (d2) 偏转后的尾翼姿态)
Fig. 4 The schematic for the 4-DoF flapping wing vehicle ((a) Inertial coordinate system and body coordinate system setup; (b) Flapping wings actuation and control mechanism; (c1) Flapping wings in default state; (c2) Flapping wings in deflection state; (d1) Tail in default state; (d2) Tail in deflection state)
图 5 运动捕捉系统辅助的四自由度扑翼飞行器实际飞行
Fig. 5 The real flight of the developed 4-DoF flapping wing system with the help of the motion capture system
图 6 不同两翼转角差下悬停飞行时的控制输入与机体姿态
Fig. 6 The control inputs and fuselage attitudes in the hovering flight with different two-wings deflection-angle deviations
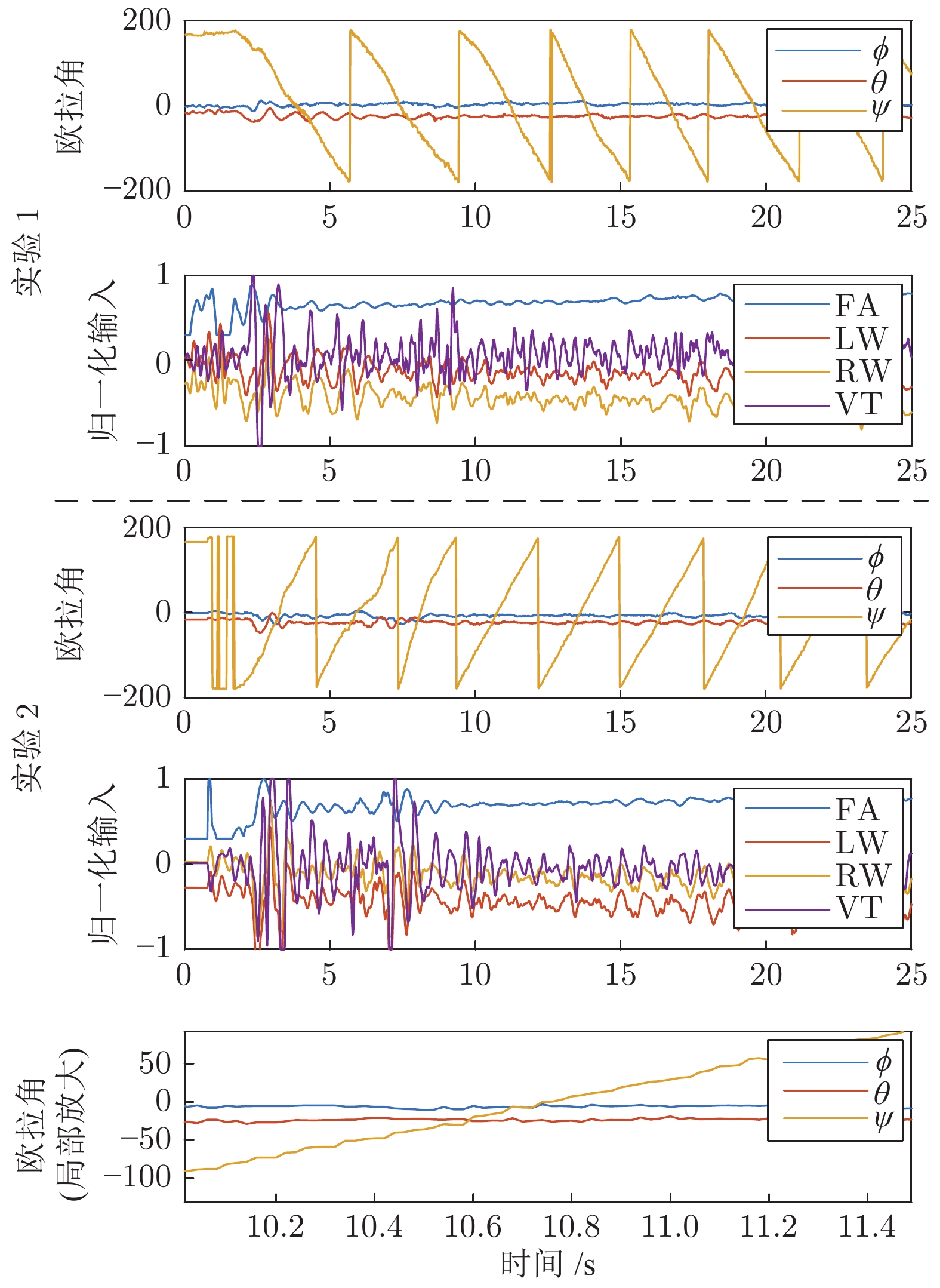
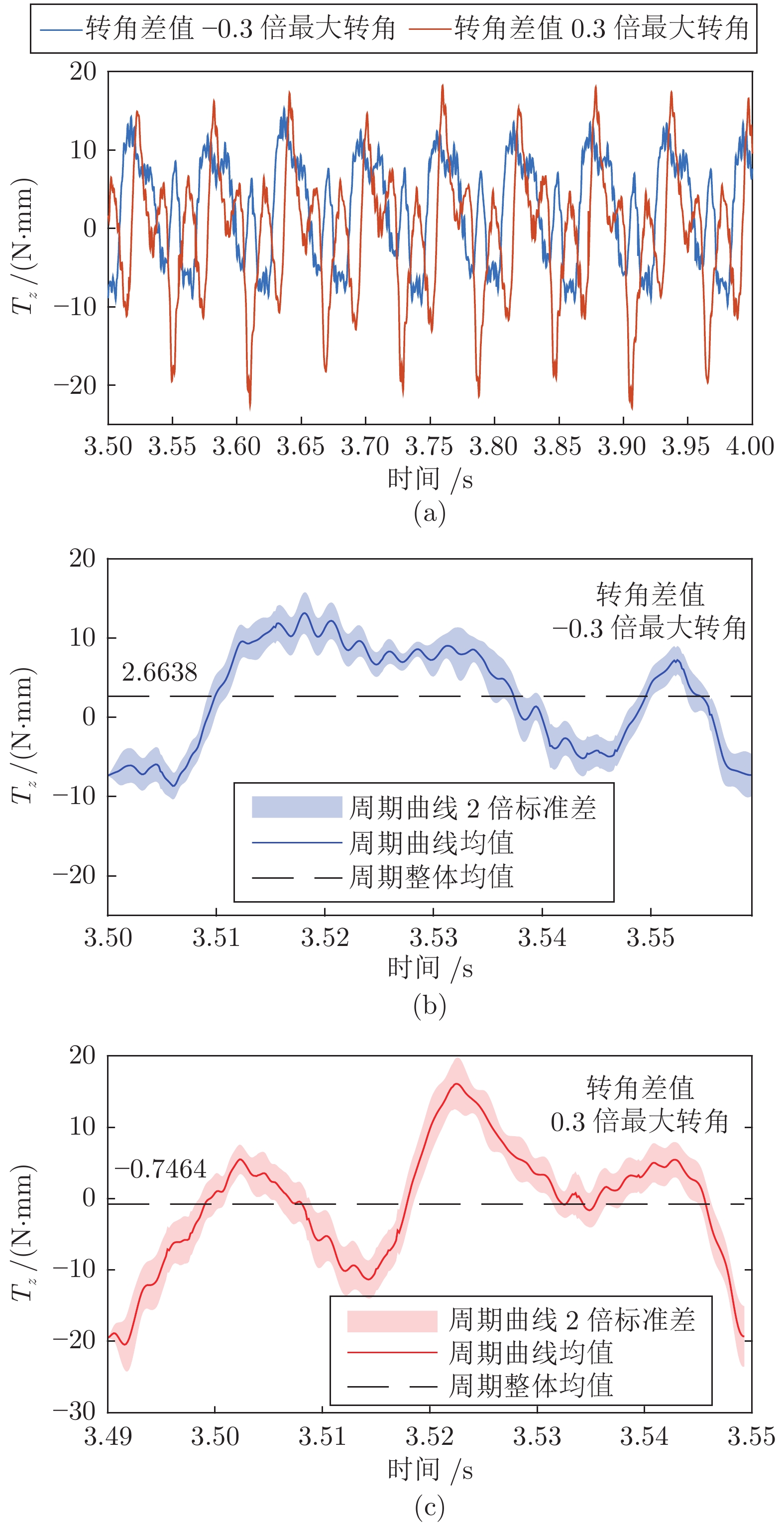
图 8 由翅翼偏转引起的绕z轴周期变化的力矩 ((a)在不同偏转角下力矩的周期变化; (b)转角差值为−0.3倍最大转角的力矩多周期统计特性; (c)转角差值为0.3倍最大转角时的力矩多周期统计特性)
Fig. 8 The periodically changing torque around z-axis induced by the wings deflection ((a) Periodic variation of torque under different deflection angles; (b) Statistical characteristics of the torque with the deflection angle of −0.3 limit angle; (c) Statistical characteristics of the torque with the deflection angle of 0.3 limit angle)
-
[1] 贺威, 丁施强, 孙长银, 扑翼飞行器的建模与控制研究进展. 自动化学报, 2017, 43(5): 685-696.He Wei, Ding Shi-Qiang, Sun Chang-Yin. Research progress on modeling and control of flapping-wing air vehicles. Acta Automatica Sinica, 2017, 43(5): 685-696. [2] 丁其川, 熊安斌, 赵新刚, 韩建达, 基于表面肌电的运动意图识别方法研究及应用综述. 自动化学报, 2016, 42(1): 13-25.Ding Qi-Chuan, Xiong An-Bin, Zhao Xin-Gang, Han Jian-Da. A Review on Researches and Applications of sEMG-based Motion Intent Recognition Methods. Acta Automatica Sinica, 2016, 42(1): 13-25. [3] 李剑锋, 李国通, 张雷雨, 杨东升, 王海东, 穿戴式柔性下肢助力机器人发展现状及关键技术分析. 自动化学报, 2020, 46(3): 427-438.Li Jian-Feng, Li Guo-Tong, Zhang Lei-Yu, Yang Dong-Sheng, Wang Hai-Dong. Advances and Key Techniques of Soft Wearable Lower Limb Power-assisted Robots. Acta Automatica Sinica, 2020, 46(3): 427-438. [4] 王天柱, 吴正兴, 喻俊志, 谭民, 张峰, 冰雪运动生物力学及其机器人研究进展. 自动化学报, 2019, 45(9): 1620-1636.Wang Tian-Zhu, Wu Zheng-Xing, Yu Jun-Zhi, Tan Min, Zhang Feng. Progress and Perspective of Ice and Snow Sport Biomechanics and Related Robots. Acta Automatica Sinica, 2019, 45(9): 1620-1636. [5] Fei, F, Tu Z, Zhang J, Deng X Y. Learning extreme hummingbird maneuvers on flapping wing robots. In: Proceedings of the 2019 International Conference on Robotics and Automation (ICRA). Montreal, Canada: IEEE, 2019. 109−115 [6] Tu, Z, Fei, F, Zhang J, Deng X. An At-Scale Tailless Flapping-Wing Hummingbird Robot. I. Design, Optimization, and Experimental Validation. IEEE Transactions on Robotics, 2020, to be published. [7] He G, Su T, Jia T, Zhao L, Zhao Q. Dynamics Analysis and Control of a Bird Scale Underactuated Flapping-Wing Vehicle. IEEE Transactions on Control Systems Technology, 2020, 28(4): 1233-1242. doi: 10.1109/TCST.2019.2908145 [8] 昂海松, 微型飞行器的设计原则和策略. 航空学报, 2016, 37(1): 69-80.Ang Hai-song, Design principles and strategies of micro air vehicle, Acta Aeronautica et Astronautica Sinica, 2016, 37(1): 69-80. [9] 昂海松, 肖天航, 郑祥明, 微型飞行器的低雷诺数、非定常气动设计与分析, 无人系统技术, 2018(4): 17-32.Ang Hai-song, Xiao Tian-hang, ZHENG Xiang-ming. Design and Analysis of Low Reynolds Number and Unsteady Aerodynamic Based on Micro Air Vehicles, Unmanned Systems Technology, 2018(4): 17-32. [10] 杨文青, 宋笔锋, 宋文萍, 陈利丽, 仿生微型扑翼飞行器中的空气动力学问题研究进展与挑战, 实验流体力学, 2015, 29(3): 1-10.Yang Wen-qing, Song Bi-feng, Song Wen-pin, Chen Li-li. The progress and challenges of aerodynamics in the bionic flapping-wing micro air vehicle, Journal of Experiments in Fluid Mechanics, 2015, 29(3): 1-10. [11] Ellington C P, Van Den Berg C, Willmott A P. Leading-edge vortices in insect flight, Nature, 1996, 384(6610): 626. doi: 10.1038/384626a0 [12] Van Den Berg C, Ellington C P. The three–dimensional leading–edge vortex of a “hovering” model hawkmoth. Philosophical Transactions of the Royal Society of London. Series B: Biological Sciences, 1997, 352(1351): 329-340. doi: 10.1098/rstb.1997.0024 [13] Dickinson M H, Lehmann F O, Sane S P. Wing rotation and the aerodynamic basis of insect flight. Science, 1999, 284(5422): 1954-1960. doi: 10.1126/science.284.5422.1954 [14] Sane S P, Dickinson M H. The aerodynamic effects of wing rotation and a revised quasi-steady model of flapping flight, Journal of experimental biology, 2002, 205(8): 1087-1096. [15] Weis-Fogh T. Quick estimates of flight fitness in hovering animals, including novel mechanisms for lift production[J]. Journal of experimental Biology, 1973, 59(1): 169-230. [16] Miller L A, Peskin C S. Flexible clap and fling in tiny insect flight. Journal of Experimental Biology, 2009, 212(19): 3076-3090. doi: 10.1242/jeb.028662 [17] Percin M, Hu Y, van Oudheusden B W, Remes B, Scarano F. Wing flexibility effects in clap-and-fling. International Journal of Micro Air Vehicles, 2011, 3(4): 217-227. doi: 10.1260/1756-8293.3.4.217 [18] Shyy W, Aono H, Chimakurthi S K, Trizila P, Kang C, Cesnik C, H Liu. Recent progress in flapping wing aerodynamics and aeroelasticity. Progress in Aerospace Sciences, 2010, 46(7): 284-327. doi: 10.1016/j.paerosci.2010.01.001 [19] Chin D D, Lentink D. Flapping wing aerodynamics: from insects to vertebrates. Journal of Experimental Biology, 2016, 219(7): 920-932. doi: 10.1242/jeb.042317 [20] Lee Y J, Lua K B, Lim T T, Yeo K S, A quasi-steady aerodynamic model for flapping flight with improved adaptability. Bioinspiration & biomimetics, 2016, 11(3): 036005. [21] Nabawy M R A, Crowther W J. On the quasi-steady aerodynamics of normal hovering flight part I: the induced power factor. Journal of The Royal Society Interface, 2014, 11(93): 20131196. doi: 10.1098/rsif.2013.1196 [22] Nabawy M R A, Crowther W J. On the quasi-steady aerodynamics of normal hovering flight part II: model implementation and evaluation. Journal of The Royal Society Interface, 2014, 11(94): 20131197. doi: 10.1098/rsif.2013.1197 [23] Nogar S M, Gogulapati A, McNamara J J, Oppenheimer M W, Doman B. Control-oriented modeling of coupled electromechanical-aeroelastic dynamics for flapping-wing vehicles. Journal of Guidance, Control, and Dynamics, 2017, 40(7): 1664-1679. doi: 10.2514/1.G002503 [24] Sun M, Wang J, Xiong Y. Dynamic flight stability of hovering insects. Acta Mechanica Sinica, 2007, 23(3): 231-246. doi: 10.1007/s10409-007-0068-3 [25] Sun M. Insect flight dynamics: stability and control. Reviews of Modern Physics, 2014, 86(2): 615. doi: 10.1103/RevModPhys.86.615 [26] Wu J H, Sun M. Floquet stability analysis of the longitudinal dynamics of two hovering model insects. Journal of the Royal Society Interface, 2012, 9(74): 2033-2046. doi: 10.1098/rsif.2012.0072 [27] Taha H E, Tahmasian S, Woolsey C A, Nayfeh A H, Muhammad R H. The need for higher-order averaging in the stability analysis of hovering, flapping-wing flight. Bioinspiration & biomimetics, 2015, 10(1): 016002. [28] Cheng B, Deng X. Translational and rotational damping of flapping flight and its dynamics and stability at hovering. IEEE Transactions on Robotics, 2011, 27(5): 849-864. doi: 10.1109/TRO.2011.2156170 [29] Zhang J, Cheng B, Deng X. Instantaneous wing kinematics tracking and force control of a high-frequency flapping wing insect MAV. Journal of Micro-Bio Robotics, 2016, 11(1-4): 67-84. doi: 10.1007/s12213-015-0085-4 [30] Karásek M, Muijres F T, De Wagter C, Remes B, de Croon G. A tailless aerial robotic flapper reveals that flies use torque coupling in rapid banked turns. Science, 2018, 361(6407): 1089-1094. doi: 10.1126/science.aat0350 [31] Jafferis N T, Helbling E F, Karpelson M, Wood R. Untethered flight of an insect-sized flapping-wing microscale aerial vehicle. Nature, 2019, 570(7762): 491. doi: 10.1038/s41586-019-1322-0 [32] Chen Y, Wang H, Helbling E F, Jafferis N T, Zufferey R, Ong A, et al. A biologically inspired, flapping-wing, hybrid aerial-aquatic microrobot. Science Robotics, 2017, 2(11): eaao5619. doi: 10.1126/scirobotics.aao5619 [33] Ramezani A, Shi X C, Chung S J, Hutchinson S. Nonlinear flight controller synthesis of a bat-inspired micro aerial vehicle. In: Proceedings of the 2016 AIAA Guidance, Navigation, and Control Conference. San Diego, USA: AIAA, 2016. [34] Ramezani A, Shi X C, Chung S J, Hutchinson S. Lagrangian modeling and flight control of articulated-winged bat robot. In: Proceedings of the 2015 IEEE/RSJ International Conference on Intelligent Robots and Systems. Hamburg, Germany: IEEE/RSJ 2015. 2867−2874 [35] Orlowski C T, Girard A R. Longitudinal flight dynamics of flapping-wing micro air vehicles. Journal of Guidance, Control, and Dynamics, 2012, 35(4): 1115-1131. doi: 10.2514/1.55923 [36] Orlowski C T, Girard A R. Dynamics, stability, and control analyses of flapping wing micro-air vehicles. Progress in Aerospace Sciences, 2012, 51: 18-30. doi: 10.1016/j.paerosci.2012.01.001 [37] Hassan A M, Taha H E. Differential-geometric-control formulation of flapping flight multi-body dynamics. Journal of Nonlinear Science, 2019, 29(4): 1379-1417. doi: 10.1007/s00332-018-9520-8 [38] Fenichel N. Geometric singular perturbation theory for ordinary differential equations. Journal of differential equations, 1979, 31(1), 53-98. doi: 10.1016/0022-0396(79)90152-9 [39] Hek G. Geometric singular perturbation theory in biological practice. Journal of Mathematical Biology, 2010, 60(3), 347-386. doi: 10.1007/s00285-009-0266-7 [40] Farkas M. Periodic Motions. Springer Science and Business Media, 2013. [41] Qian C, Fang Y, Li Y. Quaternion-Based Hybrid Attitude Control for an Under-Actuated Flapping Wing Aerial Vehicle. IEEE/ASME Transactions on Mechatronics, 2019, 24(5): 2341-2352. doi: 10.1109/TMECH.2019.2930584 [42] Phan H V, Park H C. Generation of control moments in an insect-like tailless flapping-wing micro air vehicle by changing the stroke-plane angle. Journal of Bionic Engineering, 2016, 13(3): 449-457. doi: 10.1016/S1672-6529(16)60318-9 -
下载:
计量
- 文章访问数: 861
- HTML全文浏览量: 461
- PDF下载量: 234
- 被引次数: 0
