Distributed Event-triggered Fixed-time Scaled Consensus Control for Second-order Multi-agent Systems
-
摘要: 研究了在无向拓扑下, 由多个子群组成的二阶多智能体系统的固定时间比例一致性问题, 采用反推法设计了一种基于事件触发的固定时间非线性比例一致控制策略, 该策略包含分段式事件触发函数: 当智能体在追踪虚拟速度时, 给出了基于速度信息的触发条件; 当智能体速度与虚拟速度达到一致时, 切换至基于位置信息的触发条件, 可有效减少系统能量耗散及控制器更新频次. 通过在位置和速度状态上设置比例参数, 在固定时间内可实现不同子群智能体之间的比例一致. 利用代数图论、线性矩阵不等式以及Lyapunov稳定性理论, 证明在该控制策略下, 二阶多智能体系统能实现固定时间比例一致性, 且不存在Zeno行为. 最后, 仿真实例进一步验证了理论结果的有效性.Abstract: The problem of fixed-time proportional consensus of second-order multi-agent systems composed of multiple subgroups under undirected topology is studied. A fixed-time non-linear proportional consensus control strategy based on event triggering is designed by using backstepping method. The strategy includes a piecewise event-triggering function. When an agent tracks virtual speed, a trigger bar based on velocity information is given. When the agent achieves the same virtual speed, switching to the event trigger condition based on location information can efiectively reduce the energy dissipation of the system and the update frequency of the controller. By setting proportional parameters in the position and speed state, the proportional consensus among different sub-group agents can be achieved in a fixed time. The Lyapunov stability theory, linear matrix inequality and algebraic graph theory are used to prove the control strategy. Second-order multi-agent systems can achieve fixed-time scaled consensus without the Zeno behavior. The simulation results further verify the validity of the theoretical results.
-
图 5 智能体1在触发条件(17)下的测量误差及阈值变化趋势
Fig. 5 The evolution of the error norm and the threshold of agent 1 with trigger function (17)
图 6 智能体1在触发条件(25)下的测量误差及阈值变化趋势
Fig. 6 The evolution of the error norm and the threshold of agent 1 with trigger function (25)
图 7 各智能体在控制策略(10)下的触发间隔及在时间触发控制策略下的触发间隔
Fig. 7 The triggered interval of each agent undercontrol scheme (10) and the trigger interval underthe time trigger control strategy
-
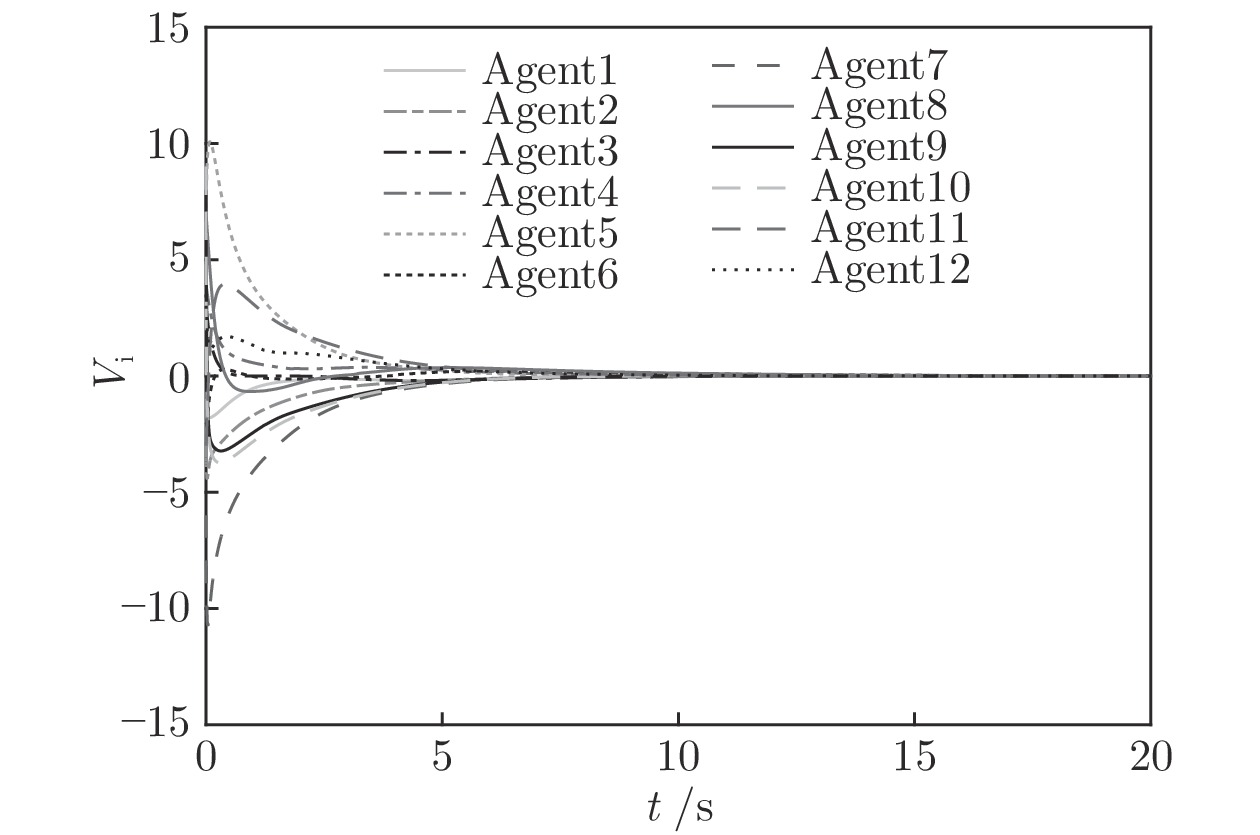
[1] Thunberg J, Goncalves J, Hu X. Consensus and formation control on SE(3) for switching topologies[J]. Automatica, 2016, 66(5): 63-82. [2] Xiao F, Wang L, Chen J, Gao Y P. Finite-time formation control for multi-agent systems[J]. Automatica, 2009, 45(11): 2605-2611. doi: 10.1016/j.automatica.2009.07.012 [3] Martin S. Multi-agent flocking under topological interactions[J]. Systems & Control Letters, 2014, 69(1): 53-61. [4] 陈世明, 化俞新, 祝振敏, 赖强. 邻域交互结构优化的多智能体快速蜂拥控制算法[J]. 自动化学报, 2015, 41(12): 2092-2099.CHEN Shi-Ming, HUA Yu-Xin, ZHU Zhen-Min, LAI Qiang. Fast flocking algorithm for multi-agent systems by opti-mizing local interactive topology[J]. Acta Automatica Sinica, 2015, 41(12): 2092-2099. [5] Huang N, Duan Z, Zhao Y. Consensus of multi-agent systems via delayed and intermittent communications[J]. IET Control Theory and Applications, 2015, 9(1): 62-73. doi: 10.1049/iet-cta.2014.0729 [6] Zhao M, Peng C, He W L, Song Y. Event-triggered communication for leader-following consensus of second-order multiagent systems[J]. IEEE Transactions on Cybernetics, 2018, 48(6): 1888-1897. doi: 10.1109/TCYB.2017.2716970 [7] Li J P, Yang Y N, Hua C C, Guan X P. Fixed-time backstepping control design for high-order strict-feedback non-linear systems via terminal sliding mode[J]. IET Control Theory and Applications, 2017, 11(8): 1184-1193. doi: 10.1049/iet-cta.2016.1143 [8] Wang H H, Chen B, Lin C, Sun Y M, Wang F. Adaptive flnite-time control for a class of uncertain high-order nonlinear systems based on fuzzy approximation[J]. IET Control Theory and Applications, 2017, 11(5): 677-684. doi: 10.1049/iet-cta.2016.0947 [9] Paulo T. Event-triggered real-time scheduling of stabilizing control tasks[J]. IEEE Transactions on Automatic Control, 2007, 52(9): 2680-1685. [10] Dimos V D, Emilio F, Karl H J. Distributed event-triggered control for multi-agent systems[J]. IEEE Transactions on Automatic Control, 2012, 57(5): 1291-1297. doi: 10.1109/TAC.2011.2174666 [11] Hu A H, Cao J, Hu M F, Guo L X. Event-triggered consensus of multi-agent systems with noises[J]. Journal of the Franklin Institute, 2015, 352(9): 3489-3503. doi: 10.1016/j.jfranklin.2014.08.005 [12] Fan Y, Feng G, Wang Y, Song C. Distributed eventtriggered control of multi-agent systems with combinational measurements[J]. Automatica, 2013, 49(2): 671-675. doi: 10.1016/j.automatica.2012.11.010 [13] Hu A H, Cao J. Consensus of multi-agent systems via intermittent event-triggered control[J]. International Journal of Systems Science, 2017, 48(2): 280-287. doi: 10.1080/00207721.2016.1179817 [14] Zhu Y K, Guan X P, Luo X Y, Li S B. Finite-time consensus of multi-agent system via nonlinear event-triggered control strategy[J]. IET Control Theory and Applications, 2015, 9(17): 2548-2552. doi: 10.1049/iet-cta.2014.0533 [15] Yu S H, Wang Y L, Jin L N. Comments on ’Finite-time consensus of multi-agent system via non-linear event-triggered control strategy’[J]. IET Control Theory and Applications, 2017, 11(10): 1658-1661. doi: 10.1049/iet-cta.2016.0955 [16] Dong Y, Xian J G. Finite-time event-triggered consensus for non-linear multi-agent networks under directed network topology[J]. IET Control Theory and Applications, 2017, 11(15): 2458-2464. doi: 10.1049/iet-cta.2017.0085 [17] Liu J, Yu Y, Sun J, Sun C Y. Distributed event-triggered fixed-time consensus for leader-follower multiagent systems with nonlinear dynamics and uncertain disturbances[J]. International Journal of Robust and Nonlinear Control, 2018, 28(11): 3543-3559 doi: 10.1002/rnc.4098 [18] Roy S. Scaled consensus[J]. Automatica, 2015, 51 : 259-262. doi: 10.1016/j.automatica.2014.10.073 [19] Meng D, Jia Y. Scaled consensus problems on switching networks[J]. IEEE Transactions on Automatic Control, 2016, 61(6): 1664-1669. doi: 10.1109/TAC.2015.2479119 [20] Yu J, Shi Y. Scaled group consensus in multiagent systems with flrst/second-order continuous dynamics[J]. IEEE Transactions on Cybernetics, 2018, 48(8): 2259-2271. doi: 10.1109/TCYB.2017.2731601 [21] Meng D, Jia Y. Robust consensus algorithms for multiscale coordination control of multi-vehicle systems with disturbances[J]. IEEE Transactions on Industrial Electronics, 2016, 63(2): 1107-1119. doi: 10.1109/TIE.2015.2478740 [22] Olfatisaber R, Murray R M. Consensus problems in networks of agents with switching topology and timedelays[J]. IEEE Transactions on Automatic Control 2004, 49(9): 1520-1533. doi: 10.1109/TAC.2004.834113 [23] Ning B, Jin J, Zheng J C, Man Z H. Finite-time and fixed-time leader-following consensus for multi-agent systems with discontinuous inherent dynamics[J]. International Journal of Control, 2017, 91(6): 1259-1270. [24] Zuo Z, Lin T. A new class of flnite-time nonlinear consensus protocols for multi-agent systems[J]. International Journal of Control, 2014, 87(2): 363-370. doi: 10.1080/00207179.2013.834484 [25] Wei X Y, Yu W W, Wang H, Yao Y Y, Mei F. An observer based fixed-time consensus control for second-order multi-agent systems with disturbances[J]. IEEE Transactions on Circuits and Systems Ⅱ Express Briefs, 2019, 66(2): 247-251. doi: 10.1109/TCSII.2018.2831922 -
下载:
图(12)
计量
- 文章访问数: 953
- HTML全文浏览量: 226
- PDF下载量: 319
- 被引次数: 0
