单量子比特系统状态的在线估计

唐雅茹; 丛爽; 杨靖北

doi:10.16383/j.aas.c180752

单量子比特系统状态的在线估计

doi: 10.16383/j.aas.c180752

唐雅茹^1,,
丛爽^1, ,,
杨靖北^1,

1.
中国科学技术大学自动化系合肥 230027

基金项目:

国家自然科学基金 61973290

详细信息

作者简介:
唐雅茹中国科学技术大学自动化系硕士研究生. 2017年获得合肥工业大学学士学位.主要研究方向为量子态估计以及开放量子系统控制. E-mail: yrtang@mail.ustc.edu.cn

杨靖北北京理工大学计算机学院副研究员. 2005年获得中国科学技术大学博士学位.主要研究方向为信息抽取和情感分析. E-mail: banbei99@mail.ustc.edu.cn

通讯作者:
丛爽中国科学技术大学自动化系教授. 1995年获得意大利罗马大学系统工程博士学位.主要研究方向为运动控制中的先进控制策略, 模糊逻辑控制, 神经网络设计与应用, 机器人协调控制以及量子系统控制.本文通信作者. E-mail: scong@ustc.edu.cn

计量
- 文章访问数: 1197
- HTML全文浏览量: 157
- PDF下载量: 123
- 被引次数: 0
出版历程
- 收稿日期: 2018-11-12
- 录用日期: 2019-04-07
- 刊出日期: 2020-08-26

On-line State Estimation of One-qubit System

1.
Department of Automation, University of Science and Technology of China, Hefei 230027

Funds:

National Natural Science Foundation of China 61973290

More Information

Author Bio:
TANG Ya-Ru Master student in the Department of Automation, University of Science and Technology of China. She received her bachelor degree from Hefei University of Technology in 2017. Her research interest covers online quantum state estimation and open quantum systems control

YANG Jing-Bei Ph.D. in the Department of Automation, University of Science and Technology of China. He received his Ph. D. degree from the Department of Automation, University of Science and Technology of China in 2018. His research interest covers quantum measurement and quantum systems control

Corresponding author: CONG Shuang Professor in the Department of Automation, University of Science and Technology of China. She received her Ph. D. in system engineering from the University of Rome "Sapienza", Rome, Italy, in 1995. Her research interest covers advanced control strategies for motion control, fuzzy logic control, neural networks design and applications, robotic coordination control, and quantum systems control. Corresponding author of this paper

摘要

摘要: 针对具有退相干效应与测量反馈随机噪声的随机开放量子系统, 采用对状态影响较弱的连续弱测量在线获取一系列状态的部分信息, 实现量子状态的在线估计.由泡利矩阵构造初始测量算符, 并推导出在线的随时间变化的测量算符; 基于压缩传感理论来减少测量次数; 采用最小二乘优化算法对自由演化中的量子密度矩阵状态进行重构, 完整地给出了量子态在线估计的过程.所提出的在线量子态估计方案, 在一个量子位系统上进行了系统仿真实验.数值仿真实验结果表明, 在满足压缩传感理论的条件下, 仅需2次连续弱测量所得到的测量值之后, 就可以高精度地实现在线变化的单比特量子密度矩阵估计.
- 单量子比特系统 /
- 量子连续弱测量 /
- 压缩传感理论 /
- 量子态在线估计
Abstract: For a stochastic open quantum system with decoherence efiect and measurement feedback random noise, the continuous weak measurement is used to obtain state information on the line to realize the online estimation of quantum state. The Pauli matrix is used to construct the initial measurement operator, and online time-varying measurement operator is derived. The compressed sensing theory is used to reduce the number of measurements. The least squares optimization algorithm is used to reconstruct the state of the quantum density matrix in free evolution, and the whole process of online estimation of quantum state is derived. The proposed online estimation scheme of quantum state is carried out on the one-qubit system. The numerical simulation results show that under the condition of satisfying the compressed sensing theory, the quantum density matrix estimation of high-precision online of one-qubit can be achieved only after 2 measurements of continuous weak measurement.
- One-qubit system /
- quantum continuous weak measurement /
- compressed sensing theory /
- online estimation of quantum state
Recommended by Associate Editor ZHU Ji-Hong
注释:

1) 本文责任编委朱纪洪

HTML全文

图 1 基于连续弱测量的量子态在线估计过程

Fig. 1 Structure of online quantum state estimation based on continuous weak measurement

下载: 全尺寸图片幻灯片

图 2 量子间接弱测量过程

Fig. 2 Process of quantum indirect weak measurement

下载: 全尺寸图片幻灯片

图 3 无外加控制作用下系统的自由演化轨迹及其在线状态估计

Fig. 3 Free evolution trajectory and online state estimation of the system without external control

下载: 全尺寸图片幻灯片

图 4 相互作用强度为0.3情况下, 外加恒定控制下系统的状态演化轨迹

Fig. 4 State evolution trajectory of system under external constant control under interaction strength of 0.3

下载: 全尺寸图片幻灯片

图 5 相互作用强度为0.5情况下, 外加恒定控制下系统的状态演化轨迹

Fig. 5 State evolution trajectory of system under external constant control under interaction strength of 0.5

下载: 全尺寸图片幻灯片

图 6 量子态在线估计的性能曲线

Fig. 6 Performances of online quantum state estimation

下载: 全尺寸图片幻灯片

参考文献(17)

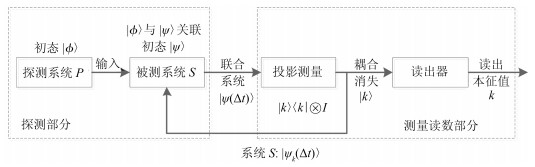
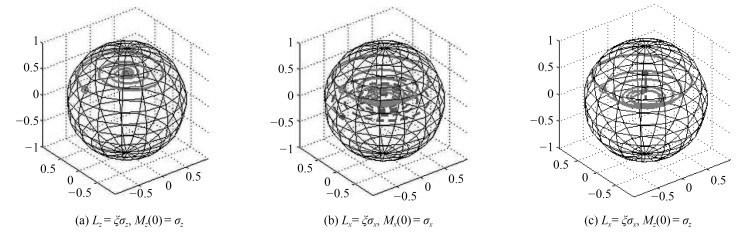
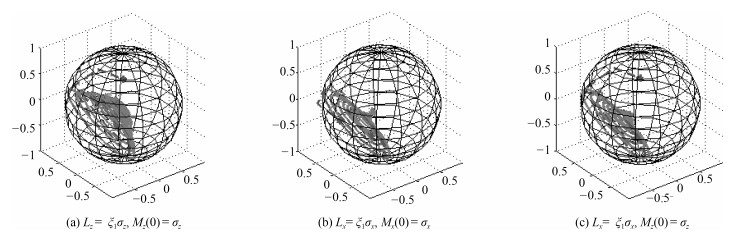
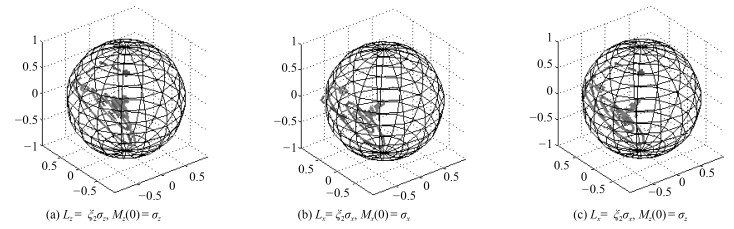
[1]	丛爽, 胡龙珍, 杨霏, 刘建秀. Non-Markovian开放量子系统的特性分析与状态转移.自动化学报, 2013, 39(4): 360-370 doi: 10.3724/SP.J.1004.2013.00360 Cong Shuang, Hu Long-Zhen, Yang Fei, Liu Jian-Xiu. Characteristics analysis and state transfer for non-markovian open quantum systems. Acta Automatica Sinica, 2013, 39(4): 360-370 doi: 10.3724/SP.J.1004.2013.00360
[2]	Vogel K, Risken H. Determination of quasiprobability distributions in terms of probability distributions for the rotated quadrature phase. Physical Review A, 1989, 40: 2847-2849 doi: 10.1103/PhysRevA.40.2847
[3]	Gambetta J, Wiseman H M. State and dynamical parameter estimation for open quantum systems. Physical Review A, 2001, 64: 042105 doi: 10.1103/PhysRevA.64.042105
[4]	Silberfarb A, Jessen P S, Deutsch I H. Quantum state reconstruction via continuous measurement. Physical Review Letters, 2005, 95: 030402 doi: 10.1103/PhysRevLett.95.030402
[5]	Zhang J, Liu Y X, Wu R B, Jacobs K, Nori F. Quantum feedback: theory, experiments, and applications. Physics Reports, 2017, 679: 1-60 doi: 10.1016/j.physrep.2017.02.003
[6]	Harraz S, Cong S. State transfer via on-line state estimation and Lyapunov-based feedback control for a N-qubit system. Entropy, 2019, 21(8): 751 doi: 10.3390/e21080751
[7]	D'Alessandro D. On quantum state observability and measurement. Journal of Physics A: Mathematical and General, 2003, 36(37): 9721-9735 doi: 10.1088/0305-4470/36/37/310
[8]	Chuang I L, Gershenfeld N, Kubinec M. Experimental implementation of fast quantum searching. Physical Review Letters, 1998, 80(15): 3408-3411 doi: 10.1103/PhysRevLett.80.3408
[9]	Candès E J, Romberg J, Tao T. Robust uncertainty principles: Exact signal reconstruction from highly incomplete frequency information. IEEE Transactions on information theory, 2006, 52(2): 489-509 http://ieeexplore.ieee.org/document/1580791
[10]	Zheng K, Li K Z, and Cong S. A reconstruction algorithm for compressive quantum tomography using various measurement sets. Scientific Reports, 2016, 6: 38497 doi: 10.1038/srep38497
[11]	Yang J B, Cong S, Shuang F, Rabitz H. Manipulations between eigenstates of 2-level quantum system based on the optimal measurement. IEEE/CAA Journal of Automatica Sinica, 2016, 3(1): 35-41 doi: 10.1109/JAS.2016.7373760
[12]	Gross D. Recovering low-rank matrices from few coefficients in any basis. IEEE Transactions on Information Theory, 2011, 57(3): 1548-1566 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=e8839ae100493f89b0ecb767df21303e
[13]	Smith A, Riofrío, C. A, Anderson B E, et al. Quantum state tomography by continuous measurement and compressed sensing. Physical Review A, 2012, 87(3): 184-191 http://arxiv.org/abs/1208.5015
[14]	Youssry A, Ferrie C, Tomamichel M. Efficient online quantum state estimation using a matrix-exponentiated gradient method. New Journal of Physic, 2019, 21: 033006 doi: 10.1088/1367-2630/ab0438
[15]	Wiseman H M, Miburn G J. Quantum measurement and control. Cambridge University Press, 2010
[16]	D'Ariano G M, Paris M G A, Sacchi M F. Quantum tomographic methods. Lecture Notes in Physics, 2004, 649: 7-58 doi: 10.1007/978-3-540-44481-7_2
[17]	Cahill K E, Glauber R J. Density operators and quasiprobability distributions. Physical Review, 1969, 177(5): 1882-1902 http://adsabs.harvard.edu/abs/1969PhRv..177.1882C