一种带时间窗口的双脉冲控制器下的忆阻振荡系统滞同步

吴鸿娟; 熊江; 冯玉明; 涂正文

doi:10.16383/j.aas.c180686

一种带时间窗口的双脉冲控制器下的忆阻振荡系统滞同步

doi: 10.16383/j.aas.c180686

吴鸿娟^{1, 2, ,},
熊江^{1, 2,},
冯玉明^{1, 2,},
涂正文^{1, 3,}

1.
重庆三峡学院智能信息处理与控制重庆高校市级重点实验室重庆 404120
2.
重庆三峡学院计算机科学与工程学院重庆 404120
3.
重庆三峡学院数学与统计学院重庆 404120

基金项目:

国家自然科学基金 11601047

重庆市教育委员会科学技术研究项目 KJQN201901205

重庆市基础研究与前沿探索项目 cstc2018jcyjAX0588

重庆市发展和改革委员会 2017[1007]

详细信息

作者简介:
熊江重庆三峡学院教授.重庆大学自动化学院工学硕士. 2002年9月~ 2003年7月, 在华东师范大学MMIT实验室作国内访问学者. 2011年起担任重庆三峡学院计算机科学与工程学院院长.主要研究方向为控制理论, 无线局域网, 信息安全, 物联网, 嵌入式应用. E-mail: xjcq123@sohu.com

冯玉明重庆三峡学院教授, 2016年获得应用数学专业博士学位. 2012年1月~ 2012年10月以及2014年12月~ 2015年4月分别在意大利Udine University以及卡塔尔Texas A & M University at Qatar进行研究访问.主要研究方向为脉冲控制理论, 神经网络, 混沌控制与同步, 图像加密, 超代数, 超图. E-mail: yumingfeng25928@163.com

涂正文重庆三峡学院副教授, 2018年获得数学方向博士学位. 2011年7月开始在重庆三峡学院数学与统计学院任教.主要研究方向为动力系统的稳定性, 神经网络的动力学行为分析.E-mail: tuzhengwen@163.com

通讯作者:
吴鸿娟重庆三峡学院副教授. 2010年获得计算机技术专业硕士学位. 2014年9月~ 2015年7月以及2015年11月~ 2016年5月分别在东南大学以及美国University of California, San Diego访学.主要研究方向为混沌控制与同步, 神经网络, 数据挖掘, 数据库应用系统.本文通信作者.E-mail: juan10329@163.com

计量
- 文章访问数: 1475
- HTML全文浏览量: 64
- PDF下载量: 96
- 被引次数: 0
出版历程
- 收稿日期: 2018-10-22
- 录用日期: 2019-01-18
- 刊出日期: 2020-07-24

Lag Synchronization of Memristor Oscillator Systems via a Double Impulsive Controller With Time Windows

WU Hong-Juan^{1, 2
, ,},
XIONG Jiang^{1, 2
,},
FENG Yu-Ming^{1, 2
,},
TU Zheng-Wen^{1, 3
,}

1.
Key Laboratory of Intelligent Information Processing and Control of Chongqing Municipal Institutions of Higher Education, Chongqing Three Gorges University, Chongqing 404120
2.
School of Computer Science and Engineering, Chongqing Three Gorges University, Chongqing 404120
3.
School of Mathematics and Statistics, Chongqing Three Gorges University, Chongqing 404120

Funds:

National Natural Science Foundation of China 11601047

Scientiflc and Technological Research Program of Chongqing Municipal Education Commission KJQN201901205

Chongqing Cutting-edge and Applied Foundation Research Program cstc2018jcyjAX0588

Program of Chongqing Development and Reform Commission 2017[1007]

More Information

Author Bio:
XIONG Jiang Professor at Chongqing Three Gorges University (CTGU). He obtained his master degree in Engineering from School of Automation, Chongqing University, Chongqing, China. From Sep. 2002 to Jul. 2003, he has served as a visiting scholar with MMIT Laboratory, East China Normal University, Shanghai, China. From 2011, he has been appointed as dean of School of Computer Science and Engineering of CTGU. His research interest covers control theory, WLAN, information security, internet of things, embedded application

FENG Yu-Ming Professor at Chongqing Three Gorges University (CTGU). He received his Ph. D. degree in applied mathematics in 2016. From Jan. 2012 to Oct. 2012 and from Dec. 2014 to Apr. 2015, he has served as a research scholar in Udine University, Udine, Italy and in Texas A & M University at Qatar, Doha, Qatar, respectively. His research interest covers impulsive control theory, neural networks, chaos control and synchronization, image encryption, hyperalgebras, and hypergraphs

TU Zheng-Wen Associate professor at Chongqing Three Gorges University (CTGU). He received his Ph. D. degree in mathematics in 2018. Since Jul. 2011, he has been with the School of Mathematics and Statistics of CTGU. His research interest covers stability of dynamical systems and dynamical behaviors of neural networks

Corresponding author: WU Hong-Juan Associate professor at Chongqing Three Gorges University. She received her master degree in computer technology in 2010. From Sep. 2014 to Jul. 2015 and from Nov., 2015 to May, 2016, she has served as a visiting scholar with Southeast University, Nanjing, China and with University of California, San Diego, USA, respectively. Her research interest covers chaos control and synchronization, neural networks, data mining, and database application system. Corresponding author of this paper

摘要

摘要: 在真实的环境中实现复杂忆阻振荡系统的同步时, 因为信息干扰及通信问题, 驱动和响应系统之间总是存在信息传输时滞, 即时滞问题具有普遍性; 另外, 脉冲控制信号的输入总是存在输入误差, 并不能实现精确地输入.本文考虑到上述实际存在的信息传输时滞和脉冲输入误差, 设计了一种比较接近真实情况的、灵活的可以带有不同时间窗口和不同控制增益的双脉冲切换控制器, 并且利用该控制器实现了两个复杂忆阻振荡系统的滞同步.基于Lyapunov稳定性理论、矩阵不等式以及脉冲控制等相关理论, 本文找出了实现一类五阶复杂忆阻振荡系统同步的条件.最后的仿真实验进一步验证了本控制方法的可行性.
- 忆阻振荡系统 /
- 同步 /
- 传输时滞 /
- 时间窗口 /
- 双脉冲控制器
Abstract: In real environment, while realizing the synchronization of complex memristor oscillators, there are always some transmission delays between master system and slave system because of the signal interference and some communication problem. That is, the delay problem is so common. On the other hand, there are also some input errors because the impulsive control signals can not be input so accurately. Considering the transmission delay and the input error, we design one flexible double impulsive switch controller, which can reflect the real environment better, with two time windows and two control gains, then use it to realize the lag synchronization of two complex memristor oscillator systems. Based on Lyapunov stability theory, matrix inequality, and impulsive control with time windows, synchronization conditions for one type of flve-order complex memristor oscillator systems are given. Simulation results are displayed to verify the feasibility of this method.
- Memristor oscillator system /
- synchronization /
- transmission delay /
- time window /
- double impulsive controller
Recommended by Associate Editor MEI Sheng-Wei
注释:

1) 本文责任编委梅生伟

HTML全文

图 1 五阶忆阻振荡系统模型

Fig. 1 The model of five-order memristor oscillator system

下载: 全尺寸图片幻灯片

图 2 五阶忆阻振荡系统的混沌吸引子

Fig. 2 Attractors of a five-order memristor oscillator system

下载: 全尺寸图片幻灯片

图 3 带时间窗口的双脉冲切换控制器

Fig. 3 A double-impulsive switch controller with time windows

下载: 全尺寸图片幻灯片

图 4 当传输时滞τ= 0.3时, 利用带时间窗口的双脉冲切换控制器实现两个忆阻振荡系统的滞同步情况

Fig. 4 Lag synchronization of the two memristor oscillators via a double-impulsive switch controller with time windows and the transmission delay τ= 0.3

下载: 全尺寸图片幻灯片

图 5 当传输时滞τ = 0.2时, 利用带时间窗口的双脉冲切换控制器实现两个忆阻振荡系统的滞同步情况

Fig. 5 Lag synchronization of the two memristor oscillators via a double-impulsive switch controller with time windows and the transmission delay τ = 0.2

下载: 全尺寸图片幻灯片

图 6 当传输时滞τ= 0.3时, 利用带时间窗口的单脉冲控制器实现两个忆阻振荡系统的滞同步情况

Fig. 6 Lag synchronization of the two memristor oscillators via a single-impulsive controller with time window and the transmission delay τ = 0.3

下载: 全尺寸图片幻灯片

图 7 当传输时滞τ = 0.2时, 利用带时间窗口的单脉冲控制器实现两个忆阻振荡系统的滞同步情况

Fig. 7 Lag synchronization of the two memristor oscillators via a single-impulsive controller with time window and the transmission delay τ= 0.2

下载: 全尺寸图片幻灯片

参考文献(31)

[1]	Chua L O. Memristor-the missing circuit element. IEEE Transaction on Circuit Theory, 1971, 18(5): 507-519 doi: 10.1109/TCT.1971.1083337
[2]	Strukov D B, Snider G S, Stewartand D R, Williams R S. The missing memristor found. Nature, 2008, 453: 80-83 doi: 10.1038/nature06932
[3]	Itoh M, Chua L O. Memristor oscillators. International Journal of Bifurcation and Chaos, 2008, 18(11): 3183-3206 doi: 10.1142/S0218127408022354
[4]	Ventra M D, Pershin Y V, Chua L O. Circuit elements with memory: memristors, memcapacitors, and meminductors. Proceedings of the IEEE, 2009, 9(10): 1715-1716 http://d.old.wanfangdata.com.cn/OAPaper/oai_arXiv.org_0901.3682
[5]	Guckert L, Swartzlander E E. Optimized memristor-based multipliers. IEEE Transaction on Circuits and Systems Ⅰ, 2017, 64(2): 373-385 doi: 10.1109/TCSI.2016.2606433
[6]	Wu A, Wen S, Zeng Z. Synchronization control of a class of memristor-based recurrent neural networks. Information Science, 2012, 183(1): 106-116 doi: 10.1016/j.ins.2011.07.044
[7]	李传东, 葛均辉, 田园.脉冲神经网络的忆阻器突触联想学习电路分析.重庆大学学报, 2014, 37(7): 115-124 http://d.old.wanfangdata.com.cn/Periodical/cqdxxb201407016 Li Chuan-Dong, Ge Jun-Hui, Tian Yuan. Associative learning of memristive synapses circuits based on spiking neural networks. Journal of Chongqing University, 2014, 37(7):115-124 http://d.old.wanfangdata.com.cn/Periodical/cqdxxb201407016
[8]	Wu A, Zeng Z. Exponential stabilization of memristive neural networks with time delays. IEEE Transactions on Neural Networks & Learning Systems, 2012, 23(12): 1919-1929 http://europepmc.org/abstract/med/24808147
[9]	Wu H, Li R, Yao R, Zhang X. Weak, modifled and function projective synchronization of chaotic memristive neural networks with time delays. Neurocomputing, 2015, 149:667-676 doi: 10.1016/j.neucom.2014.08.008
[10]	Wu H, Li R, Wei H, Zhang X, Yao R. Synchronization of a class of memristive neural networks with time delays via sampled-data control. International Journal of Machine Learning and Cybernetics, 2015, 6(3): 365-373 doi: 10.1007/s13042-014-0271-z
[11]	Wu A. Hyperchaos synchronization of memristor oscillator system via combination scheme. Advances in Difierence Equations, 2014, DOI: 10.1186/1687-1847-2014-86
[12]	Wen S, Zeng Z, Huang T, Chen Y. Fuzzy modeling and synchronization of difierent memristorbased chaotic circuits. Physics Letters A, 2013, DOI: 10.1016/j.physleta.2013.05.046
[13]	Vaidyanathan S, Pham VT, Volos C. Adaptive control, synchronization and circuit simulation of a memristor-based hyperchaotic system with hidden attractors. Advances in Memristors, Memristive Devices and Systems, 2017, DOI: 10.1007/978-3-319-51724-7_5
[14]	Yang Z, Xu D. Stability analysis and design of impulsive control systems with time delay. IEEE Transactions on Automatic Control, 2007, 52(8): 1448-1454 doi: 10.1109/TAC.2007.902748
[15]	Fu X, Zhu Q. Stability of nonlinear impulsive stochastic systems with Markovian switching under generalized average dwell time condition. Science China Information Sciences, 2018, DOI: 10.1007/s11432-018-9496-6
[16]	Guan Z, Chen L, Ueta T. On impulsive control of a periodically forced chaotic pendulum system. IEEE Transactions on Automatic Control, 2000, 45(9): 1724-1727 doi: 10.1109/9.880633
[17]	Huang T, Li C, Duan S, Starzyk J A. Robust exponential stability of uncertain delayed neural networks with stochastic perturbation and impulse efiects. IEEE Transactions On Neural Networks And Learning Systems, 2012, 23(6):866-875 doi: 10.1109/TNNLS.2012.2192135
[18]	Feng Y, Zou L, Tu Z. Stability analysis for a class of nonlinear impulsive switched systems. The Journal of Nonlinear Sciences and Applications, 2017, 10: 4544-4551 doi: 10.22436/jnsa.010.08.46
[19]	Li D, Wang S, Zhang X, Yang D. Impulsive control for permanent magnet synchronous motors with uncertainties: LMI approach. Chinese Physics B, 2010, 19(1): 160-166 doi: 10.1088/1674-1056/19/1/010506
[20]	Hu C, Jiang H. Stabilization and synchronization of unifled chaotic system via impulsive control. Abstract and Applied Analysis, 2014, DOI: 10.1155/2014/369842
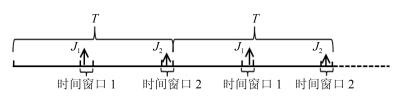
[21]	Y. Feng, C. Li, Huang T. Sandwich control systems with impulse time windows. International Journal of Machine Learning and Cybernetics, 2016, DOI: 10.1007/s13042-016-0580-5
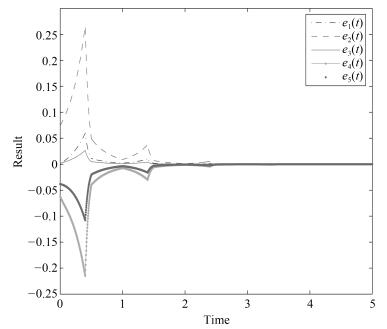
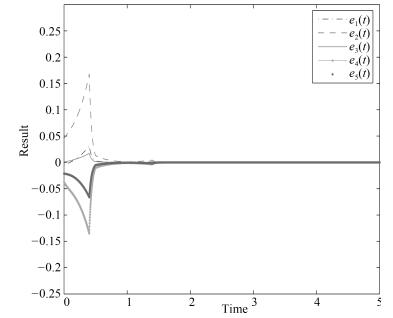
[22]	Jiang Y, Li C. Globally exponential stability of memristive neural networks with time-varying delays and synchronous switching. Acta Automatica Sinica, 2017, 43(8): 1465-1469 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zdhxb201708018
[23]	Zou L, Peng Y, Feng Y, Tu Z. Impulsive control of nonlinear systems with impulse time window and bounded gain error. Nonlinear Analysis: Modelling and Control, 2018, 23(1): 40-49 http://www.researchgate.net/publication/322400071_Impulsive_control_of_nonlinear_systems_with_impulse_time_window_and_bounded_gain_error
[24]	Zhang G, Zeng Z. Exponential stability for a class of memristive neural networks with mixed time-varying delays. Applied Mathematics & Computation, 2018, 321: 544-554 http://www.ingentaconnect.com/content/el/00963003/2018/00000321/00000001/art00033
[25]	Luo Y P, Xia W H, Liu G R, Deng F Q. LMI approach to exponential stabilization of distributed parameter control systems with delay. Acta Automatica Sinica, 2009, 35(3): 299-304 http://www.ams.org/mathscinet-getitem?mr=2535951
[26]	Liu H, Wang Z, Shen B. Stability analysis for discrete-time stochastic memristive neural networks with both leakage and probabilistic delays. Neural Networks, 2018, 102: 1-9 doi: 10.1016/j.neunet.2018.02.003
[27]	周笔锋, 罗毅平.时滞分布参数系统中和控制器设计.自动化学报, 2018, 44(12): 2222-2227 doi: 10.16383/j.aas.2018.c170084 Zhou Bi-Feng, Luo Yi-Ping. Neutralization Control of Distributed Parameter Systems With Delay. Acta Automatica Sinica, 2018, 44(12): 2222-2227 doi: 10.16383/j.aas.2018.c170084
[28]	Yang X, Ho D W C. Synchronization of delayed memristive neural networks: robust analysis approach. IEEE Transactions on Cybernetics, 2016, 46(12): 3377-3387 doi: 10.1109/TCYB.2015.2505903
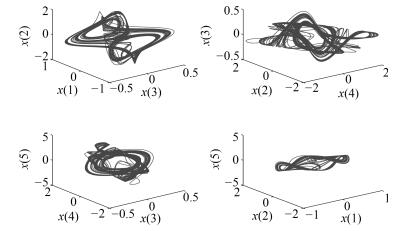
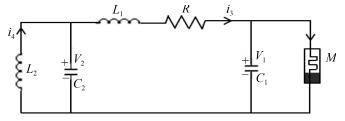
[29]	包伯成, 王其红, 许建平.基于忆阻元件的五阶混沌电路研究.电路与系统学报, 2011, 16(2): 66-70 doi: 10.3969/j.issn.1007-0249.2011.02.013 Bao Bo-Cheng, Wang Qi-Hong, Xu Jian-Ping. On memristor based flve-order chaotic circuit. Journal of Circuits and Systems, 2011, 16(2): 66-70 doi: 10.3969/j.issn.1007-0249.2011.02.013
[30]	Li Q, Liu S. Adaptive modifled function projective lag synchronization of memristor-based flve-order chaotic circuit systems. Advances in Mathematical Physics, 2017, 2017(5):1-8 http://www.researchgate.net/publication/315598475_Adaptive_Modified_Function_Projective_Lag_Synchronization_of_Memristor-Based_Five-Order_Chaotic_Circuit_Systems
[31]	Sanchez E N, Perez J P. Input-to-state stability (ISS) analysis for dynamic neural networks. IEEE Transactions on Circuits Systems-Ⅰ, 1999, 46(11): 1395-1398 doi: 10.1109/81.802844