Stability Analysis of Lattice Model Considering the Honk Effect and Driver Heterogeneity
-
摘要: 道路环境及密集交通流随机波动是交通扰动的诱因, 文中考虑道路环境中的汽车鸣笛效应和驾驶员异质性的影响, 提出鸣笛发生临界密度的概念, 建立了更符合实际的格子流体动力学模型, 并揭示非饱和交通状态下诱发交通流失稳的机理.在线性稳定性分析中利用扰动法得到了该模型的稳定性条件, 并基于还原微扰法对该模型的非线性稳定性问题进行研究, 通过求解mKDV方程获取的扭结-反扭结孤立波描述了在临界点附近密度波的传输规则.仿真结果表明, 考虑有鸣笛效应的新格子模型相比于Nagatani模型的稳定性更强, 而较大的临界密度对交通流稳定性存在消极影响; 与以往微观模型相比, 本文模型能解释鸣笛现象发生的自然条件, 即密度高且流量低的地方, 同时驾驶员特性也对交通流的稳定性存在着显著影响.Abstract: The road environment and traffic flow fluctuation are the incentive of traffic disturbance. This paper developed a new lattice model by introducing a concept of critical density by considering driver heterogeneity in the context of honk effect, and revealed the mechanism of inducing traffic flow instability. The stability condition of the lattice model is obtained by using the perturbation method in the linear stability analysis. The nonlinear stability of the proposed lattice model is studied through the reduced perturbation method, and the kink-antikink soliton solution by solving the mKDV equation describes the propagation discipline of density waves near the critical point. The simulation experiments verified that the stability of the modified lattice model is stronger than that of Nagatani model, while the large critical density has a negative effect on the stability of traffic flow. Compared to the previous microscopic models, the proposed model is able to explain the natural condition for honk occurrence, that is, high density and low flow area. In addition, the results show that the driver characteristic also has a significant impact on the stability of traffic flow.
-
Key words:
- Traffic flow /
- lattice model /
- stability analysis /
- honk effect /
- driver heterogeneity /
- critical density
1) 本文责任编委 王鼎 -
图 1 对于不同的参数$p$下的车辆的灵敏度与密度相图$(\rho, a)$
Fig. 1 The phase diagram$(\rho, a)$of the sensitivity and the density under different values of parameter $p$
图 2 在不同参数权重$p$下新模型的密度时空演化图
Fig. 2 The space-time evolution of density for the new model under different values of parameter $p$
图 3 参数$q$对交通流稳定性的影响
Fig. 3 The influence of parameter $q$ on the traffic flow stability
图 4 临界密度$\rho_{{\rm lim}_{1}}$对交通流稳定性的影响
Fig. 4 The influence of critical density $\rho_{{\rm lim}_{1}}$ on the traffic flow stability
图 5 参数$c$对交通流稳定性的影响
Fig. 5 The influence of parameter $c$ on the traffic flow stability
-
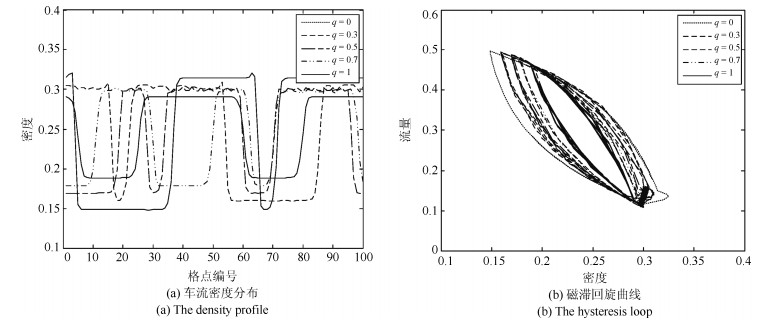
[1] Lighthill M J, Whitham G B. On kinematic waves I. Flood movement in long rivers. Proceedings of the Royal Society of London A Mathematical Physical and Engineering Sciences, 1955, 229(1178): 281-316 doi: 10.1098/rspa.1955.0088 [2] Lighthill M J, Whitham G B. On kinematic waves Ⅱ. A therory of traffic flow on long crowded roads. Proceedings of the Royal Society A-Mathematical Physical and Engineering Sciences, 1955, 229(1178): 317-345 [3] Richards P I. Shock waves on highway. Operations Research, 1956, 4(1): 45-51 http://www.researchgate.net/publication/224010978_Shock_waves_on_a_highway [4] 吴正.高速交通中堵塞形成阶段的交通流模型.交通运输工程学报, 2003, 3(2): 61-64 doi: 10.3321/j.issn:1671-1637.2003.02.014Wu Zheng. Traffic flow modelling for jam developing procedure on expressway. Journal of Traffic and Transportation Engineering, 2003, 3(2): 61-64 doi: 10.3321/j.issn:1671-1637.2003.02.014 [5] 姜锐, 吴清松.一种新的交通流动力学模型.科学通报, 2000, 45(17): 1895-1899 doi: 10.3321/j.issn:0023-074X.2000.17.019Jiang Rui, Wu Qing-Song. A new traffic flow dynamics model. Chinese Science Bulletin, 2000, 45(17): 1895-1899 doi: 10.3321/j.issn:0023-074X.2000.17.019 [6] 夏冬雪, 李春贵.基于邻近车道车辆和多前车信息的双车道交通流动力学模型.系统工程理论与实践, 2012, 32(7): 1562-1567 doi: 10.3969/j.issn.1000-6788.2012.07.022Xia Dong-Xue, Li Chun-Gui. Hydrodynamic model for two lane traffic flow considering the information of multi-vehicles ahead and lateral vehicles. Systems Engineering - Theory and Practice, 2012, 32(7): 1562-1567 doi: 10.3969/j.issn.1000-6788.2012.07.022 [7] Guo J, Jin H Z, Chen X L, Wu D J. An improved one-dimensional cellular automation model of traffic flow. In: Proceedings of the 2008 Chinese Control and Decision Conference. Yantai, China: IEEE, 2008. 4481-4485 [8] Zhang Z, Ming C, Wang L. Research of longitudinal safety separation based on nagel-schreckenberg traffic flow model. Physics Procedia, 2012, 33(1): 573-579 http://www.sciencedirect.com/science/article/pii/S1875389212014198 [9] 张杰, 贺国光.基于一维元胞自动机模型的交通流混沌研究.武汉理工大学学报, 2009, 33(1): 33-36 doi: 10.3963/j.issn.1006-2823.2009.01.009Zhang Jie, He Guo-Guang. Study on chaos in traffic flow based on the one-dimensional cellular automation model. Journal of Wuhan University of Technology, 2009, 33(1): 33-36 doi: 10.3963/j.issn.1006-2823.2009.01.009 [10] 李永福, 孙棣华, 崔明月.全速度差跟驰模型的Lyapunov稳定性分析.控制理论与应用, 2010, 27(12): 1669-1673 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=kzllyyy201012013Li Yong-Fu, Sun Di-Hua, Cui Ming-Yue. Lyapunov stability analysis for the full velocity difference car following model. Control Theory and Application, 2010, 27(12): 1669-1673 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=kzllyyy201012013 [11] 吴洋, 罗霞, 刘昱岗.跟驰驾驶行为的滑模控制系统建模.控制理论与应用, 2008, 25(4): 671-676 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=kzllyyy200804015Wu Yang, Luo Xia, Liu Yu-Gang. Sliding mode control system modeling of car following driving behavior. Control Theory and Application, 2008, 25(4): 671-676 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=kzllyyy200804015 [12] Ngoduy D. Linear stability of a generalized multi-anticipative car following model with time delays. Communications in Nonlinear Science and Numerical Simulation, 2015, 22(1-3): 420-426 doi: 10.1016/j.cnsns.2014.08.019 [13] 翟聪, 刘伟铭, 谭飞刚.一类耦合映射模糊时滞跟驰系统的反馈控制.华南理工大学学报, 2017, 45(1): 9-17 doi: 10.3969/j.issn.1000-565X.2017.01.002Zhai Cong, Liu Wei-Ming, Tan Fei-Gang. Feedback control of a class of coupled map fuzzy time delay car following system. Journal of South China University of Technology, 2017, 45(1): 9-17 doi: 10.3969/j.issn.1000-565X.2017.01.002 [14] Nagatani T. TDGL and MKdV equations for jamming transition in the lattice models of traffic. Physica A Statistical Mechanics and Its Applications, 1999, 264(3): 581-592 [15] Peng G, Liu C, Tuo M. Influence of the traffic interruption probability on traffic stability in lattice model for two-lane freeway. Physica A Statistical Mechanics and Its Applications, 2015, 436(1): 952-959 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=24c49031af8404f8bde6e7d27bbb73f3 [16] Tian J F, Yuan Z Z, Jia B. The stabilization effect of the density difference in the modified lattice hydrodynamic model of traffic flow. Physica A Statistical Mechanics and Its Applications, 2012, 391(19): 4476-4482 doi: 10.1016/j.physa.2012.04.027 [17] Ge H X, Cheng R J. The "backward looking" effect in the lattice hydrodynamic model. Physica A Statistical Mechanics and Its Applications, 2008, 387(387): 6952-6958 http://www.sciencedirect.com/science/article/pii/S0378437108005220 [18] Li Y, Zhang L, Zheng T, et al. Lattice hydrodynamic model based delay feedback control of vehicular traffic flow considering the effects of density change rate difference. Communications in Nonlinear Science and Numerical Simulation, 2015, 29(1-3): 224-232 doi: 10.1016/j.cnsns.2015.05.008 [19] Wang Y N, Cheng R J, Ge H X. A lattice hydrodynamic model based on delayed feedback control considering the effect of flow rate difference. Physica A Statistical Mechanics and Its Applications, 2017, 479(1): 478-484 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=68836b876df3fbc0803eb2d334c8af7e [20] Tian Y F, Jia B, Li X G. Flow difference effect in the lattice hydrodynamic model. Chinese Physics B, 2010, 19(4): 31-36 [21] Tang T Q, Li C Y, Wu Y H. Impact of the honk effect on the stability of traffic flow. Physica A Statistical Mechanics and Its Applications, 2011, 390(20): 3362-3368 doi: 10.1016/j.physa.2011.05.010 [22] Wen H, Rong Y, Zeng C. The effect of driver$'$s characteristics on the stability of traffic flow under honk environment. Nonlinear Dynamics, 2016, 84(3): 1517-1528 doi: 10.1007/s11071-015-2585-0 [23] Kuang H, Xu Z P, Li X L. An extended car-following model accounting for the honk effect and numerical tests. Nonlinear Dynamics, 2017, 87(1): 149-157 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=524e59944316d22f807da5fdf33fbeaf [24] Zheng L, Ma S, Zhong S. Analysis of honk effect on the traffic flow in a cellular automaton model. Physica A Statistical Mechanics and Its Applications, 2011, 390(6): 1072-1084 doi: 10.1016/j.physa.2010.11.014 [25] Jia B, Jiang R, Wu Q S. Honk effect in the two-lane cellular automaton model for traffic flow. Physica A Statistical Mechanics and Its Applications, 2005, 348(3): 544-552 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=82d9dadecd9789442049c825793ac1c6 [26] Zhai C, Wu W T. An extended multi-phase lattice model with consideration of optimal current changes with memory. Cluster Computing, 2019, (22): S7447-S7457 doi: 10.1007/s10586-018-1773-3 [27] Zhai C, Wu W T. Stability analysis of two-lane lattice hydrodynamic model considering lane-changing and memorial effects. Modern Physics Letters B, 2018, 32(20): 1-16 http://www.researchgate.net/publication/325968639_Stability_analysis_of_two-lane_lattice_hydrodynamic_model_considering_lane-changing_and_memorial_effects [28] 潘晓东.人体信息技术在道路交通环境与安全性评价中的应用.中国公路学报, 2007, 14(1): 109-111 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgglxb2001z1027Pan Xiao-Dong. The application of body information technology on road and traffic enviroment and safety evalution. China Journal of Highway and Transport, 2007, 14(1): 109-111 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgglxb2001z1027 [29] Golias I, Karlaftis M G. An international comparative study of self-reported driver behavior. Transportation Research Part F Psychology and Behaviour, 2001, 4(4): 243-256 doi: 10.1016/S1369-8478(01)00026-2 [30] Tricot N, Sonnerat D, Popieul J C. Driving styles and traffic density diagnosis in simulated driving conditions. Intelligent Vehicle Symposium, 2002, 2(1): 298-303 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=CC027197004 [31] Sharma S. Lattice hydrodynamic modeling of two-lane traffic flow with timid and aggressive driving behavior. Physica A Statistical Mechanics and Its Applications, 2015, 421(1): 401-411 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=be39c249063c878bfec525463eac2079 [32] Peng G, Li Q. A new macro model of traffic flow by incorporating both timid and aggressive driving behaviors. Modern Physics Letters B, 2016, 30(29): 5429-5431 http://smartsearch.nstl.gov.cn/paper_detail.html?id=fa2559ae169c9c2dbfe33757c8bc49ef [33] Zhai C, Wu W T. A new car-following model considering driver$'$s characteristics and traffic jerk. Nonlinear Dynamics, 2018, 93(4): 2185-2199 doi: 10.1007/s11071-018-4318-7 -
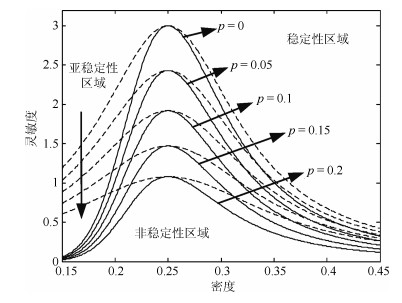
下载:
图(6)
计量
- 文章访问数: 873
- HTML全文浏览量: 173
- PDF下载量: 94
- 被引次数: 0
