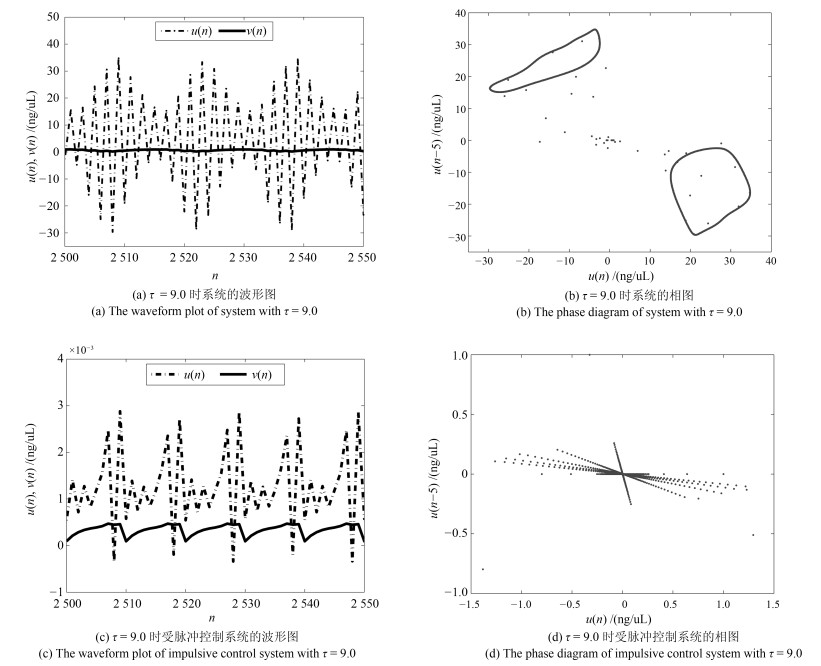
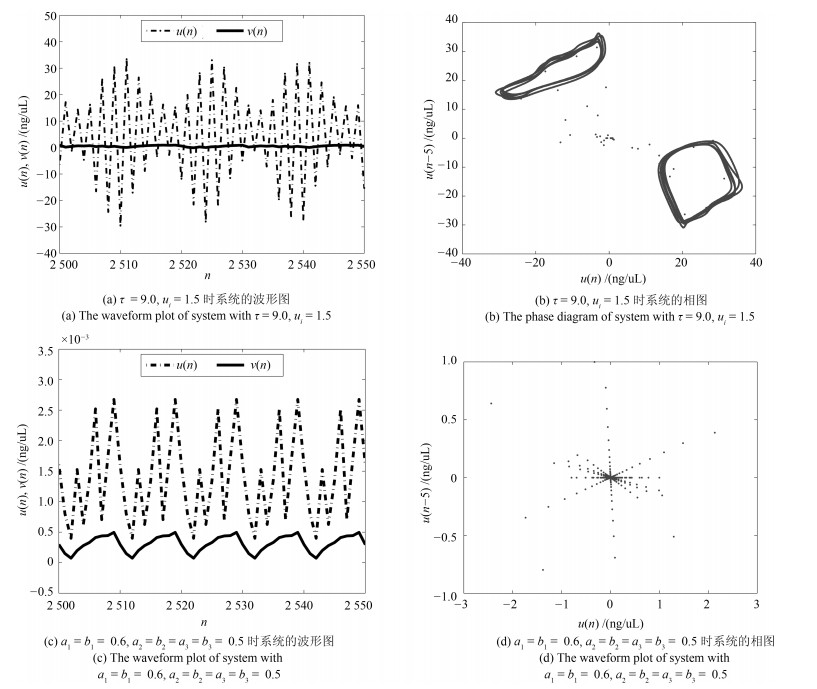
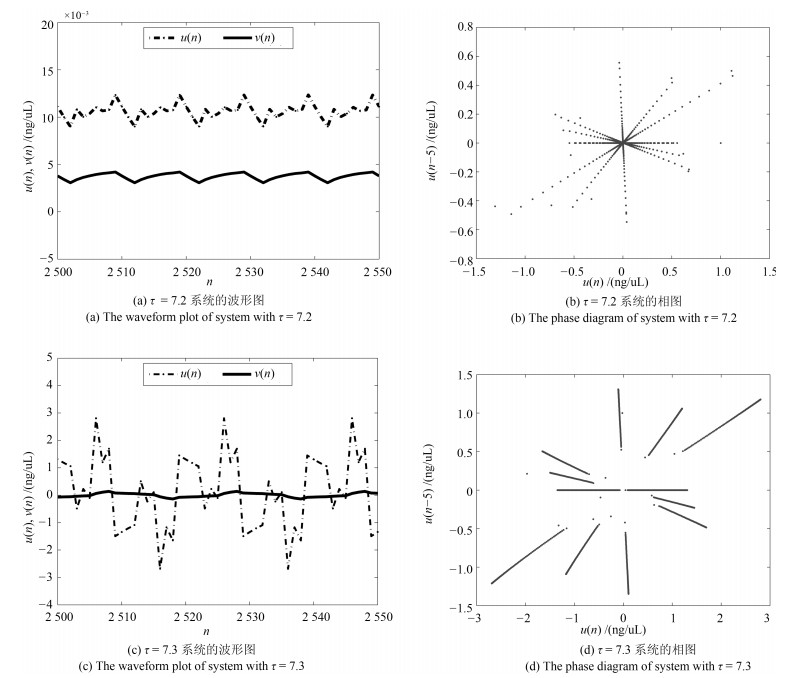
|
[1]
|
Lakshmikantham V, Bainov D D, Simeonov P S. Theory of Impulsive Differential Equations. Singapore: World Scientific, 1989.
|
|
[2]
|
Yang X S, Lam J, Ho D W C, Feng Z G. Fixed-time synchronization of complex networks with impulsive effects via nonchattering control. IEEE Transactions on Automatic Control, 2017, 62(11): 5511-5521 doi: 10.1109/TAC.2017.2691303
|
|
[3]
|
Rakkiyappan R, Velmurugan G, George J N, Selvamani R. Exponential synchronization of Lur'e complex dynamical networks with uncertain inner coupling and pinning impulsive control. Applied Mathematics and Computation, 2017, 307: 217-231 doi: 10.1016/j.amc.2017.02.041
|
|
[4]
|
Zhu Q X, Song B. Exponential stability of impulsive nonlinear stochastic differential equations with mixed delays. Nonlinear Analysis: Real World Applications, 2011, 12(5): 2851-2860 doi: 10.1016/j.nonrwa.2011.04.011
|
|
[5]
|
Long S J, Xu D Y. Global exponential stability of non-autonomous cellular neural networks with impulses and time-varying delays. Communications in Nonlinear Science and Numerical Simulation, 2013, 18(6): 1463-1472 doi: 10.1016/j.cnsns.2012.10.015
|
|
[6]
|
苏晓明, 张品, 祝君宇.广义时变脉冲系统的时域稳定.自动化学报, 2016, 42(2): 309-314 doi: 10.16383/j.aas.2016.c150284Su Xiao-Ming, Zhang Pin, Zhu Jun-Yu. Finite-time stability of linear time-varying descriptor impulse systems. Acta Automatica Sinica, 2016, 42(2): 309-314 doi: 10.16383/j.aas.2016.c150284
|
|
[7]
|
Yang T. Impulsive Control Theory. Berlin, Heidelberg: Springer-Verlag, 2001.
|
|
[8]
|
Zhang L, Yang X S, Xu C, Feng J W. Exponential synchronization of complex-valued complex networks with time-varying delays and stochastic perturbations via time-delayed impulsive control. Applied Mathematics and Computation, 2017, 306: 22-30 doi: 10.1016/j.amc.2017.02.004
|
|
[9]
|
Ai Z D, Chen C C. Asymptotic stability analysis and design of nonlinear impulsive control systems. Nonlinear Analysis: Hybrid Systems, 2017, 24: 244-252 doi: 10.1016/j.nahs.2016.10.003
|
|
[10]
|
Jiang X W, Ding L, Guan Z H, Yuan F S. Bifurcation and chaotic behavior of a discrete-time Ricardo-Malthus model. Nonlinear Dynamics, 2013, 71(3): 437-446 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=153d7e803be74d253e30d2512aba68d0
|
|
[11]
|
Yue D D, Guan Z H, Chen J, Ling G, Wu Y H. Bifurcations and chaos of a discrete-time model in genetic regulatory networks. Nonlinear Dynamics, 2017, 87(1): 567-586 doi: 10.1007/s11071-016-3061-1
|
|
[12]
|
Cao J Z, Jiang H J. Hopf bifurcation analysis for a model of single genetic negative feedback autoregulatory system with delay. Neurocomputing, 2013, 99: 381-389 doi: 10.1016/j.neucom.2012.07.021
|
|
[13]
|
Ouannas A, Odibat Z, Shawagfeh N, Alsaedi A, Ahmad B. Universal chaos synchronization control laws for general quadratic discrete systems. Applied Mathematical Modelling, 2017, 45: 636-641 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=7d376a18122845cc7edb70f1ef7a9473
|
|
[14]
|
Salman S M, Yousef A M, Elsadany A A. Stability, bifurcation analysis and chaos control of a discrete predator-prey system with square root functional response. Chaos, Solitons and Fractals, 2016, 93: 20-31 doi: 10.1016/j.chaos.2016.09.020
|
|
[15]
|
Din Q. Global stability and Neimark-Sacker bifurcation of a host-parasitoid model. International Journal of Systems Science, 2017, 48(6): 1194-1202 doi: 10.1080/00207721.2016.1244308
|
|
[16]
|
刘峰.基于脉冲控制的分叉与混沌系统研究[博士学位论文], 华中科技大学, 中国, 2008Liu Feng. Study on Bifurcation and Chaos System with Impulsive Control [Ph.D. dissertation], Huazhong University of Science and Technology, China, 2008
|
|
[17]
|
Zhang Y, Sun J T, Feng G. Impulsive control of discrete systems with time delay. IEEE Transactions on Automatic Control, 2009, 54(4): 830-834 http://d.old.wanfangdata.com.cn/Periodical/xtkxysx201801009
|
|
[18]
|
Wu S C, Li C D, Liao X F, Duan S K. Exponential stability of impulsive discrete systems with time delay and applications in stochastic neural networks: a Razumikhin approach. Neurocomputing, 2012, 82: 29-36 doi: 10.1016/j.neucom.2011.09.029
|
|
[19]
|
Liu F, Guan Z H, Wang H O, Li Y Q. Impulsive control of bifurcations. Mathematics and Computers in Simulation, 2009, 79(7): 2180-2191 doi: 10.1016/j.matcom.2008.12.003
|
|
[20]
|
Zheng Y A, Nian Y B, Liu Z R. Impulsive control for the stabilization of discrete chaotic system. Chinese Physics Letters, 2002, 19(9): 1251-1253 doi: 10.1088/0256-307X/19/9/310
|
|
[21]
|
Liu F, Yin X, Zhang Z, Sun F L. Stability and neimark-sacker bifurcation analysis in a genetic network with delay. Journal of Advanced Computational Intelligence and Intelligent Informatics, 2017, 21(2): 278-283 doi: 10.20965/jaciii.2017.p0278
|




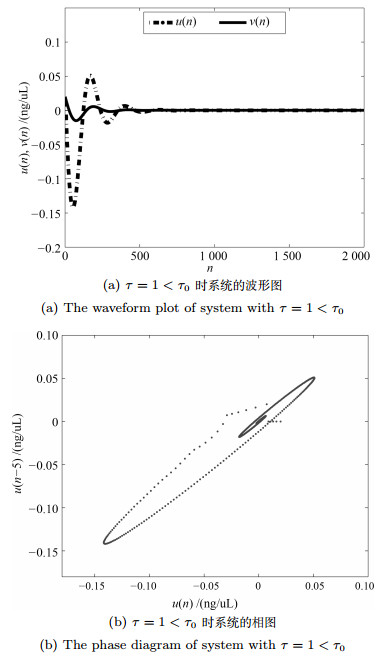
 下载:
下载: