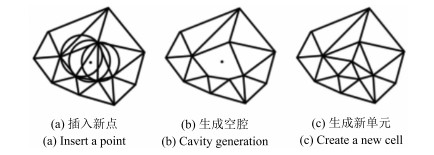
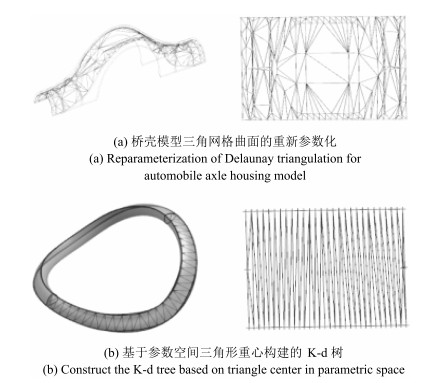
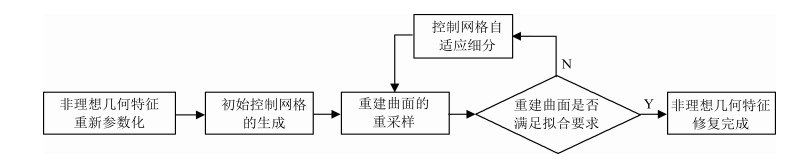
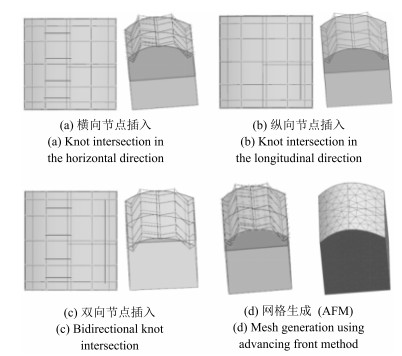
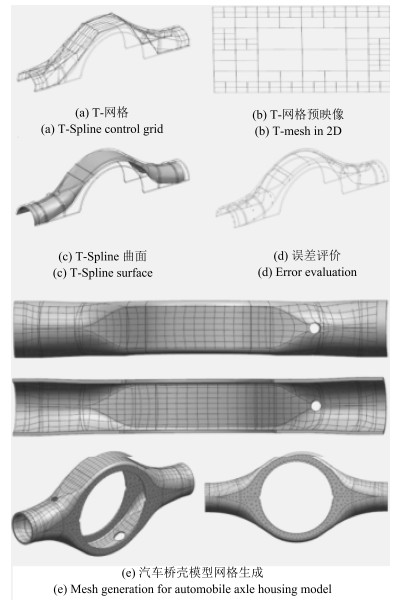
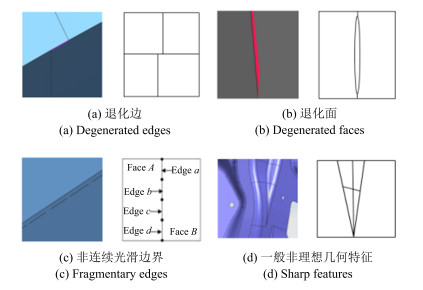
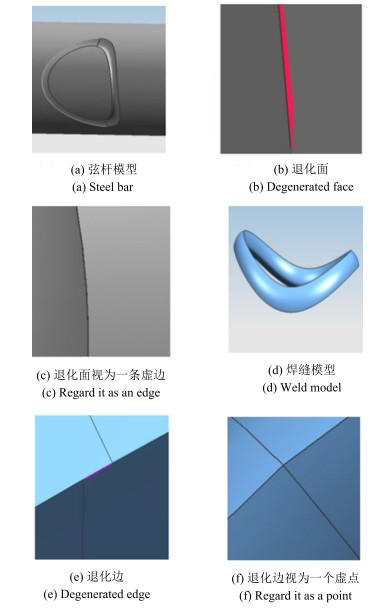
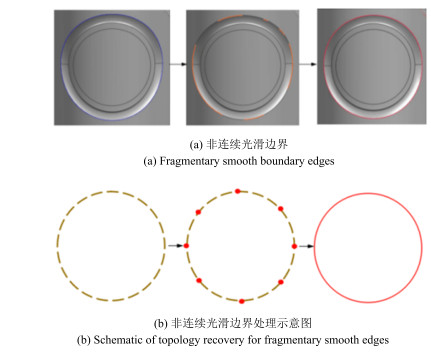
-
摘要: 从高质量曲面网格生成的需求出发，提出了一种基于T-Spline的全自动几何拓扑修复方法.本文方法创新性主要可归纳为：1）对原有计算机辅助设计（Computer aided design，CAD）几何模型不进行任何修改保留其本真，自动识别CAD几何模型中常见不必要的几何特征，成功解决了CAD几何模型中存在的几何瑕疵，如短边、窄面、退化边、退化面、非连续光滑边界及尖锐特征等，利用新生成的"虚边"、"虚面"处理几何瑕疵，同时通过虚拓扑重构CAD几何模型的B-Rep；2）开发了一套CAD/CAE集成系统，统一了几何模型与计算分析模型，实现计算机辅助工程（Computer aided engineering，CAE）与CAD两者的无缝集成，所有拓扑修复操作及后续CAE分析计算均在同一环境下进行，避免了几何模型在CAE与CAD系统间进行转换时造成的数据丢失.该方法能够对复杂实体实现全自动几何拓扑修复及网格生成，实验表明，在保证不失真的前提下，修复后的几何模型能够生成质量良好的网格且能降低网格的生成规模，验证了本文方法的实用性和有效性，以满足工程实际分析的需要.Abstract: An automatic topology recovery method using T-Spline is presented to reconstruct surfaces by virtual operations for handling unwanted geometric features and facilitating mesh generation without modifying the original input computer aided design (CAD) model. Innovations of the method primarily are in two aspects. Firstly, it presents an automatic topology recovery method using T-Spline to identificate and handle unwanted geometric features in solid modeling automatically, such as short edges, small faces, degenerated edges, degenerated faces, fragmentary boundary edges, sharp features, etc. And a valid B-Rep of CAD model is reconstructed using virtual topology. Secondly, in order to make a truly seamless interaction between CAD and computer aided engineering (CAE), a system for CAE analysis is developed to improve the efficiency and accuracy of the simulation. All operations and CAE analysis can be set up directly on the CAD model, thus automatic simulation is possible and geometric simplification is avoided. In CAE analysis, on account of the requirement for automatic simulation of entire process, the method based on T-spline can relieve the burden of mesh generation and promote CAE analysis to some extent.
-
Key words:
- T-Spline /
- automatic topology recovery /
- virtual topology /
- curve and surface fitting /
- mesh generation
1) 本文责任编委 李成栋 -
图 1 美国 Sandia 国家实验室数值模拟过程用时数据统计
Fig. 1 Statistics related to numerical computation from Sandia National Laboratories of USA
图 2 完整实体CAE分析软件前处理模块框架
Fig. 2 Preprocessing module of center for complete solid analysis software for engineering structures
图 3 完整实体CAE分析软件整体框架
Fig. 3 The software framework of center for complete solid analysis software for engineering structures
图 4 完整实体CAE分析软件界面
Fig. 4 The software interface of center for complete solid analysis software for engineering structures
图 12 基于相邻面片间公共边界处的光滑度和几何中心处的法矢夹角判断准则示意图
Fig. 12 The judgment criteria for seeded region growing algorithm images
图 14 Delaunay三角剖分的重要特性
Fig. 14 The most important characteristic of Delaunay triangulation
图 18 验证改进目标单元快速搜索算法效率算例
Fig. 18 An example to verify the e–ciency of target cell searching algorithm
图 21 自适应T-Spline曲面重建流程
Fig. 21 The flowchart of automatic T-Spline surface reconstruction algorithm
图 22 自适应T-Spline曲面重建实例
Fig. 22 An example of automatic T-Spline surface reconstruction algorithm
图 24 任意自由边界或含空洞的非理想几何特征
Fig. 24 Nonideal geometric features with complex boundary or holes
图 25 任意自由边界的非理想几何特征自动修复
Fig. 25 Topology recovery for nonideal geometric features with arbitrary boundary
图 26 三角形及四边形网格的质量因子
Fig. 26 The quality factor of triangular and quadrilateral mesh generation
图 27 汽车桥壳模型全自动几何拓扑修复
Fig. 27 The results of automobile axle housing model after automatic topology recovery
图 28 钢架焊缝模型全自动几何拓扑修复
Fig. 28 The results of steel frame model after automatic topology recovery
表 1 本文改进快速搜索方法与传统搜索方法数据对比
Table 1 Comparison between traditional method and our method
方法 随机点数量 搜索耗时(s) 传统搜索方法 628 611 96.28 本文改进方法 628 611 4.69 
下载: 导出CSV
表 2 自适应T-Spline拟合曲面误差及网格质量评价
Table 2 Relevant experimental data of T-Spline fltting for automatic surface reconstruction
算例 $E_{ m}$ ($10^{-5}$ mm) RMSE ($10^{-5}$ mm) $a_{\min}$ $a_{\rm aver}$ 拟合曲面 1.28 0.96 0.862 0.978
下载: 导出CSV
表 3 T-Spline局部节点插入算法拟合曲面误差及网格质量评价
Table 3 Relevant experimental data of T-Spline fltting by the local reflnement algorithm
局部节点插入 $E_{m}$($10^{-5}$ mm) RMSE($10^{-5}$ mm) $a_{\rm min}$ $a_{\rm aver}$ 横向节点插入 3.32 1.63 0.875 0.937 纵向节点插入 2.87 1.39 0.913 0.961 双向节点插入 2.71 1.15 0.936 0.975
下载: 导出CSV
表 4 任意自由边界非理想几何特征的拟合曲面误差及网格质量评价
Table 4 Relevant experimental data of T-Spline fltting for nonideal geometric features with arbitrary boundary
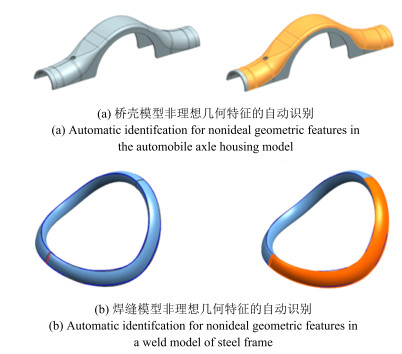
算例 $E_{m}$($10^{-5}$ mm) RMSE($10^{-5}$ mm) $a_{\rm min}$ $a_{\rm aver}$ 拟合曲面 2.93 1.26 0.837 0.935
下载: 导出CSV
表 5 汽车桥壳模型拟合曲面误差及网格质量评价
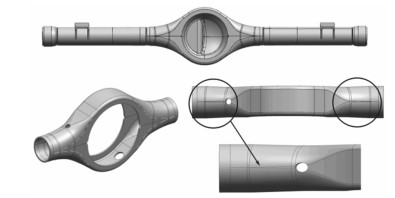
Table 5 Relevant experimental data of T-Spline fltting for automobile axle housing model
算例 $E_{m}$($10^{-3}$ mm) RMSE($10^{-3}$ mm) $a_{\min}$ $a_{\rm aver}$ 汽车桥壳模型 7.96 2.57 0.672 0.916
下载: 导出CSV
表 6 汽车桥壳模型拓扑修复前后数据对比
Table 6 Relevant experimental data of automatic topology recovery for automobile axle housing model
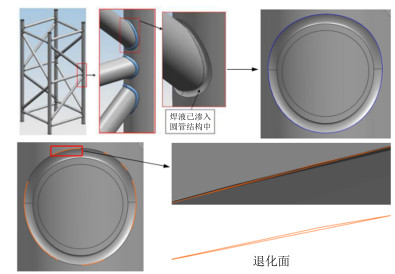
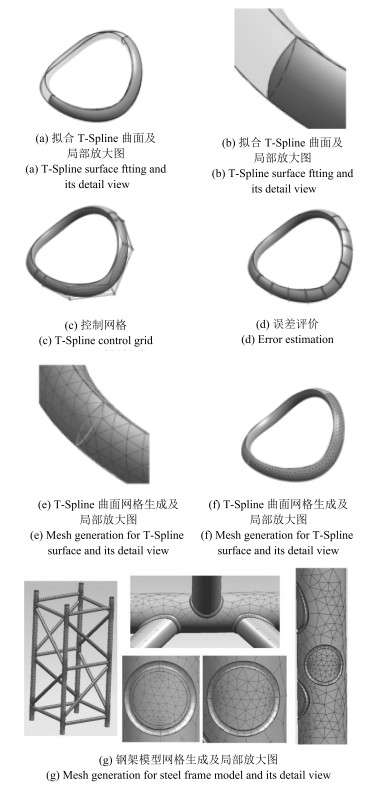
汽车桥壳模型 曲面数量 曲线数量 顶点数量 修复前 241 969 724 修复后 92 392 276
下载: 导出CSV
表 7 钢架焊缝模型拟合曲面误差及网格质量评价
Table 7 Relevant experimental data of T-Spline fltting for steel frame model
算例 $E_{m}$($10^{-4}$ mm) RMSE($10^{-4}$ mm) $a_{\rm min}$ $a_{\rm aver}$ 汽车桥壳模型 3.79 1.82 0.885 0.965
下载: 导出CSV
表 8 钢架模型拓扑修复前后数据对比
Table 8 Relevant experimental data of automatic topology recovery method for steel frame model
汽车桥壳模型 曲面数量 曲线数量 顶点数量 修复前 393 1547 1326 修复后 357 952 769
下载: 导出CSV
-
[1] Lancaster P, Šalkauskas K. Curve and Surface Fitting:An Introduction. London:Academic Press, 1986. [2] Piegl L, Tiller W. The NURBS Book (2nd edition). New York:Springer, 1997. [3] Butlin G, Stops C. CAD data repair. In:Proceedings of the 5th International Meshing Roundtable. Pittsburgh, PA, USA, 1996.7-12 [4] Uva A E, Monno G, Hamann B. A new method for the repair of CAD data with discontinuities. In:Proceedings of the 1998 Italian-Spanish Seminar on Design and Feasibility of Industrial Products. Vico Equense, Italy, 1998.59-73 [5] Keller J B. Removing small features from computational domains. Journal of Computational Physics, 1994, 113(1):148-150 doi: 10.1006/jcph.1994.1124 [6] Morvan S M, Fadel G M. IVECS:an interactive correction of STL files in a virtual environment. In:Proceedings of the 1996 Solid Freeform Fabrication Symposium. Austin, Texas, USA, 1996. [7] Attene M, Campen M, Kobbelt L. Polygon mesh repairing:an application perspective. ACM Computing Surveys (CSUR), 2013, 45(2):Article No. 15 http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0230137292/ [8] Patel P S, Marcum D L. Robust and efficient CAD topology generation using adaptive tolerances. International Journal for Numerical Methods in Engineering, 2008, 75(3):355-378 doi: 10.1002/nme.2263 [9] Zhang J M, Qin X Y, Han X, Li G Y. A boundary face method for potential problems in three dimensions. International Journal for Numerical Methods in Engineering, 2009, 80(3):320-337 doi: 10.1002/nme.2633 [10] Center for Complete Solid Analysis Software for Engineering Structures[Online], available:http://www.5acae.com, January 30, 2018 [11] Sederberg T W, Zheng J M, Bakenov A, Nasri A. T-splines and T-NURCCs. ACM Transactions on Graphics (TOG), 2003, 22(3):477-484 doi: 10.1145/882262.882295 [12] Mehnert A, Jackway P. An improved seeded region growing algorithm. Pattern Recognition Letters, 1997, 18(10):1065 -1071 doi: 10.1016/S0167-8655(97)00131-1 [13] Lee D T, Schachter B J. Two algorithms for constructing a Delaunay triangulation. International Journal of Computer and Information Sciences, 1980, 9(3):219-242 doi: 10.1007/BF00977785 [14] Bentley J L. Multidimensional binary search trees used for associative searching. Communications of the ACM, 1975, 18(9):509-517 doi: 10.1145/361002.361007 [15] Lévy B, Petitjean S, Ray N, Maillot J. Least squares conformal maps for automatic texture atlas generation. ACM Transactions on Graphics (TOG), 2002, 21(3):362-371 http://www.researchgate.net/publication/220720636_Least_squares_conformal_maps_for_automatic_texture_atlas_generation [16] Li W C, Ray N, Lévy B. Automatic and interactive mesh to T-spline conversion. In:Proceedings of the 4th Eurographics Symposium on Geometry Processing-SGP. The Eurographics Association, 2006. [17] Sederberg T W, Cardon D L, Finnigan G T, North N S, Zheng J M, Lyche T. T-spline simplification and local refinement. ACM Transactions on Graphics (TOG), 2004, 23(3):276-283 doi: 10.1145/1015706.1015715 [18] Wang B Y. A local meshless method based on moving least squares and local radial basis functions. Engineering Analysis with Boundary Elements, 2015, 50:395-401 doi: 10.1016/j.enganabound.2014.10.001 [19] Lee C K, Lo S H. A new scheme for the generation of a graded quadrilateral mesh. Computers and Structures, 1994, 52(5):847-857 doi: 10.1016/0045-7949(94)90070-1 [20] Park C, Noh J S, Jang I S, Kang J M. A new automated scheme of quadrilateral mesh generation for randomly distributed line constraints. Computer-Aided Design, 2007, 39(4):258-267 doi: 10.1016/j.cad.2006.12.002 [21] 刘芬, 周华民, 李德群. STL错误的手工修复方法研究.计算机工程与应用, 2006, 42(11):91-93 doi: 10.3321/j.issn:1002-8331.2006.11.029Liu Fen, Zhou Hua-Min, Li De-Qun. Research on manual repair methods of STL file errors. Computer Engineering and Applications, 2006, 42(11):91-93 doi: 10.3321/j.issn:1002-8331.2006.11.029 [22] 赵吉宾, 刘伟军, 王越超. STL文件的错误检测与修复算法研究.计算机应用, 2003, 23(2):32-33, 36 doi: 10.3969/j.issn.1001-3695.2003.02.011Zhao Ji-Bin, Liu Wei-Jun, Wang Yue-Chao. Research on algorithm for diagnosis and modification of STL file errors. Computer Applications, 2003, 23(2):32-33, 36 doi: 10.3969/j.issn.1001-3695.2003.02.011 [23] 张必强, 邢渊, 阮雪榆.面向网格简化的STL拓扑信息快速重建算法.上海交通大学学报, 2004, 38(1):39-42 doi: 10.3321/j.issn:1006-2467.2004.01.010Zhang Bi-Qiang, Xing Yuan, Ruan Xue-Yu. Fast generation of the topological information in STL for mesh simplification. Journal of Shanghai Jiaotong University, 2004, 38(1):39-42 doi: 10.3321/j.issn:1006-2467.2004.01.010 [24] 曹秉万, 陈建军, 郑耀, 黄争舸, 郑建靖.面向混合曲面模型的自动拓扑生成算法.浙江大学学报(工学版), 2014, 48(5):923-933 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zjdxxb-gx201405025Cao Bing-Wan, Chen Jian-Jun, Zheng Yao, Huang Zheng-Ge, Zheng Jian-Jing. Automatic topology generation algorithm for hybrid surface models. Journal of Zhejiang University (Engineering Science), 2014, 48(5):923-933 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zjdxxb-gx201405025 -
计量
- 文章访问数: 1539
- HTML全文浏览量: 252
- PDF下载量: 76
- 被引次数: 0
