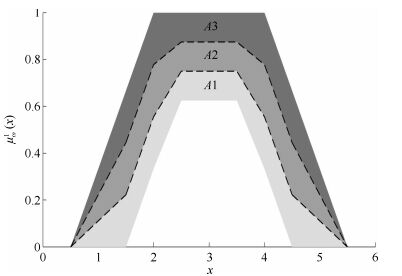
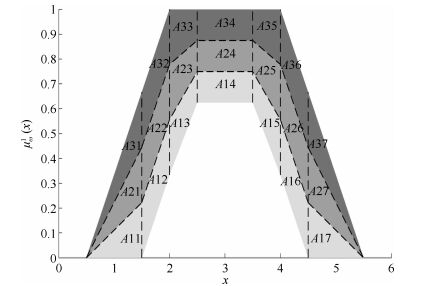
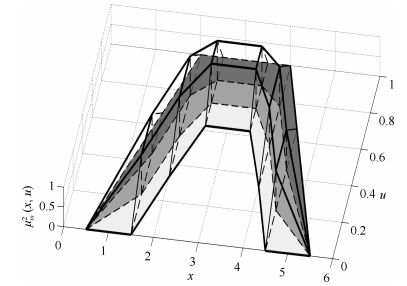
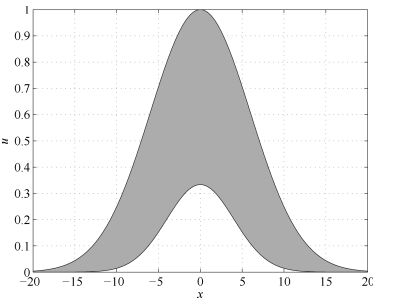
|
[1]
|
Zadeh L A. The concept of a linguistic variable and its application to approximate reasoning-Ⅰ. Information Sciences, 1975, 8(3):199-249 doi: 10.1016/0020-0255(75)90036-5
|
|
[2]
|
Aliev R A, Pedrycz W, Guirimov B G, Aliev R R, Ilhan U, Babagil M, Mammadli S. Type-2 fuzzy neural networks with fuzzy clustering and differential evolution optimization. Information Sciences, 2011, 181(9):1591-1608 doi: 10.1016/j.ins.2010.12.014
|
|
[3]
|
Pedrycz W. Granular computing:Analysis and Design of Intelligent Systems. Boca Raton, FL:CRC Press, 2013.
|
|
[4]
|
潘永平, 黄道平, 孙宗海. Ⅱ型模糊控制综述.控制理论与应用, 2011, 28(1):13-23 http://www.cnki.com.cn/Article/CJFDTOTAL-KZLY201101003.htmPan Yong-Ping, Huang Dao-Ping, Sun Zong-Hai. Overview of type-2 fuzzy logic control. Control Theory & Applications, 2011, 28(1):13-23 http://www.cnki.com.cn/Article/CJFDTOTAL-KZLY201101003.htm
|
|
[5]
|
Aisbett J, Rickard J T, Morgenthaler D. Multivariate modeling and type-2 fuzzy sets. Fuzzy sets and Systems, 2011, 163(1):78-95 doi: 10.1016/j.fss.2010.10.001
|
|
[6]
|
Uncu O, Turksen I B. Discrete interval type 2 fuzzy system models using uncertainty in learning parameters. IEEE Transactions on Fuzzy Systems, 2007, 15(1):90-106 doi: 10.1109/TFUZZ.2006.889765
|
|
[7]
|
Mendel J M, Liu X W. Simplified interval type-2 fuzzy logic systems. IEEE Transactions on Fuzzy Systems, 2013, 21(6):1056-1069 doi: 10.1109/TFUZZ.2013.2241771
|
|
[8]
|
Mendel J M, Rajati M R. On computing normalized interval type-2 fuzzy sets. IEEE Transactions on Fuzzy Systems, 2014, 22(5):1335-1340 doi: 10.1109/TFUZZ.2013.2280133
|
|
[9]
|
Wu D R, Mendel J M. Aggregation using the linguistic weighted average and interval type-2 fuzzy sets. IEEE Transactions on Fuzzy Systems, 2007, 15(6):1145-1161 doi: 10.1109/TFUZZ.2007.896325
|
|
[10]
|
Hagras H. Type-2 FLCs:a new generation of fuzzy controllers. IEEE Computational Intelligence Magazine, 2007, 2(1):30-43 doi: 10.1109/MCI.2007.357192
|
|
[11]
|
Mendel J M, Wu H W. New results about the centroid of an interval type-2 fuzzy set, including the centroid of a fuzzy granule. Information Sciences, 2007, 177(2):360-377 doi: 10.1016/j.ins.2006.03.003
|
|
[12]
|
Mendel J M, John R I, Liu F L. Interval type-2 fuzzy logic systems made simple. IEEE Transactions on Fuzzy Systems, 2006, 14(6):808-821 doi: 10.1109/TFUZZ.2006.879986
|
|
[13]
|
Mendel J M. On a 50% savings in the computation of the centroid of a symmetrical interval type-2 fuzzy set. Information Sciences, 2005, 172(3-4):417-430 doi: 10.1016/j.ins.2004.04.006
|
|
[14]
|
Hao M S, Mendel J M. Similarity measures for general type-2 fuzzy sets based on the α-plane representation. Information Sciences, 2014, 277:197-215 doi: 10.1016/j.ins.2014.01.050
|
|
[15]
|
Juang C F, Huang R B, Lin Y Y. A recurrent self-evolving interval type-2 fuzzy neural network for dynamic system processing. IEEE Transactions on Fuzzy Systems, 2009, 17(5):1092-1105 doi: 10.1109/TFUZZ.2009.2021953
|
|
[16]
|
莫红, 王飞跃, 赵亮.一一映射下区间二型模糊集合的语言动力学轨迹.模式识别与人工智能, 2010, 23(2):144-147 http://www.cnki.com.cn/Article/CJFDTOTAL-MSSB201002002.htmMo Hong, Wang Fei-Yue, Zhao Liang. LDS trajectories under one-to-one mappings in interval type-2 fuzzy sets. Pattern Recognition and Artificial Intelligence, 2010, 23(2):144-147 http://www.cnki.com.cn/Article/CJFDTOTAL-MSSB201002002.htm
|
|
[17]
|
莫红, 王飞跃, 肖志权, 陈茜.基于区间二型模糊集合的语言动力系统稳定性.自动化学报, 2011, 37(8):1018-1024 http://www.aas.net.cn/CN/abstract/abstract17522.shtmlMo Hong, Wang Fei-Yue, Xiao Zhi-Quan, Chen Qian. Stabilities of linguistic dynamic systems based on interval type-2 fuzzy sets. Acta Automatica Sinica, 2011, 37(8):1018-1024 http://www.aas.net.cn/CN/abstract/abstract17522.shtml
|
|
[18]
|
张伟斌, 胡怀中, 刘文江.基于二型模糊逻辑的交通流量预测.西安交通大学学报, 2007, 41(10):1160-1164 doi: 10.3321/j.issn:0253-987x.2007.10.008Zhang Wei-Bin, Hu Huai-Zhong, Liu Wen-Jiang. Traffic flow forecast based on type-2 fuzzy logic approach. Journal of Xi'an Jiaotong University, 2007, 41(10):1160-1164 doi: 10.3321/j.issn:0253-987x.2007.10.008
|
|
[19]
|
李成栋, 易建强, 余意, 赵冬斌.柔索驱动并联机构的二型模糊神经逆控制.自动化学报, 2010, 36ć(3):459-464 http://www.aas.net.cn/CN/abstract/abstract13692.shtmlLi Cheng-Dong, Yi Jian-Qiang, Yu Yi, Zhao Dong-Bin. Inverse control of cable-driven parallel mechanism using type-2 fuzzy neural network. Acta Automatica Sinica, 2010, 36(3):459-464 http://www.aas.net.cn/CN/abstract/abstract13692.shtml
|
|
[20]
|
Zadeh L A. Fuzzy sets. Information and Control, 1965, 8(3):338-353 doi: 10.1016/S0019-9958(65)90241-X
|
|
[21]
|
Mo H, Wang F Y, Zhou M, Li R M, Xiao Z Q. Footprint of uncertainty for type-2 fuzzy sets. Information Sciences, 2014, 272:96-110 doi: 10.1016/j.ins.2014.02.092
|
|
[22]
|
Mo H, Wang J, Li X, Wu Z L. Linguistic Dynamic Modeling and Analysis of Psychological Health State Using Interval Type-2 Fuzzy Sets. IEEE/CAA Journal of Automatica Sinica, 2015, 2(4):366-373 doi: 10.1109/JAS.2015.7296531
|
|
[23]
|
Mendel J M. Advances in type-2 fuzzy sets and systems. Information Sciences, 2007, 177(1):84-110 doi: 10.1016/j.ins.2006.05.003
|
|
[24]
|
Mendel J M. General type-2 fuzzy logic systems made simple:a tutorial. IEEE Transactions on Fuzzy Systems, 2014, 22(5):1162-1182 doi: 10.1109/TFUZZ.2013.2286414
|
|
[25]
|
Mendel J M. Type-2 fuzzy sets and systems:a retrospective. Informatik-Spektrum, 2015, 38(6):523-532 doi: 10.1007/s00287-015-0927-4
|
|
[26]
|
Mendel J M, Rajati M R, Sussner P. On clarifying some definitions and notations used for type-2 fuzzy sets as well as some recommended changes. Information Sciences, 2016, 340-341:337-345 doi: 10.1016/j.ins.2016.01.015
|
|
[27]
|
王晓波. 2型Fuzzy集的分解定理.模糊数学, 1:9-13 http://www.cnki.com.cn/Article/CJFDTOTAL-MUTE200101003.htmWang Xiao-Bo. Decomposition theorem of type-2 fuzzy sets, Fuzzy Mathematics, 1983, 1:9-13 http://www.cnki.com.cn/Article/CJFDTOTAL-MUTE200101003.htm
|
|
[28]
|
Rickard J T, Aisbett J, Gibbon G. Fuzzy subsethood for fuzzy sets of type-2 and generalized type-n. IEEE Transactions on Fuzzy Systems, 2009, 17(1):50-60 doi: 10.1109/TFUZZ.2008.2006369
|
|
[29]
|
Mendel J M, John R I B. Type-2 fuzzy sets made simple. IEEE Transactions on Fuzzy Systems, 2002, 10(2):117-127 doi: 10.1109/91.995115
|
|
[30]
|
Mendel J M. On KM algorithms for solving type-2 fuzzy set problems. IEEE Transactions on Fuzzy Systems, 2013, 21(3):426-446 doi: 10.1109/TFUZZ.2012.2227488
|
|
[31]
|
黄宣国.空间解析几何与微分几何.上海:复旦大学出版社, 2003.Huang Xuan-Guo. Spatial Analytic Geometry and Differential Geometry. Shanghai:Press of Fudan University, 2003.
|
|
[32]
|
Wang F Y. Modeling, analysis and synthesis of linguistic dynamic systems:a computational theory. In:Proceedings of the 1995 IEEE International Workshop on Architecture for Semiotic Modeling and Situation Control in Large Complex Systems. Monterey, CA, USA:IEEE, 1995. 173-178
|
|
[33]
|
Wang F Y. Outline of a computational theory for linguistic dynamic systems:toward computing with words. International Journal of Intelligent Control and Systems, 1998, 2(2):211-224 https://www.researchgate.net/publication/285014549_Outline_of_a_computational_theory_for_linguistic_dynamic_systems_Toward_computing_with_words
|
|
[34]
|
王飞跃.词计算和语言动力学系统的基本问题和研究.自动化学报, 2005, 31(6):844-852 http://www.aas.net.cn/CN/abstract/abstract15941.shtmlWang Fei-Yue. Fundamental issues in research of computing with words and linguistic dynamic systems. Acta Automatica Sinica, 2005, 31(6):844-852 http://www.aas.net.cn/CN/abstract/abstract15941.shtml
|
|
[35]
|
Mo H, Wang F Y. Linguistic dynamic systems based on computing with words and their stabilities. Science in China Series F:Information Sciences, 2009, 52(5):780-796 doi: 10.1007/s11432-009-0089-6
|
|
[36]
|
莫红, 王飞跃.语言动力系统与二型模糊逻辑.北京:中国科学技术出版社, 2013.Mo Hong, Wang Fei-Yue. Linguistic Dynamic Systems and Type-2 Fuzzy Logic. Beijing:Chinese Press of Science and Technology, 2013.
|
|
[37]
|
Zhou J, Chen C L P, Chen L, Li H X. A collaborative fuzzy clustering algorithm in distributed network environments. IEEE Transactions on Fuzzy Systems, 2014, 22(6):1443-1456 doi: 10.1109/TFUZZ.2013.2294205
|
|
[38]
|
Chen C L P, Wang J, Wang C H, Chen L. A new learning algorithm for a fully connected neuro-fuzzy inference system. IEEE Transactions on Neural Networks and Learning Systems, 2014, 25(10):1741-1757 doi: 10.1109/TNNLS.2014.2306915
|




 下载:
下载: