Standoff Tracking Control With Respect to Moving Target via Finite-time Stabilization
-
摘要: 针对单无人机对单目标的环航跟踪问题，设计了一个能保证无人机在速度有界条件下，飞行轨迹快速收敛到期望航迹的控制器.1）根据无人机运动特性，设计了一个考虑目标运动状态的控制方案，并利用Lyapunov稳定性定理给出了系统渐近稳定的充分条件.2）结合饱和控制和有限时间控制，得到使无人机相对目标距离在有限时间内收敛到期望值的充分条件.3）用数值算例比较验证了所提控制器的有效性.
-
关键词:
- 环航跟踪 /
- Lyapunov渐近稳定 /
- 饱和控制 /
- 有限时间稳定
Abstract: To realize the standoff tracking with respect to a moving target, this paper presents a controller to enable the unmanned aerial vehicle (UAV) to rapidly track the desired circular path with speed constraint. According to the kinetic characteristics of UAV, a control strategy considering the target motion state is given, and a corresponding sufficient condition ensuring asymptotic stability is derived with the Lyapunov stability theory. Using finite-time and saturated control techniques, a control law is proposed which ensures the relative distance converges to the desired value in a finite time. Simulation results verify the effectiveness of the proposed control law.1) 本文责任编委 朱纪洪 -
图 3 饱和约束下的有限时间稳定控制参数
Fig. 3 Control parameters of flnite-time stability subject to saturation
图 4 目标速度对环航跟踪误差的影响
Fig. 4 The influences of the target velocity on the standofi tracking errors
-
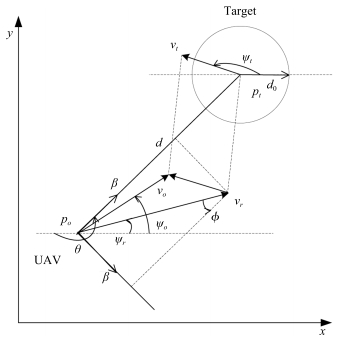
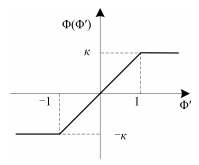
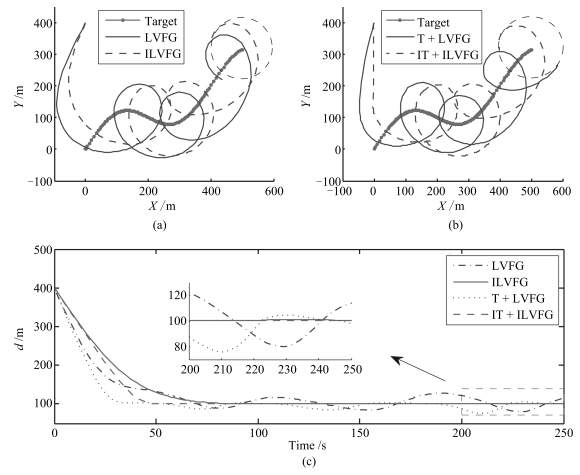
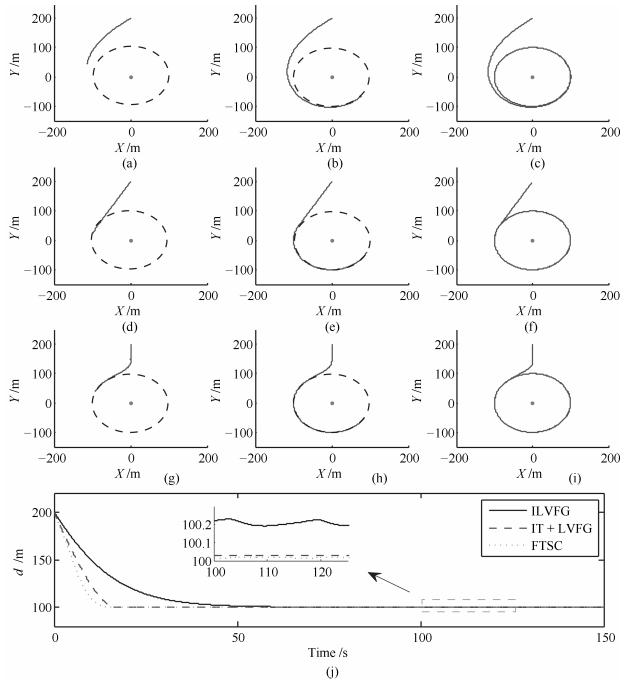
[1] Deghat M, Shames I, Anderson B D O, Yu C B. Localization and circumnavigation of a slowly moving target using bearing measurements. IEEE Transactions on Automatic Control, 2014, 59(8):2182-2188 doi: 10.1109/TAC.2014.2299011 [2] Deghat M, Davis E, See T, Shames I, Anderson B D O, Yu C B. Target localization and circumnavigation by a non-holonomic robot. In:Proceedings of the 2012 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS). Vilamoura, Algarve, Portugal:IEEE, 2012. 1227-1232 [3] Deghat M, Xia L, Anderson B, Hong Y G. Multi-target localization and circumnavigation by a single agent using bearing measurements. International Journal of Robust and Nonlinear Control, 2015, 25(14):2362-2374 doi: 10.1002/rnc.v25.14 [4] Shames I, Dasgupta S, Fidan B, Anderson B D O. Circumnavigation using distance measurements under slow drift. IEEE Transactions on Automatic Control, 2012, 57(4):889-903 doi: 10.1109/TAC.2011.2173417 [5] Cao Y C, Muse J, Casbeer D, Kingston D. Circumnavigation of an unknown target using UAVs with range and range rate measurements. In:Proceedings of the 52nd IEEE Conference on Decision and Control (CDC). Firenze, Italy:IEEE, 2013. 3617-3622 [6] 张民, 田鹏飞, 陈欣.一种无人机定距盘旋跟踪制导律及稳定性证明.航空学报, 2016, 37(11):3425-3434 http://d.old.wanfangdata.com.cn/Periodical/hkxb201611020Zhang Min, Tian Peng-Fei, Chen Xin. UAV guidance law for circumnavigating and tracking ground target and its stability proof. Acta Aeronautica et Astronautica Sinica, 2016, 37(11):3425-3434 http://d.old.wanfangdata.com.cn/Periodical/hkxb201611020 [7] Matveev A S, Semakova A A, Savkin A V. Range-only based circumnavigation of a group of moving targets by a non-holonomic mobile robot. Automatica, 2016, 65:76-89 doi: 10.1016/j.automatica.2015.11.032 [8] Zhang M F, Liu H H T. Vision-based tracking and estimation of ground moving target using unmanned aerial vehicle. In:Proceedings of the 2010 American Control Conference. Baltimore, MD, USA:IEEE, 2010. 6968-6973 [9] Zhu S Q, Wang D W, Chen Q J. Standoff tracking control of moving target in unknown wind. In:Proceedings of the 48th IEEE Conference on Decision and Control, 2009 Held Jointly with the 28th Chinese Control Conference. Shanghai, China:IEEE, 2009. 776-781 [10] Lawrence D A. Lyapunov vector fields for UAV flock coordination. In:Proceedings of the 2nd AIAA "Unmanned Unlimited" Conference and Workshop and Exhibit. San Diego, California, USA:AIAA, 2003. [11] Frew E W, Lawrence D A, Morris S. Coordinated standoff tracking of moving targets using Lyapunov guidance vector fields. Journal of Guidance, Control, and Dynamics, 2008, 31(2):290-306 doi: 10.2514/1.30507 [12] Summers T H, Akella M R, Mears M J. Coordinated standoff tracking of moving targets:control laws and information architectures. Journal of Guidance, Control, and Dynamics, 2009, 32(1):56-69 doi: 10.2514/1.37212 [13] Yoon S, Park S, Kim Y. Circular motion guidance law for coordinated standoff tracking of a moving target. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(4):2440-2462 doi: 10.1109/TAES.2013.6621827 [14] Wang X, Zhang D B, Shen L C, Zhang J W. A virtual force approach for cooperative standoff target tracking using multiple robots. In:Proceedings of the 29th Chinese Control and Decision Conference. Yinchuan, China:IEEE, 2016. 1348-1353 [15] Oh H, Kim S, Shin H S, White B A, Tsourdos A, Rabbath C A. Rendezvous and standoff target tracking guidance using differential geometry. Journal of Intelligent and Robotic Systems, 2013, 69(1-4):389-405 doi: 10.1007/s10846-012-9751-0 [16] Shames I, Fidan B, Anderson B D O. Close target reconnaissance with guaranteed collision avoidance. International Journal of Robust and Nonlinear Control, 2011, 21(16):1823-1840 doi: 10.1002/rnc.v21.16 [17] Chen H D, Chang K C, Agate C S. UAV path planning with tangent-plus-Lyapunov vector field guidance and obstacle avoidance. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(2):840-856 doi: 10.1109/TAES.2013.6494384 [18] Kim S, Oh H, Tsourdos A. Nonlinear model predictive coordinated standoff tracking of a moving ground vehicle. Journal of Guidance, Control, and Dynamics, 2013, 36(2):557-566 doi: 10.2514/1.56254 [19] Oh H, Kim S, Tsourdos A. Road-map-assisted standoff tracking of moving ground vehicle using nonlinear model predictive control. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(2):975-986 doi: 10.1109/TAES.2014.130688 [20] Oh H, Kim S, Tsourdos A, White B A. Decentralised standoff tracking of moving targets using adaptive sliding mode control for UAVs. Journal of Intelligent and Robotic Systems, 2014, 76(1):169-183 doi: 10.1007/s10846-013-9864-0 [21] Quintero S A P, Papi F, Klein D J, Chisci L, Hespanha J P. Optimal UAV coordination for target tracking using dynamic programming. In:Proceedings of the 49th IEEE Conference on Decision and Control (CDC). Atlanta, GA, USA:IEEE, 2010. 4541-4546 [22] Oh H, Turchi D, Kim S, Tsourdos A, Pollini L, White B. Coordinated standoff tracking using path shaping for multiple UAVs. IEEE Transactions on Aerospace and Electronic Systems, 2014, 50(1):348-363 doi: 10.1109/TAES.2013.110712 [23] Zheng R H, Lin Z Y, Fu M Y, Sun D. Distributed control for uniform circumnavigation of ring-coupled unicycles. Automatica, 2015, 53:23-29 doi: 10.1016/j.automatica.2014.11.012 [24] Zhu S Q, Wang D W. Adversarial ground target tracking using UAVs with input constraints. Journal of Intelligent and Robotic Systems, 2012, 65(1-4):521-532 doi: 10.1007/s10846-011-9574-4 [25] Zhu S Q, Wang D W, Low C B. Ground target tracking using UAV with input constraints. Journal of Intelligent and Robotic Systems, 2013, 69(1-4):417-429 doi: 10.1007/s10846-012-9737-y [26] 杨晨, 程盈盈, 都海波, 王金平, 何怡刚. Buck型变换器自适应有限时间降压控制算法研究.自动化学报, 2016, 42(2):315-320 http://www.aas.net.cn/CN/abstract/abstract18821.shtmlYang Chen, Cheng Ying-Ying, Du Hai-Bo, Wang Jin-Ping, He Yi-Gang. An adaptive finite-time control algorithm for buck converter systems. Acta Automatica Sinica, 2016, 42(2):315-320 http://www.aas.net.cn/CN/abstract/abstract18821.shtml [27] 丁世宏, 李世华.输入饱和下的非线性积分系统的全局有限时间镇定.自动化学报, 2011, 37(10):1222-1231 http://www.aas.net.cn/CN/abstract/abstract17611.shtmlDing Shi-Hong, Li Shi-Hua. Global finite-time stabilization of nonlinear integrator systems subject to input saturation. Acta Automatica Sinica, 2011, 37(10):1222-1231 http://www.aas.net.cn/CN/abstract/abstract17611.shtml [28] 周映江, 王莉, 孙长银.一类非线性系统的全局渐近稳定和有限时间镇定.自动化学报, 2013, 39(5):664-672 http://www.aas.net.cn/CN/abstract/abstract17924.shtmlZhou Ying-Jiang, Wang Li, Sun Chang-Yin. Global asymptotic and finite-time stability for nonlinear systems. Acta Automatica Sinica, 2013, 39(5):664-672 http://www.aas.net.cn/CN/abstract/abstract17924.shtml [29] 李雪冰, 马莉, 丁世宏.一类新的二阶滑模控制方法及其在倒立摆控制中的应用.自动化学报, 2015, 41(1):193-202 http://www.aas.net.cn/CN/abstract/abstract18598.shtmlLi Xue-Bing, Ma Li, Ding Shi-Hong. A new second-order sliding mode control and its application to inverted pendulum. Acta Automatica Sinica, 2015, 41(1):193-202 http://www.aas.net.cn/CN/abstract/abstract18598.shtml [30] Hong Y G. Finite-time stabilization and stabilizability of a class of controllable systems. Systems and Control Letters, 2002, 46(4):231-236 doi: 10.1016/S0167-6911(02)00119-6 [31] Bhat S P, Bernstein D S. Continuous finite-time stabilization of the translational and rotational double integrators. IEEE Transactions on Automatic Control, 1998, 43(5):678-682 doi: 10.1109/9.668834 [32] Khalil H K. Nonlinear Systems (3rd edition). New Jersey: Prentice Hall, 2002. -
下载:
图(9)
计量
- 文章访问数: 1754
- HTML全文浏览量: 212
- PDF下载量: 709
- 被引次数: 0
