-
摘要: 针对未知但有界扰动下约束非线性系统, 提出一种新的鲁棒经济模型预测控制(Economic model predictive control, EMPC)策略, 保证闭环系统对扰动输入具有输入到状态稳定性(Input-to-state stability, ISS). 基于微分对策原理, 分别优化经济目标函数和关于最优经济平衡点的鲁棒稳定性目标函数, 其中经济最优性与鲁棒稳定性是具有冲突的两个控制目标. 利用鲁棒稳定性目标最优值函数构造EMPC优化的隐式收缩约束, 建立鲁棒EMPC的递推可行性和闭环系统关于最优经济平衡点相对于有界扰动输入到状态稳定性结果. 最后以连续搅拌反应器为例, 对比仿真验证本文策略的有效性.Abstract: This paper proposes a novel robust economic model predictive control (EMPC) scheme of constrained nonlinear systems with unknown but bounded disturbances, with guaranteed input-to-state stability (ISS) of the closed-loop system with respect to the disturbance. Based on the principle of differential game, economic objective functions and robust stability objective functions on economically optimal equilibrium points are optimized simultaneously, which are two conflicting control goals. The optimal value function of the robust objective is used to design an implicit contractive constraint of the EMPC optimization, which guarantees ISS of the closed-loop system at the equilibrium point with respect to the disturbance. Some sufficient conditions for recursive feasibility and ISS with respect to the disturbance are presented. Finally, an example of a continuously stirred tank reactor is utilized to illustrate the effectiveness of the proposed scheme.
-
图 2 持续扰动
$w(k)=0.1436\sin(k/2) $ 的仿真结果Fig. 2 Simulation results under continuous disturbance
$w(k)=0.1436\sin(k/2) $ 
图 3 持续扰动
$w(k)=0.1436\exp(-k/10) $ 的仿真结果Fig. 3 Simulation results under continuous disturbance
$w(k)=0.1436\exp(-k/10) $ 
图 5 不同初始状态的闭环系统相轨迹
Fig. 5 Phase trajectories of the closed-loop system from different initial states
表 1 平均经济性能和收敛过渡时间
Table 1 Average economic performance and transient time
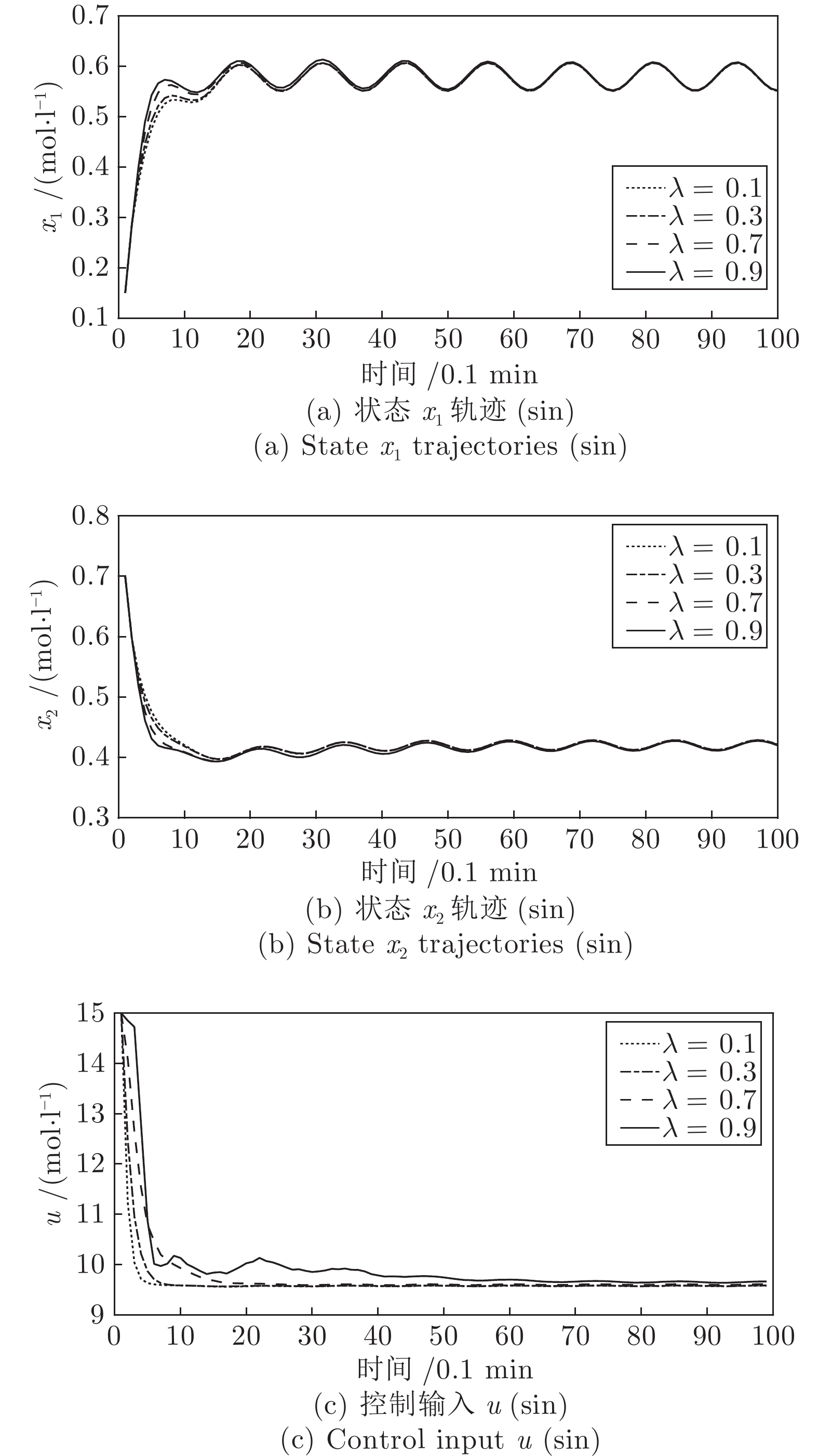
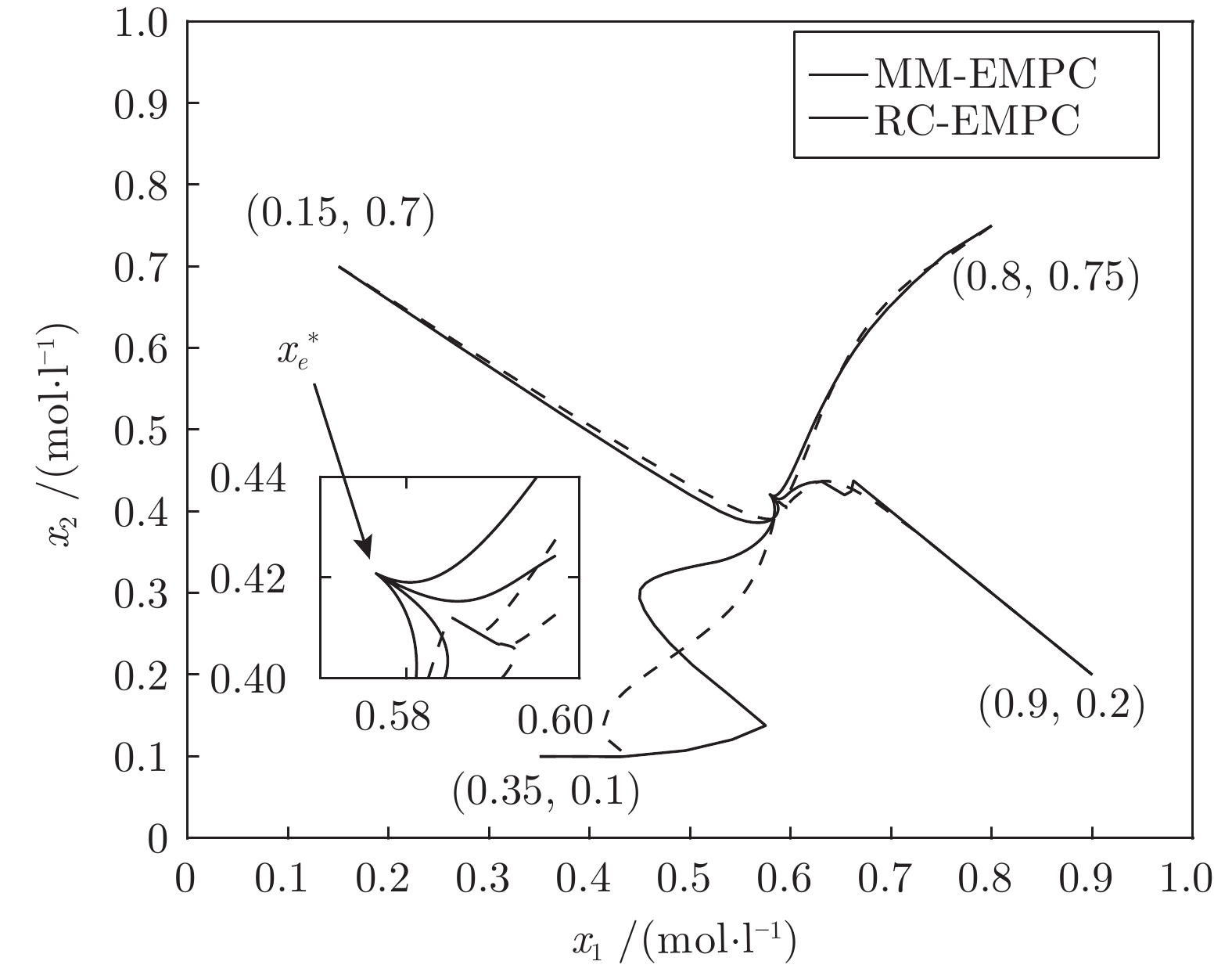
$\lambda $ $w(k)= 0.1436\sin ( {k/} 2 )$ $w(k)=0.1436\exp( - {{k/} }10)$ ${J_{{\rm{ave}}} } $ ${J_{{\rm{ave}}} } $ ${ {{T} }_{ {\text{tr} } } }$ 0.1 −3.4361 −3.3953 49Ts 0.3 −3.4464 −3.4019 54Ts 0.5 −3.4553 −3.4083 58Ts 0.7 −3.4623 −3.4143 65Ts 0.9 −3.4712 −3.4194 74Ts 
下载: 导出CSV
-
[1] Mayne D Q. Model predictive control: recent developments and future promise. Automatica, 2014, 50(12): 2967-2986. doi: 10.1016/j.automatica.2014.10.128 [2] Ellis M, Durand H, Christofides P D. A tutorial review of economic model predictive control methods. Journal of Process Control, 2014, 24(8): 1156-1178. doi: 10.1016/j.jprocont.2014.03.010 [3] Liu X J, Cui J H. Economic model predictive control of boiler-turbine system. Journal of Process Control, 2018, 66: 59-67. doi: 10.1016/j.jprocont.2018.02.010 [4] 何德峰, 张永达, 李廉明, 仇翔. 循环流化床燃烧系统无终端约束字典序经济预测控制. 化工学报, 2020, 71(3): 1210-1216.He De-Feng, Zhang Yong-Da, Li Lian-Ming, Qiu Xiang. Lexicographic economic predictive control without terminal constraints for CFBB combustion systems. CIESC Journal, 2020, 71(3): 1210-1216 (in Chinese). [5] Clarke W C, Manzie C, Brear B J. Hierarchical economic MPC for systems with storage states. Automatica, 2018, 94: 138-150. doi: 10.1016/j.automatica.2018.04.012 [6] Tian H, Lu Q G, Gopaluni R B, Zavala V M, Olson J A. An economic model predictive control framework for mechanical pulping processes. Control Engineering Practice, 2019, 85: 100-109. doi: 10.1016/j.conengprac.2019.01.008 [7] He D F, Qiu T X, Luo R S. Fuel efficiency-oriented platooning control of connected nonlinear vehicles: A distributed economic MPC approach. Asian Journal of Control, 2019, 21: 1-11. doi: 10.1002/asjc.2054 [8] Farooqi H, Fagiano L, Colaneri P, Barlini D. Shrinking horizon parametrized predictive control with application to energy-efficient train operation. Automatica, 2020, 112, 108635 [9] 张博, 郭戈, 王丽媛, 王琼. 基于信号灯状态的燃油最优车速规划与控制. 自动化学报, 2018, 44(3): 461-470.Zhang Bo, Guo Ge, Wang Li-Yuan, Wang Qiong. Vehicle speed planning and control for fuel consumption optimization with traffic light state. Acta Automatica Sinica, 2018, 44(3): 461-470 (in Chinese). [10] Diehl M Q, Amrit R, Rawlings J B. A Lyapunov function for economic optimizing model predictive control. IEEE Transactions on Automatic Control, 2011, 56(3): 703-707. doi: 10.1109/TAC.2010.2101291 [11] Ferramosca A, Limon D, Camacho E F. Economic MPC for a changing economic criterion for linear systems. IEEE Transactions on Automatic Control, 2014, 59(10): 2657-2667. doi: 10.1109/TAC.2014.2326013 [12] Angeli D, Amrit R, Rawlings J B. On average performance and stability of economic model predictive control. IEEE Transactions on Automatic Control, 2012, 57(7): 1615-1626. doi: 10.1109/TAC.2011.2179349 [13] Amrit R, Rawlings J B, Angeli D. Economic optimization using model predictive control with a terminal cost. Annual Reviews in Control, 2011, 35(2): 178-186. doi: 10.1016/j.arcontrol.2011.10.011 [14] 杨亚茹, 李少远. 切换非线性系统全局优化运行的经济预测控制. 自动化学报, 2017, 43(6): 1017-1027.Yang Ya-Ru, Li Shao-Yuan. Economic Model predictive control for global optimal operation of nonlinear switching systems. Acta Automatica Sinica, 2017, 43(6): 1017-1027 (in Chinese). [15] Limon D, Pereira M, De la Pena D M, Alamo T, Grosso J M. Single-layer economic model predictive control for periodic operation. Journal of Process Control, 2014, 24(8): 1207-1224. doi: 10.1016/j.jprocont.2014.03.013 [16] Grüne L. Economic receding horizon control without terminal constraints. Automatica, 2013, 49(3): 725-734. doi: 10.1016/j.automatica.2012.12.003 [17] Müller M A, Grüne L. Economic model predictive control without terminal constraints for optimal periodic behavior. Automatica, 2016, 70(8): 128-139. [18] Müller M A, Angeli D, Allgöwer F, Amrit R, Rawlings J B. Convergence in economic model predictive control with average constraints. Automatica, 2014, 50(12): 3100-3111. doi: 10.1016/j.automatica.2014.10.059 [19] Zavala V M. A multiobjective optimization perspective on the stability of economic MPC. IFAC-PapersOnLine, 2015, 48(8): 974-980. doi: 10.1016/j.ifacol.2015.09.096 [20] 何德峰. 约束非线性系统稳定经济模型预测控制. 自动化学报, 2016, 42(11): 1680-1690.He De-Feng. Stabilizing economic model predictive control of constrained nonlinear systems. Acta Automatica Sinica, 2016, 42(11): 1680-1690 (in Chinese). [21] He D F, Sun J, Yu L. Economic MPC with a contractive constraint for nonlinear systems. International Journal of Robust and Nonlinear Control, 2016, 26(18): 4072-4087. doi: 10.1002/rnc.3549 [22] Yu S Y, Reble M, Chen H, Allgöwer F. Inherent robustness properties of quasi-infinite horizon nonlinear model predictive control. Automatica, 2014, 50(9): 2269-2280. doi: 10.1016/j.automatica.2014.07.014 [23] Limon D, Alamo T, Camacho E F. Input-to-state stable MPC for constrained discrete-time nonlinear systems with bounded additive uncertainties. In: Proceedings of the 41st IEEE Conference on Decision and Control. Las Vegas, NV, USA: IEEE, 2002.4619−4624. [24] Yu S Y, Maier C, Chen H, Allgöwer F. Tube MPC scheme based on robust control invariant set with application to Lipschitz nonlinear systems. Systems & Control Letters, 2013, 62(2): 194-200. [25] Mayne D Q, Kerrigan E F, Wyk E, Falugi P. Tube-based robust nonlinear model predictive control. International Journal of Robust and Nonlinear Control, 2011, 21: 1341-1353. doi: 10.1002/rnc.1758 [26] Magni L, De Nicolao G, Scattolini R, Allgöwer F. Robust model predictive control for nonlinear discrete-time systems. International Journal of Robust and Nonlinear Control, 2003, 13: 229-246. doi: 10.1002/rnc.815 [27] Magni L, Raimondo D M, Scattolini R. Regional input-to-state stability for nonlinear model predictive control. IEEE Transactions on Automatic Control, 2006, 51(9): 1548-1553. doi: 10.1109/TAC.2006.880808 [28] Limon D, Alamo T, Salas F, Camacho E F. Input-to-state stability of min-max MPC controllers for nonlinear systems with bounded uncertainties. Automatica, 2006, 42(5): 797-803. doi: 10.1016/j.automatica.2006.01.001 [29] He D F, Zheng T, Ji H B. On robustness of suboptimal min-max model predictive control. WSEAS Transactions on Systems and Control, 2007, 2(8): 428-433. [30] 何德峰, 季海波, 郑涛. 持续有界扰动下的非线性H∞鲁棒预测控制. 自动化学报, 2008, 34(2): 215-219.He De-Feng, Ji Hai-Bo, Zheng Tao. Nonlinear H∞ robust predictive control with bounded persistent disturbances. Acta Automatica Sinica, 2008, 34(2): 215-219 (in Chinese). [31] He D F, Ji H B, Zheng T. On robustness of constrained non-linear H∞ predictive controllers with disturbances. International Journal of Systems Science, 2010, 41(2): 203-212. doi: 10.1080/00207720903045742 [32] Lazar M, Munoz de la Pena D, Heemels W P M H, Alamo T. On input-to-state stability of min-max nonlinear model predictive control. Systems of Control Letters, 2008, 57: 39-48. doi: 10.1016/j.sysconle.2007.06.013 [33] Broomhead T J, Manzie C, Shekhar R C, Hield P. Robust stable economic MPC with applications in engine control. In: Proceedings of the 53rd IEEE Conference on Decision and Control. Los Angeles, CA, USA: IEEE, 2014. 2511−2516 [34] Broomhead T J, Manzie C, Shekhar R C, Hield P. Robust periodic economic MPC for linear systems. Automatica, 2015, 60(10): 30-37. [35] Griffith D W, Zavala V M, Biegler L T. Robustly stable economic NMPC for non-dissipative stage costs. Journal of Process Control, 2017, 57: 116-126. doi: 10.1016/j.jprocont.2017.06.016 [36] Alessandretti A, Aguiar A P, Jones C N. An input-to-state stability approach to economic optimization in model predictive control. IEEE Transactions on Automatic Control, 2017, 62(12): 6081-6093. doi: 10.1109/TAC.2017.2700388 [37] Zhao M, Jiang C C, She M H. Robust contractive economic MPC for nonlinear systems with additive disturbance. International Journal of Control, Automatic and Systems, 2018, 16(5): 2253-2263. doi: 10.1007/s12555-017-0669-y [38] He D F, Qiu T X, Lu L Y. Input-to-state stability of contractive EMPC of nonlinear systems with bounded disturbances. IET Control Theory & Applications, 2019, 13(5): 651-658. -
图(5) / 表(1)
计量
- 文章访问数: 919
- HTML全文浏览量: 422
- PDF下载量: 304
- 被引次数: 0
