Simultaneous Estimation of Actuator and Sensor Faults for Uncertain Time-delayed Markovian Jump Systems
-
摘要: 针对具有参数不确定和延迟环节的马尔科夫跳变系统，在状态转移概率矩阵（Transition probability matrix，TPM）不确定的情形下，讨论了其执行器和传感器故障同时估计的方法.通过扩展系统状态，将系统转换为一个具有马尔科夫跳变参数的广义描述系统，基于此广义描述系统设计马尔科夫跳变观测器实现对其状态和传感器故障的估计.与此同时，还设计了一组自适应律对执行器故障进行在线调节.通过求解一组线性矩阵不等式最优化问题，得到观测器存在的充分条件.最后，针对两个数值实例，验证了所设计方法的有效性.Abstract: In this paper, a method of simultaneous estimation of actuator and sensor faults based on a class of uncertain time-delayed Markovian jump systems is presented. The transition probability matrix (TPM) is assumed to be uncertain. By extending the sensor faults as an auxiliary state, the system is converted into a generalized descriptor system with Markovian jump parameters. Based on the transformed system, a Markovian jump observer is designed to provide the estimates of the states and sensor faults. Meanwhile, a set of adaptive laws are given to adjust the actuator faults. Sufficient conditions of existence of the observer are given in terms of a linear matrix inequality optimization problem. Finally, two numerical examples are simulated to validate the effectiveness of the proposed approach.
-
图 10 F-404模型执行器故障估计曲线
Fig. 10 The curve of the estimation of actuator fault of Model F-404
图 11 F-404模型传感器故障估计曲线
Fig. 11 The curve of the estimation of sensor fault of Model F-404
-
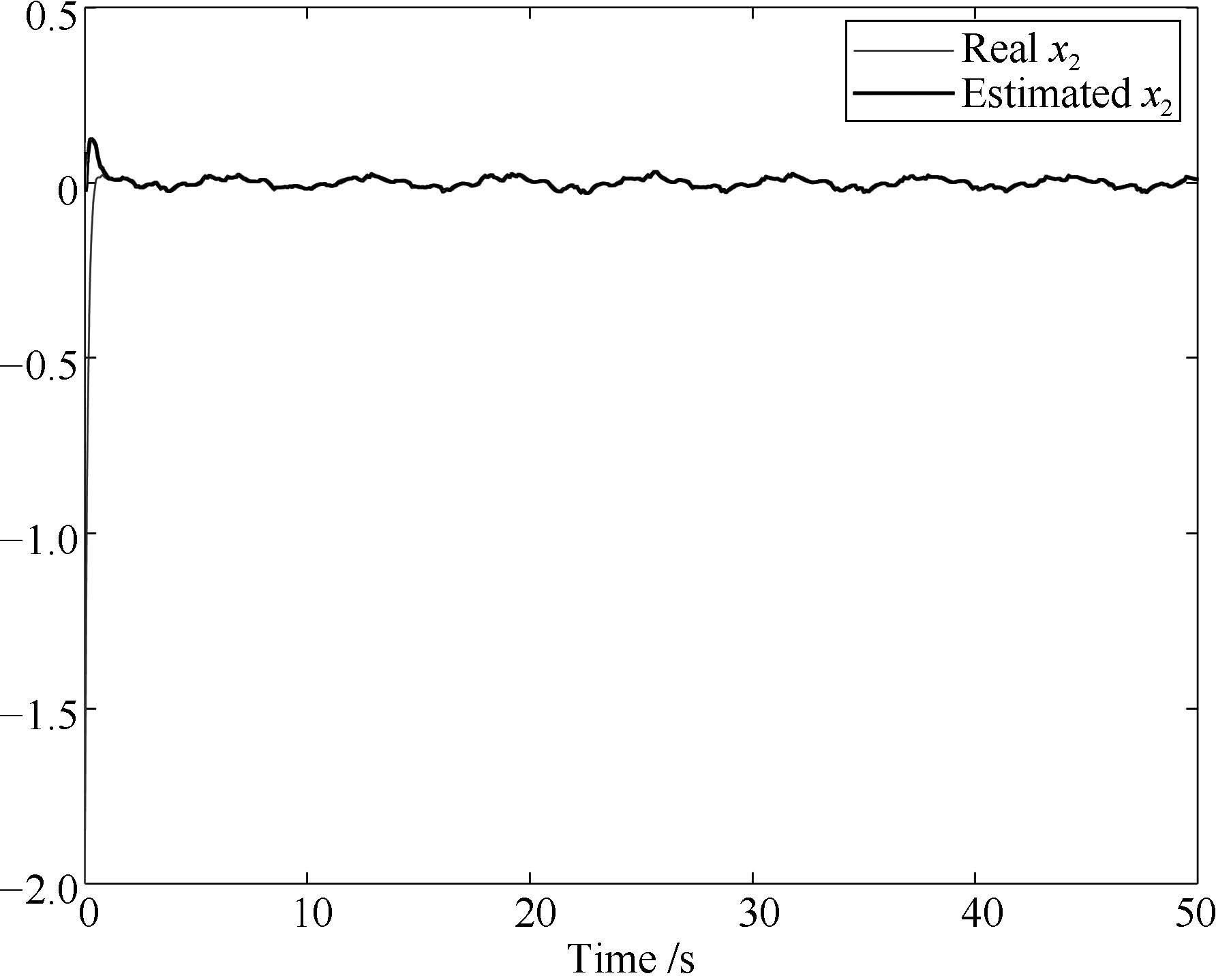
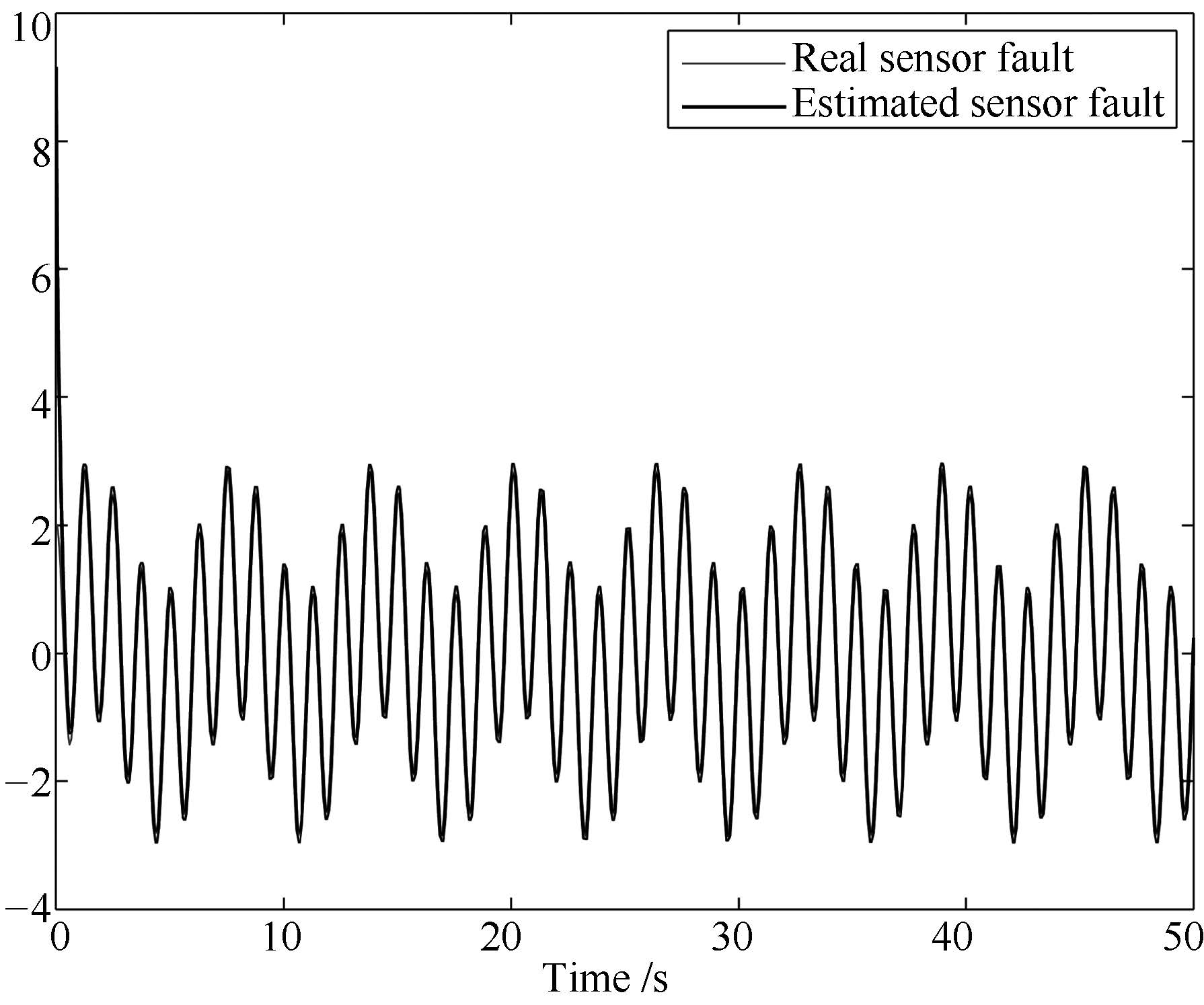
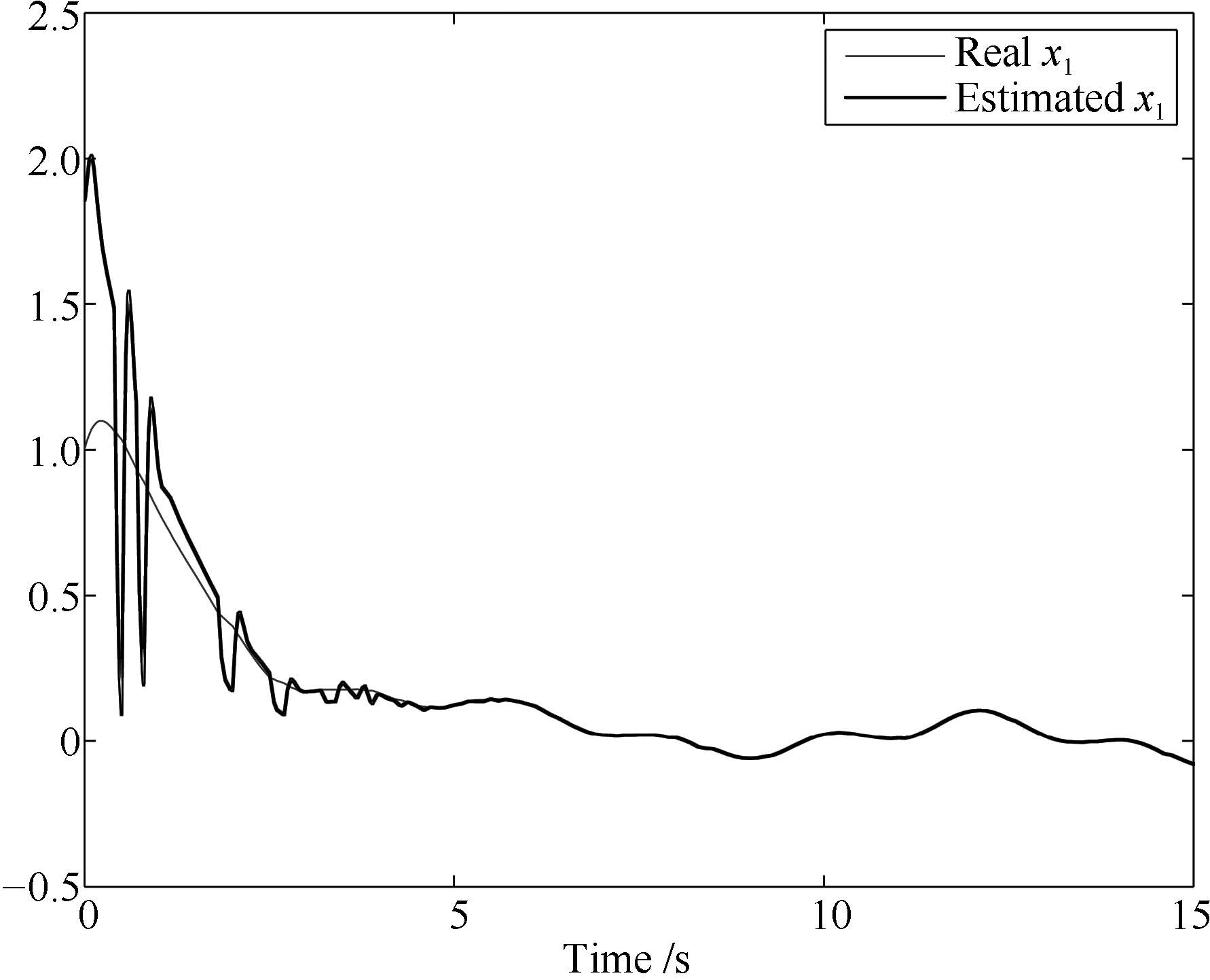
[1] Mahmoud M S, Shi P. Robust Kalman filtering for continuous time-lag systems with Markovian jump parameters. IEEE Transactions on Circuits and Systems I:Fundamental Theory and Applications, 2003, 50(1):98-105 doi: 10.1109/TCSI.2002.807504 [2] Park B Y, Kwon N K, Park P G. Stabilization of Markovian jump systems with incomplete knowledge of transition probabilities and input quantization. Journal of the Franklin Institute, 2015, 352(10):4354-4365 doi: 10.1016/j.jfranklin.2015.06.008 [3] Liu M, Ho D W C, Shi P. Adaptive fault-tolerant compensation control for Markovian jump systems with mismatched external disturbance. Automatica, 2015, 58:5-14 doi: 10.1016/j.automatica.2015.04.022 [4] Wu L G, Su X J, Shi P. Output feedback control of Markovian jump repeated scalar nonlinear systems. IEEE Transactions on Automatic Control, 2014, 59(1):199-204 doi: 10.1109/TAC.2013.2267353 [5] Zhang Y, He Y, Wu M, Zhang J. Stabilization for Markovian jump systems with partial information on transition probability based on free-connection weighting matrices. Automatica, 2011, 47(1):79-84 doi: 10.1016/j.automatica.2010.09.009 [6] 田恩刚, 岳东, 杨继全. 具有随机非线性和部分转移概率未知的马尔科夫系统的H∞控制. 控制理论与应用, 2014, 31(3):392-396Tian En-Gang, Yue Dong, Yang Ji-Quan. H∞ control for Markovian jump systems with incomplete transition probabilities and probabilistic nonlinearities. Control Theory&Applications, 2014, 31(3):392-396 [7] Li F B, Wu L G, Shi P, Lim C C. State estimation and sliding mode control for semi-Markovian jump systems with mismatched uncertainties. Automatica, 2015, 51:385-393 doi: 10.1016/j.automatica.2014.10.065 [8] Du B Z, Lam J, Zou Y, Shu Z. Stability and stabilization for Markovian jump time-delay systems with partially unknown transition rates. IEEE Transactions on Circuits and Systems I:Regular Papers, 2013, 60(2):341-351 doi: 10.1109/TCSI.2012.2215791 [9] Wu L G, Shi P, Gao H J. State estimation and sliding-mode control of Markovian jump singular systems. IEEE Transactions on Automatic Control, 2010, 55(5):1213-1219 doi: 10.1109/TAC.2010.2042234 [10] Zhang Y S, Xu S Y, Chu Y M. Sliding mode observer-controller design for uncertain Markovian jump systems with time delays. International Journal of Robust and Nonlinear Control, 2012, 22(4):355-368 doi: 10.1002/rnc.v22.4 [11] 高飞, 张洪钺. 带马尔科夫参数时滞容错控制系统稳定性分析. 北京航空航天大学学报, 2006, 32(5):566-570 http://www.cnki.com.cn/Article/CJFDTOTAL-BJHK200605016.htmGao Fei, Zhang Hong-Yue. Stability of time-delay fault tolerant control systems with Markovian parameters. Journal of Beijing University of Aeronautics and Astronautics, 2006, 32(5):566-570 http://www.cnki.com.cn/Article/CJFDTOTAL-BJHK200605016.htm [12] Li F B, Wu L G, Shi P. Stochastic stability of semi-Markovian jump systems with mode-dependent delays. International Journal of Robust and Nonlinear Control, 2014, 24(18):3317-3330 doi: 10.1002/rnc.v24.18 [13] Kao Y G, Li W, Wang C H. Nonfragile observer-based H∞ sliding mode control for Itô stochastic systems with Markovian switching. International Journal of Robust and Nonlinear Control, 2014, 24(15):2035-2047 doi: 10.1002/rnc.v24.15 [14] Cong S, Zhang H T, Zou Y. A new exponential stability condition for delayed systems with Markovian switching. Acta Automatica Sinica, 2010, 36(7):1025-1029 http://cn.bing.com/academic/profile?id=82bb61a498a6778a2d539a6c0446bbab&encoded=0&v=paper_preview&mkt=zh-cn [15] 宋杨, 董豪, 费敏锐. 基于切换频度的马尔科夫网络控制系统均方指数镇定. 自动化学报, 2012, 38(5):876-881 doi: 10.3724/SP.J.1004.2012.00876Song Yang, Dong Hao, Fei Min-Rui. Mean square exponential stabilization of Markov networked control systems based on switching frequentness. Acta Automatica Sinica, 2012, 38(5):876-881 doi: 10.3724/SP.J.1004.2012.00876 [16] Liu M, Shi P, Zhang L X, Zhao X D. Fault-tolerant control for nonlinear Markovian jump systems via proportional and derivative sliding mode observer technique. IEEE Transactions on Circuits and Systems I:Regular Papers, 2011, 58(11):2755-2764 doi: 10.1109/TCSI.2011.2157734 [17] Yu J Y, Liu M, Yang W, Shi P, Tong S Y. Robust fault detection for Markovian jump systems with unreliable communication links. International Journal of Systems Science, 2013, 44(11):2015-2026 doi: 10.1080/00207721.2012.683832 [18] Shi P, Liu M, Zhang L X. Fault-tolerant sliding-mode-observer synthesis of Markovian jump systems using quantized measurements. IEEE Transactions on Industrial Electronics, 2015, 62(9):5910-5918 doi: 10.1109/TIE.2015.2442221 [19] Chen L H, Huang X L, Fu S S. Fault-tolerant control for Markovian jump delay systems with an adaptive observer approach. Circuits, Systems, and Signal Processing, DOI: 10.1007/s00034-016-0277-8 [20] Li H Y, Gao H J, Shi P, Zhao X D. Fault-tolerant control of Markovian jump stochastic systems via the augmented sliding mode observer approach. Automatica, 2014, 50(7):1825-1834 doi: 10.1016/j.automatica.2014.04.006 [21] 王国良, 孙广兢, 薄海英. 广义马尔科夫跳变系统的部分模态依赖观测器设计. 控制与决策, 2015, 30(4):733-738 http://www.cnki.com.cn/Article/CJFDTOTAL-KZYC201504025.htmWang Guo-Liang, Sun Guang-Jin, Bo Hai-Ying. Partially mode-dependent observer design of singular Markovian jump systems. Control Theory&Applications, 2015, 30(4):733-738 http://www.cnki.com.cn/Article/CJFDTOTAL-KZYC201504025.htm [22] Shen H, Xu S Y, Zhou J P, Lu J J. Fuzzy H∞ filtering for nonlinear Markovian jump neutral systems. International Journal of Systems Science, 2011, 42(5):767-780 doi: 10.1080/00207721003790351 [23] Zhang X M, Lu G P, Zheng Y F. Observer design for descriptor Markovian jumping systems with nonlinear perturbations. Circuits, Systems&Signal Processing, 2008, 27(1):95-112 http://cn.bing.com/academic/profile?id=234d086e260c7b7eca39784a2048b582&encoded=0&v=paper_preview&mkt=zh-cn [24] He S P, Liu F. Adaptive observer-based fault estimation for stochastic Markovian jumping systems. Abstract and Applied Analysis, 2012, 2012:Article ID 176419 [25] Liu M, Cao X B, Shi P. Fuzzy-model-based fault-tolerant design for nonlinear stochastic systems against simultaneous sensor and actuator faults. IEEE Transactions on Fuzzy Systems, 2013, 21(5):789-799 doi: 10.1109/TFUZZ.2012.2224872 [26] Kchaou M, El Hajjaji A, Toumi A. Non-fragile H∞ output feedback control design for continuous-time fuzzy systems. ISA Transactions, 2015, 54:3-14 doi: 10.1016/j.isatra.2014.05.026 [27] Yu L, Chu J. An LMI approach to guaranteed cost control of linear uncertain time-delay systems. Automatica, 1999, 35(6):1155-1159 doi: 10.1016/S0005-1098(99)00007-2 [28] Boukas E K. Stochastic Switching Systems:Analysis and Design. Berlin:Birkhäuser, 2005. [29] Jiang B, Staroswiecki M, Cocquempot V. Fault accommodation for nonlinear dynamic systems. IEEE Transactions on Automatic Control, 2006, 51(9):1578-1583 doi: 10.1109/TAC.2006.878732 [30] Yan X G, Edwards C. Nonlinear robust fault reconstruction and estimation using a sliding mode observer. Automatica, 2007, 43(9):1605-1614 doi: 10.1016/j.automatica.2007.02.008 [31] 杨俊起, 朱芳来. 基于高增益鲁棒滑模观测器的故障检测和隔离. 自动化学报, 38(12):2005-2013 doi: 10.3724/SP.J.1004.2012.02005Yang Jun-Qi, Zhu Fang-Lai. FDI based on high-gain robust sliding mode observers. Acta Automatica Sinica, 2012, 38(12):2005-2013 doi: 10.3724/SP.J.1004.2012.02005 -
下载:
图(12)
计量
- 文章访问数: 2752
- HTML全文浏览量: 240
- PDF下载量: 969
- 被引次数: 0
