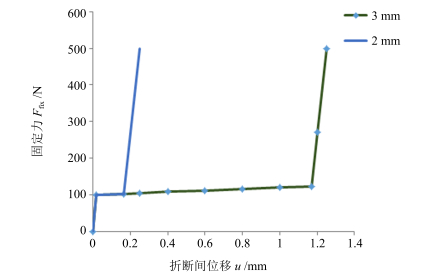
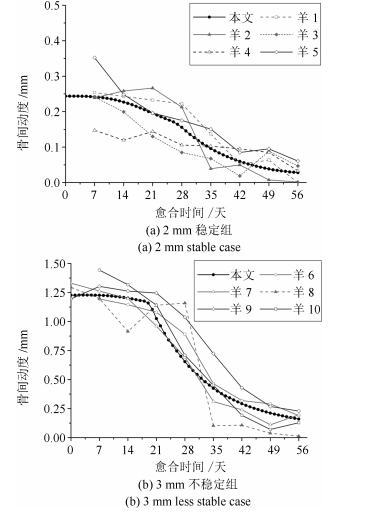
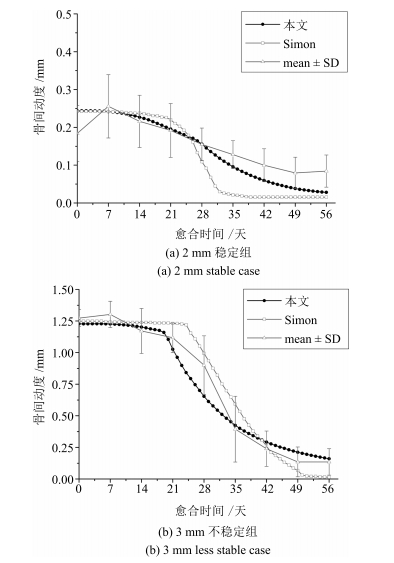
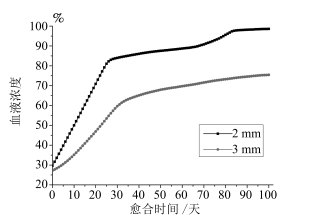
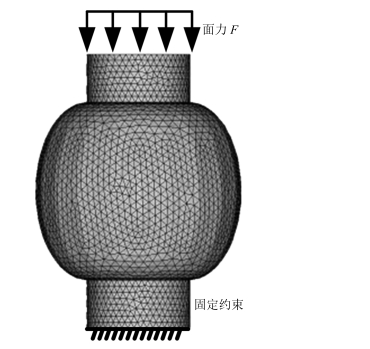
-
摘要: 为了模拟和预测肌体组织复杂的再生修复过程，提出基于力学环境和血液供给条件建立骨折愈合仿真模型.针对骨折固定的力学条件和生物学因素，用一种时间动态模型模拟二期骨折愈合阶段的机械稳定性、血管再生和组织分化之间复杂的作用关系.与以往模型不同，本研究建立骨折的三维几何模型，通过有限元法计算骨痂局部力刺激，并与模糊逻辑相结合，将血液浓度作为时-空状态变量引入到模型中，描述骨痂力学及组织分化过程.通过前进欧拉法进行组织浓度等时间步长的迭代更新，在Visual Studio 2012环境下实现愈合进程模拟.最后，利用仿真模型预测稳定与不稳定环境下骨间动度随骨折愈合时间的变化情况，并将仿真结果数据与实验数据进行对比，结果表明，仿真结果与实验数据在趋势和数值上都有较好的吻合，仿真结果数据全部分布在实验数据平均偏差范围内.该结果验证了骨折愈合模型的精确性以及在模拟骨折愈合过程方面的优势.Abstract: A fracture healing model considering mechanical environment and blood supply conditions is proposed to simulate and predict the complex regenerative repair process for tissue. For mechanics condition of fracture fixation and biological factors, a dynamic spatio-temporal model is developed to simulate the complex interactions of mechanical stability, revascularization and tissue differentiation in secondary fracture healing. Unlike previous study, a three-dimensional finite element model is established. The blood perfusion regarded as a spatio-temporal state variable is included into the model to simulate the revascularization process. With finite element method and fuzzy logic, the dynamic model can describe the callus mechanics and biological processes of tissue differentiation. The callus healing process is simulated in Visual Studio 2012 by forward Euler integration method over equidistant time steps iterative loop. Finally, the model predicates the course of interfragmentary movement of two groups with a different axial stability. Through the comparison with the experiment data, it turned out that the simulation result distributed within the scope of the average deviation of the experimental data, which corresponds well to the experiment curve trend and value. The agreement of simulation results with experiment results verifies the accuracy of the fracture healing model and the advantage of the simulating healing process.
-
Key words:
- Fracture healing /
- three-dimensional model /
- fuzzy rules /
- tissue differentiation /
- finite element
1) 本文责任编委 田捷 -
图 8 骨间动度仿真与实验数据的对比
Fig. 8 Comparison between interfragmentary movement over time and test results
图 9 骨间动度仿真与Simon仿真结果的对比
Fig. 9 Comparison between simulated interfragmentary movement and Simon results
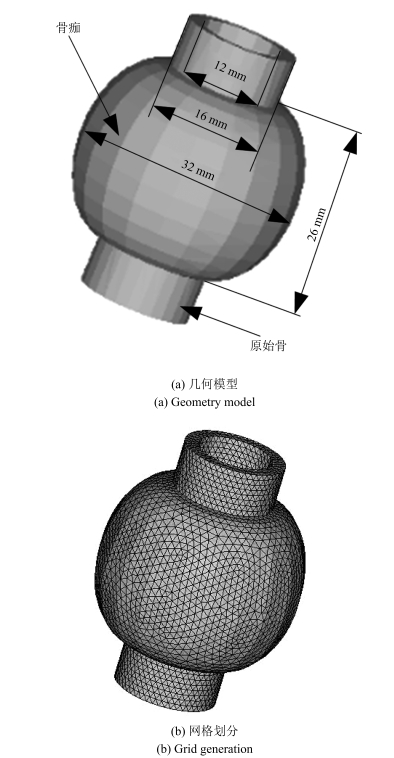
表 1 组织成分的力学特性
Table 1 Material properties of tissue types
组织成分 弹性模量(MPa) 泊松比 皮质骨 10 000 0.36 编织骨 4 000 0.36 纤维软骨 200 0.45 结缔组织 3 0.30 
下载: 导出CSV
表 2 二期骨折愈合的模糊规则
Table 2 The fuzzy rules of the fracture healing
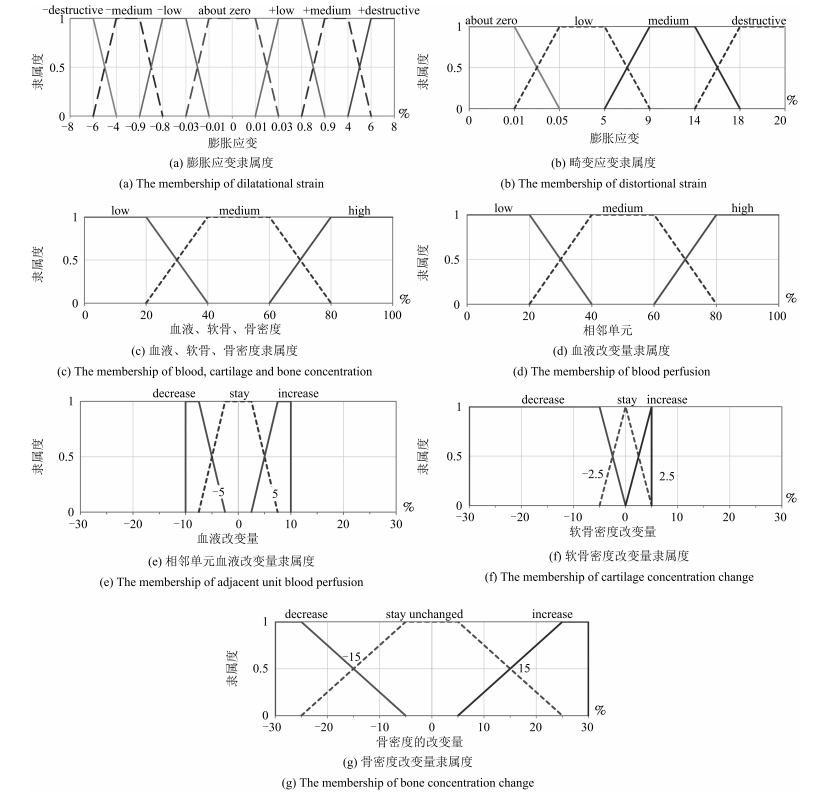
分化过程 血供 相邻血供 骨密度 邻骨密度 软骨密度 膨胀应变 畸变应变 $\Delta$血供 $\Delta$骨密度 $\Delta$软骨密度 1 低 中或高 $- $ $- $ $- $ 非负中 非中 增高 $- $ $- $ 2 中 高 $- $ $- $ $- $ 非负中 非中 增高 $- $ $- $ 3 高 $- $ $- $ $- $ $- $ 非负中 非中 增高 $- $ $- $ 4 $- $ $- $ $- $ $- $ $- $ 负中 $- $ 降低 $- $ $- $ 5 高 $- $ $- $ 中或高 低 负低 低 $- $ 增高 $- $ 6 高 $- $ $- $ 中或高 低 正低 低 $- $ 增高 $- $ 7 $- $ $- $ 低或中 $- $ 低 负中 非过载 $- $ $- $ 增高 8 $- $ $- $ $- $ $- $ 中或高 负中 非过载 $- $ $- $ 增高 9 $- $ $- $ $- $ $- $ 高 负低 非过载 $- $ $- $ 增高 10 不低 $- $ $- $ 中或高 中或高 正低 零 $- $ 增高 降低 11 不低 $- $ $- $ 中或高 中或高 负中 零 $- $ 增高 降低 12 不低 $- $ $- $ 中或高 中或高 负中 低 $- $ 增高 降低 13 不低 $- $ $- $ 中或高 中或高 负低 低 $- $ 增高 降低 14 不低 高 高 $- $ 低 负低 低 $- $ 增高 降低 15 不低 高 高 $- $ 低 负低 零 $- $ 增高 降低 16 高 $- $ $- $ $- $ $- $ 零 零 $- $ 降低 $- $ 17 $- $ $- $ $- $ $- $ $- $ 负过载 $- $ 降低 降低 降低 18 $- $ $- $ $- $ $- $ $- $ 正过载 $- $ 降低 降低 降低 19 $- $ $- $ $- $ $- $ $- $ $- $ 过载 降低 降低 降低 20 $- $ $- $ $- $ $- $ $- $ 非负过载 $- $ 不变 不变 不变 21 $- $ $- $ $- $ $- $ $- $ 非正过载 $- $ 不变 不变 不变
下载: 导出CSV
表 3 初始条件和边界条件(%)
Table 3 Initial condition and boundary condition (%)
血液浓度 骨密度 软骨密度 结缔组织 骨皮质 100 100 0 0 断端间骨痂 0 0 0 100 骨痂外边缘 30 0 0 100
下载: 导出CSV
-
[1] Kayabasi O, Erzineanli F. Finite dement modeling and analysis of a new cemented hip prosthesis. Advances in Engineering Software, 2006, 37 (7):477-483 doi: 10.1016/j.advengsoft.2005.09.003 [2] Claes L E, Heigele C A. Magnitudes of local stress and strain along bony surfaces predict the course and type of fracture healing. Journal of Biomechanics, 1999, 32 (3):255-266 doi: 10.1016/S0021-9290(98)00153-5 [3] Ament C, Hofer E P. A fuzzy logic model of fracture healing. Journal of Biomechanics, 2000, 33 (8):961-968 doi: 10.1016/S0021-9290(00)00049-X [4] Lacroix D, Prendergast P J. A mechano-regulation model for tissue differentiation during fracture healing:analysis of gap size and loading. Journal of Biomechanics, 2002, 35 (9):1163-1171 doi: 10.1016/S0021-9290(02)00086-6 [5] Prendergast P J, Huiskes R, Soballe K. Biophysical stimuli on cells during tissue differentiation at implant interfaces. Journal of Biomechanics, 1997, 30 (6):539-548 doi: 10.1016/S0021-9290(96)00140-6 [6] Bailón-Plaza A, van Der Meulen M C H. A mathematical framework to study the effects of growth factor influences on fracture healing. Journal of Theoretical Biology, 2001, 212 (2):191-209 doi: 10.1006/jtbi.2001.2372 [7] Geris L, Gerisch A, Vander Sloten J, Weiner R, Van Oosterwyck H. Angiogenesis in bone fracture healing:a bioregulatory model. Journal of Theoretical Biology, 2008, 251 (1):137-158 doi: 10.1016/j.jtbi.2007.11.008 [8] García-Aznar J M, Kuiper J H, Gómez-Benito M J, Doblaré M, Richardson J B. Computational simulation of fracture healing:influence of interfragmentary movement on the callus growth. Journal of Biomechanics, 2007, 40 (7):1467-1476 doi: 10.1016/j.jbiomech.2006.06.013 [9] Kuiper J H, Ashton B A, Richardson J B. Computer simulation of fracture callus formation and stiffness restauration. In: Proceedings of the 12th Conference of the European Society of Biomechanics. Dublin, Ireland: Royal Academy of Medicine, 2000. 61-61 [10] Doblaré M, García J M, Gómez M J. Modelling bone tissue fracture and healing:a review. Engineering Fracture Mechanics, 2004, 71 (13-14):1809-1840 doi: 10.1016/j.engfracmech.2003.08.003 [11] Gómez-Benito M J, García-Aznar J M, Kuiper J H, Doblaré M. A 3D computational simulation of fracture callus formation:influence of the stiffness of the external fixator. Journal of Biomechanical Engineering, 2006, 128 (3):290-299 http://www.ncbi.nlm.nih.gov/pubmed/16706578 [12] Isaksson H, van Donkelaar C C, Huiskes R, Ito K. A mechano-regulatory bone-healing model incorporating cell-phenotype specific activity. Journal of Theoretical Biology, 2008, 252 (2):230-246 doi: 10.1016/j.jtbi.2008.01.030 [13] Simon U, Augat P, Utz M, Claes L. A numerical model of the fracture healing process that describes tissue development and revascularisation. Computer Methods in Biomechanics and Biomedical Engineering, 2011, 14 (1):79-93 doi: 10.1080/10255842.2010.499865 [14] Steiner M, Claes L, Ignatius A, Simon U, Wehner T. Disadvantages of interfragmentary shear on fracture healing-mechanical insights through numerical simulation. Journal of Orthopaedic Research, 2014, 32 (7):865-872 doi: 10.1002/jor.22617 [15] Steiner M, Claes L, Ignatius A, Niemeyer F, Simon U, Wehner T. Prediction of fracture healing under axial loading, shear loading and bending is possible using distortional and dilatational strains as determining mechanical stimuli. Journal of the Royal Society Interface, 2013, 10 (86):1123-1126 http://europepmc.org/abstract/med/23825112 [16] Carter D R, Hayes W C. The compressive behavior of bone as a two-phase porous structure. The Journal of Bone & Joint Surgery, 1977, 59 (7):954-962 doi: 10.1007/978-1-4471-5451-8_116 [17] Shefelbine S J, Augat P, Claes L, Simon U. Trabecular bone fracture healing simulation with finite element analysis and fuzzy logic. Journal of Biomechanics, 2005, 38 (12):2440-2450 doi: 10.1016/j.jbiomech.2004.10.019 [18] Claes L, Augat P, Suger G, Wilke H J. Influence of size and stability of the osteotomy gap on the success of fracture healing. Journal of Orthopaedic Research, 1997, 15 (4):577-584 doi: 10.1002/(ISSN)1554-527X [19] Duda G N, Eckert-Hübner K, Sokiranski R, Kreutner A, Miller R, Claes L. Analysis of interfragmentary movement as a function of musculoskeletal loading conditions in sheep. Journal of Biomechanics, 1998, 31 (3):201-210 https://www.sciencedirect.com/science/article/pii/S0021929097001279 [20] Kaspar D, Seidl W, Neidlinger-Wilke C, Ignatius A, Claes L. Dynamic cell stretching increases human osteoblast proliferation and cicp synthesis but decreases osteocalcin synthesis and alkaline phosphatase activity. Journal of Biomechanics, 2000, 33 (1):45-51 doi: 10.1016/S0021-9290(99)00171-2 [21] Guo Z Y, Yan Z Q, Bai L, Zhang M L, Jiang Z L. Flow shear stress affects macromolecular accumulation through modulation of internal elastic lamina fenestrae. Clinical Biomechanics, 2008, 23 (1):S104-S111 https://www.sciencedirect.com/journal/clinical-biomechanics/vol/23/suppl/S1 [22] 金大地, 史占军.骨与骨折愈合.成都:电子科技大学出版社, 1994. 15-16Jin Da-Di, Shi Zhan-Jun. Bone and Fracture Healing. Chengdu:University of Electronic Science and Technology Press, 1994. 15-16 [23] 李瑞宗.骨肿瘤诊断图谱.天津:天津科技翻译出版公司, 1993. 3-5Li Rui-Zong. Atlas of Bone Tumor Diagnosis. Tianjin:Tianjin Science and Technology Translation and Publishing Company, 1993. 3-5 [24] 金岩.组织工程学原理与技术.西安:第四军医大学出版社, 2004. 8-10Jin Yan. Principle and Technology of Tissue Engineering. Xi'an:The Fourth Military Medical University Press, 2004. 8-10 [25] 周树权.应力与微动对骨折愈合的影响.医药前沿, 2012, 2 (8):328-329 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=hainanyx201001051Zhou Shu-Quan. The effect of stress and micro motion on fracture healing. Frontiers of Medicine, 2012, 2 (8):328-329 http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=hainanyx201001051 [26] 王志鑫, 陈浩宏.骨折愈合学.武汉:湖北科学技术出版社, 1995. 16-19Wang Zhi-Xin, Chen Hao-Hong. Fracture Healing. Wuhan:Hubei Science and Technology Press, 1995. 16-19 [27] 郑阿奇, 丁有和. Visual C++教程.北京:机械工业出版社, 2015. 38-54Zheng A-Qi, Ding You-He. Visual C++ Course. Beijing:Machinery Industry Press, 2015. 38-54 [28] 侯增广, 赵新刚, 程龙, 王启宁, 王卫群.康复机器人与智能辅助系统的研究进展.自动化学报, 2016, 42 (12):1765-1779 http://www.aas.net.cn/CN/abstract/abstract18966.shtmlHou Zeng-Guang, Zhao Xin-Gang, Cheng Long, Wang Qi-Ning, Wang Wei-Qun. Recent advances in rehabilitation robots and intelligent assistance systems. Acta Automatica Sinica, 2016, 42 (12):1765-1779 http://www.aas.net.cn/CN/abstract/abstract18966.shtml [29] 宋亚飞, 王晓丹, 雷蕾.基于直觉模糊集的时域证据组合方法研究.自动化学报, 2016, 42 (9):1322-1338 http://www.aas.net.cn/CN/abstract/abstract18921.shtmlSong Ya-Fei, Wang Xiao-Dan, Lei Lei. Combination of temporal evidence sources based on intuitionistic fuzzy sets. Acta Automatica Sinica, 2016, 42 (9):1322-1338 http://www.aas.net.cn/CN/abstract/abstract18921.shtml -
图(11) / 表(3)
计量
- 文章访问数: 1820
- HTML全文浏览量: 265
- PDF下载量: 687
- 被引次数: 0
