-
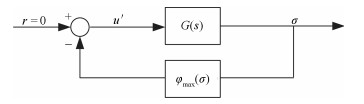
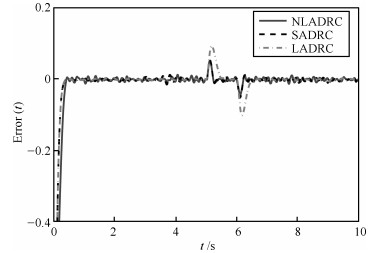
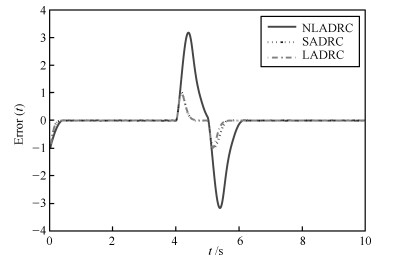
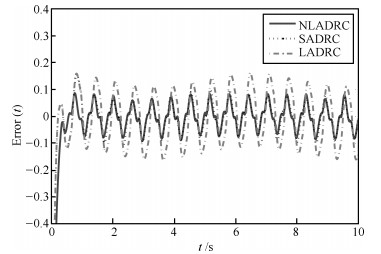
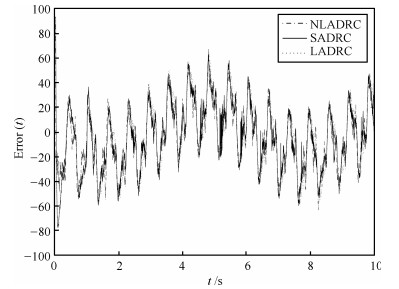
摘要: 非线性自抗扰控制 (Nonlinear active disturbance rejection control, NLADRC) 较线性自抗扰控制 (Linear active disturbance rejection control, LADRC) 具有跟踪精度高、抗干扰能力强等优点, 但在参数整定、稳定性分析以及控制性能分析等方面有一定的困难, 阻碍了非线性自抗扰控制在实际中的应用, 而线性自抗扰控制成为工程应用的首选.本文提出一种线性/非线性自抗扰控制切换控制方法, 该方法既综合了线性/非线性自抗扰控制的优点, 又解决了非线性自抗扰控制在参数整定、稳定性分析等方面的困难:首先, 分析线性/非线性自抗扰控制各自优缺点, 并给出了一种切换控制策略; 其次, 提出一种基于优化进行查表或利用拟合公式的参数整定方法; 再次, 提出基于劳斯判据和鲁棒波波夫判据的稳定性分析方法.通过仿真验证了该切换方法在跟踪精度、抗干扰能力等方面具有一定优势.该切换控制方法将有助于更好地发挥非线性机制在要求实现高精度、高抗扰能力场合的独特优势, 有望在工程实际中获得应用.Abstract: Nonlinear active disturbance rejection control (NLADRC) is superior to linear active disturbance rejection control (LADRC) in tracking precision, anti-disturbance ability, and so on. However, there are difficulties in parameter tuning, stability analysis, performance analysis, etc, which inhibits its application. Therefore, LADRC is more popular in engineering applications. This paper presents a linear/nonlinear active disturbance rejection switching control method, which has both of the advantages of LADRC and NLADRC, and solves the problems of parameter tuning, stability analysis, etc. Firstly, the characteristics of LADRC and NLADRC are presented, and then a switching strategy is proposed. Secondly, a simple practical parameter tuning method is provided. Thirdly, the stability is analyzed through Routh criterion and Popov criterion. Simulations show that the switching control method is superior to both LADRC and NLADRC in tracking precision and anti-disturbance. The proposed method fully demonstrates the advantages of nonlinear functions in occasions when high tracking precision and strong anti-disturbance ability are needed, and is hopefully to be adopted in practical application.
-
表 1 参数优化表
Table 1 Parameter optimization
h 噪声 M β01 β02 β03 0.001 0.0075~0.015 30 60 240 980 0.001 0.0025~0.0075 60 90 550 3 320 0.001 0.001~0.0025 120 150 1 460 15 280 0.005 0.0075~0.015 10 45 130 418 0.005 0.0025~0.0075 30 90 540 3 350 0.005 0.001~0.0025 60 120 1 000 8 000 0.01 0.0075~0.015 5 30 60 125 0.01 0.0025~0.0075 10 45 140 410 0.01 0.001~0.0025 20 60 250 980 -
[1] 韩京清.控制理论-模型论还是控制论.系统科学与数学, 1989, 9(4):328-335 http://www.cnki.com.cn/Article/CJFDTOTAL-STYS198904005.htmHan Jing-Qing. Control theory, is it a model analysis approach or a direct control approach? Journal of Systems Science and Mathematical Sciences, 1989, 9(4):328-335 http://www.cnki.com.cn/Article/CJFDTOTAL-STYS198904005.htm [2] 韩京清, 王伟.非线性跟踪一微分器.系统科学与数学, 1994, 14(2):177-183 http://www.cnki.com.cn/Article/CJFDTOTAL-STYS199402011.htmHan Jing-Qing, Wang Wei. Nonlinear tracking-differentiator. Journal of Systems Science and Mathematical Sciences, 1994, 14(2):177-183 http://www.cnki.com.cn/Article/CJFDTOTAL-STYS199402011.htm [3] 韩京清.非线性PID控制器.自动化学报, 1994, 20(4):487-490 http://www.aas.net.cn/CN/abstract/abstract14059.shtmlHan Jing-Qing. Nonlinear PID controller. Acta Automatica Sinica, 1994, 20(4):487-490 http://www.aas.net.cn/CN/abstract/abstract14059.shtml [4] 韩京清.一类不确定对象的扩张状态观测器.控制与决策, 1995, 10(1):85-88 http://www.cnki.com.cn/Article/CJFDTOTAL-KZYC501.019.htmHan Jing-Qing. The "extended state observer" of a class of uncertain systems. Control and Decision, 1995, 10(1):85-88 http://www.cnki.com.cn/Article/CJFDTOTAL-KZYC501.019.htm [5] 韩京清.自抗扰控制器及其应用.控制与决策, 1998, 13(1):19-23 http://cdmd.cnki.com.cn/Article/CDMD-10216-1014033694.htmHan Jing-Qing. Auto-disturbance-rejection controller and it's applications. Control and Decision, 1998, 13(1):19-23 http://cdmd.cnki.com.cn/Article/CDMD-10216-1014033694.htm [6] Gao Z Q. Scaling and bandwidth-parameterization based controller tuning. In:Proceedings of the 2003 American Control Conference. Denver, Colorado, USA:IEEE, 2003. 4989-4996 [7] 韩京清.自抗扰控制技术-估计补偿不确定因素的控制技术.北京:国防工业出版社, 2008. 183-287Han Jing-Qing. Active Disturbance Rejection Control Technique-the Technique for Estimating and Compensating the Uncertainties. Beijing:National Defense Industry Press, 2008. 183-287 [8] 陈星.自抗扰控制器参数整定方法及其在热工过程中的应用[硕士学位论文], 清华大学, 中国, 2008.Chen Xing. Active Disturbance Rejection Controller Tuning and Its Applications to Thermal Processes[Master dissertation], Tsinghua University, China, 2008. [9] 王海强, 黄海.扩张状态观测器的性能与应用.控制与决策, 2013, 28(7):1078-1082 http://www.cnki.com.cn/Article/CJFDTOTAL-KZYC201307025.htmWang Hai-Qiang, Huang Hai. Property and applications of extended state observer. Control and Decision, 2013, 28(7):1078-1082 http://www.cnki.com.cn/Article/CJFDTOTAL-KZYC201307025.htm [10] 齐晓慧, 李杰, 韩帅涛.基于BP神经网络的自适应自抗扰控制及仿真.兵工学报, 2013, 34(6):776-782 http://www.cnki.com.cn/Article/CJFDTOTAL-BIGO201306019.htmQi Xiao-Hui, Li Jie, Han Shuai-Tao. Adaptive active disturbance rejection control and its simulation based on BP neural network. Acta Armamentarii, 2013, 34(6):776-782 http://www.cnki.com.cn/Article/CJFDTOTAL-BIGO201306019.htm [11] 刘朝华, 张英杰, 章兢, 吴建辉.基于免疫双态微粒群的混沌系统自抗扰控制.物理学报, 2011, 60(1):791-799 http://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201101123.htmLiu Zhao-Hua, Zhang Ying-Jie, Zhang Jing, Wu Jian-Hui. Active disturbance rejection control of a chaotic system based on immune binary-state particle swarm optimization algorithm. Acta Physica Sinica, 2011, 60(1):791-799 http://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201101123.htm [12] 刘福才, 贾亚飞, 任丽娜.基于混沌粒子群优化算法的异结构混沌反同步自抗扰控制.物理学报, 2013, 62(12):120509 http://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201312016.htmLiu Fu-Cai, Jia Ya-Fei, Ren Li-Na. Anti-synchronizing different chaotic systems using active disturbance rejection controller based on the chaos particle swarm optimization algorithm. Acta Physica Sinica, 2013, 62(12):120509 http://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201312016.htm [13] 武雷, 保宏, 杜敬利, 王从思.一种自抗扰控制器参数的学习算法.自动化学报, 2014, 40(3):556-560 http://www.aas.net.cn/CN/abstract/abstract18321.shtmlWu Lei, Bao Hong, Du Jing-Li, Wang Cong-Si. A learning algorithm for parameters of automatic disturbances rejection controller. Acta Automatica Sinica, 2014, 40(3):556-560 http://www.aas.net.cn/CN/abstract/abstract18321.shtml [14] 李述清, 张胜修, 刘毅男, 周帅伟.根据系统时间尺度整定自抗扰控制器参数.控制理论与应用, 2012, 29(1):125-129 http://www.cnki.com.cn/Article/CJFDTOTAL-KZLY201201021.htmLi Shu-Qing, Zhang Sheng-Xiu, Liu Yi-Nan, Zhou Shuai-Wei. Parameter-tuning in active disturbance rejection controller using time scale. Control Theory & Application, 2012, 29(1):125-129 http://www.cnki.com.cn/Article/CJFDTOTAL-KZLY201201021.htm [15] Wu D, Chen K. Frequency-domain analysis of nonlinear active disturbance rejection control via the describing function method. IEEE Transactions on Industrial Electronics, 2013, 60(9):3906-3914 doi: 10.1109/TIE.2012.2203777 [16] Wu D, Chen K. Limit cycle analysis of active disturbance rejection control system with two nonlinearities. ISA transactions, 2014, 53(4):947-954 doi: 10.1016/j.isatra.2014.03.001 [17] Guo B Z, Zhao Z L. On convergence of nonlinear active disturbance rejection for SISO systems. In:Proceeding of the 24th Chinese Control and Decision Conference. Taiyuan, China:IEEE, 2012. 3507-3512 [18] Guo B Z, Zhao Z L. On convergence of the nonlinear active disturbance rejection control for MIMO systems. SIAM Journal on Control and Optimization, 2013, 51(2):1727-1757 doi: 10.1137/110856824 [19] Dahleh M, Tesi A, Vicino A. On the robust Popov criterion for interval Lur'e system. IEEE transactions on automatic control, 1993, 38(9):1400-1405 doi: 10.1109/9.237655 [20] Gao Z Q, Hu S H, Jiang F. A novel motion control design approach based on active disturbance rejection. In:Proceedings of the 40th IEEE Conference on Decision and Control. Orlando, FL:IEEE, 2001. 4877-4882 -




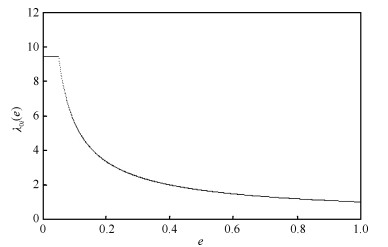
 下载:
下载: